Research progress on the application and development of the material point method in dynamic simulation of geological hazards
-
摘要:
在解决崩塌、滑坡、泥石流等大变形地质灾害问题时，常采用数值模拟的方法。如何准确高效地模拟这类问题一直以来都是个难题。物质点法（Material Point Method，MPM）作为一种新兴的数值方法，克服了传统有限元和有限差分等数值方法在模拟大变形时产生的网格畸变问题，并已成功应用于地质灾害中的大变形分析。为了解物质点法在地质灾害大变形模拟中的研究进展，本文在现有研究的基础上简要介绍了物质点法的基本原理，主要总结了物质点法在模拟滑坡、泥石流、地裂缝等地质灾害大变形问题中的应用以及最新的研究进展。同时，指出了物质点法在现有研究中存在的精度、计算效率、多物理场耦合等问题，并展望了物质点法在工程地质中进一步发展的趋势。
Abstract:Numerical simulation is commonly used to address large deformation geological disasters such as collapses, landslides, and debris flows. Accurately and efficiently simulating these issues has always been a challenge. The Material Point Method (MPM), as emerging numerical method, overcomes the grid distortion problems of traditional numerical methods such as the finite element method (FEM) and finite difference method (FDM) when simulating large deformations, and has been successfully applied in the large deformation analysis of geological disasters. In order to understand the research progress of MPM in the large deformation simulation of geological disasters, this paper briefly introduces the basic principles of MPM based on current research. It also summarizes the application of MPM in simulating large deformations of geological disasters such as landslide, debris flow, and ground fracture, highlighting the latest research progress. Furthermore, it identifies issues in existing MPM research, such as accuracy, computational efficiency, and coupling of multi-physics fields, and discusses future trends in MPM development withinengineering geology.
-
0. 引 言
水库蓄水后，岩土体长期受库水冲刷、浸泡、浪蚀、侵蚀及水位周期性涨落等因素耦合作用，岩土体的强度降低，结构弱化，水文和工程地质条件发生改变，诱发岸坡发生滑移、坍塌等岸线后退现象，进而形成与新环境条件相适应的库岸形态，即库岸再造[1 − 2]。水库塌岸是一个涉及工程地质学、岩土工程学和环境地质学等多个学科的复杂问题。它可能对水库的正常运行和周边的地质环境带来负面影响，会造成库区土地资源减少，水土流失严重，周边居民被迫搬迁，会增加河道淤积，诱发古滑坡复活，诱发涌浪等严重危害。它已经成为影响库区经济发展及库周人民财产安全的重大地质灾害问题[3]，也是威胁库区两岸人民稳定生活的紧急社会难题。
当前，我国大型水利，水库数量和总库容跃居世界之首，水库蓄水运行期间，塌岸是不可避免的重大地质灾害。尤其是西南高山峡谷地区的河流水库，地形地貌的多样性和地质条件的复杂性，加之地质构造的活跃性以及库水的强烈动力作用，共同促成了塌岸现象的多样性，并且塌岸点分布广泛，影响面积大。澜沧江流域主要是峡谷河道型水库，在上游河段规划布置了八个梯级的水电站，如，里底、托巴、乌弄龙等以发电为主的大型水电站。经调查，该流域水库蓄水后，随着库水位的上升，岸坡局部可能产生不同程度调整变形，新增部分滑坡、库岸变形、塌岸等影响区，造成基础设施沉陷、塌方，部分房屋开裂等，严重影响当地居民的生产生活。从上世纪60年代起，国内外学者[4 − 6]已经开始重点关注水库塌岸问题了。在塌岸的影响因素，形成机理、破坏机制、塌岸模式、塌岸过程、预测方法等方面均开展了全面深入地研究[7]。
塌岸机理的研究通常是对塌岸现场的地质条件和影响因素的深入调查[8]，包括岸坡的组成与结构、形态特征、水库水位的波动[9]、波浪作用、水库的长期浸泡以及冻融循环等多种因素。塌岸形态及特征取决于水文因素（流速、击岸浪的波高、水库动态）、地质地貌因素（被冲刷岩层的产状、岩土体的物理力学性质、被冲刷岩土体的均匀性、岸坡形态），这两大因素决定了塌岸的类别和范围。王小东，等[10]从河流侵蚀的角度出发研究岸坡物质流失与河道地形变化的关系，提出了一些模型和方法用于计算侵蚀量以及预测达到平衡状态时的岸坡形态。塌岸现象最常见的诱因是河床的侵蚀加深以及河岸的冲刷，而这两者的发生都依赖于水流条件。水流的强度越大，塌岸发生的可能性及其严重程度也会大幅增加。因此，塌岸的发生主要受水流条件的制约，它在塌岸的产生和发展中扮演着关键角色，是主要的驱动因素。
目前，用于预测水库塌岸的方法[11]主要包括动力法、图解法、工程类比法、模拟试验法和水动力预测法等。动力法通过分析塌岸量、波能和岩土体抗冲刷能力之间的关联，构建预测模型。这种方法具有明确的物理基础，但要确立一个准确的“关系方程”，需要依赖一定数量的实测数据作为支撑。王跃敏，等[12]在过去的10年中，通过对数十个水库塌岸的深入调查和研究，提出了“两段法”，以指导外福铁路线（连接外洋和福州）的塌岸预测和设计工作。尽管“两段法”被用于预测塌岸宽度，但在应用过程中，还需要通过卡丘金法进行对照分析，以全面评估其合理性，并做出科学的选择。即“两段法”的适用性仍需通过广泛的实际应用来进一步验证和确认[4, 13]。汤高明，等[14]在对现有塌岸预测方法进行系统分析的基础上，结合三峡库区岸坡结构的特点，提出了三种塌岸预测方法：岸坡结构法[15]、三段法和多元回归分析法。数值模拟手段[16 − 18]作为行之有效的方法常用于研究塌岸的稳定性，预测塌岸的宽度和高程，本文在传统有限元分析方法的基础上研发了适用于土质塌岸的有限元分析方法[19 − 20]。
调查发现茨中移民安置点前缘在2019年汛期开始持续坍塌，2020年塌岸强度加剧，前缘不时有土体剥落坍塌入水。本文以茨中前缘塌岸为例，选取典型塌岸断面作为地质原型，分析安置点前缘塌岸模式及成因机制，研究岸坡塌岸过程及塌岸机理。分别采用卡丘金法、岸坡结构法、本文提出的有限元方法预测茨中前缘塌岸宽度和高程，为茨中前缘塌岸防治提供决策依据，对当地移民安置点的建设和搬迁安全以及促进库区两岸区域经济可持续发展具有重要意义。同时，本文的研究成果也可为其他类似地区的水库塌岸研究和防治提供参考，具有一定的普适性。
1. 工程概况
1.1 工程背景
乌弄龙水电站位于滇西纵谷山原区，地貌特征是北高南低，北部属青藏高原，南部属云贵高原，平面上呈现出三江并流的地貌景观。库区为高山峡谷地形，河谷形态多呈不对称“V”字型，局部呈“U”型，江水面与山顶一般高差500 m～
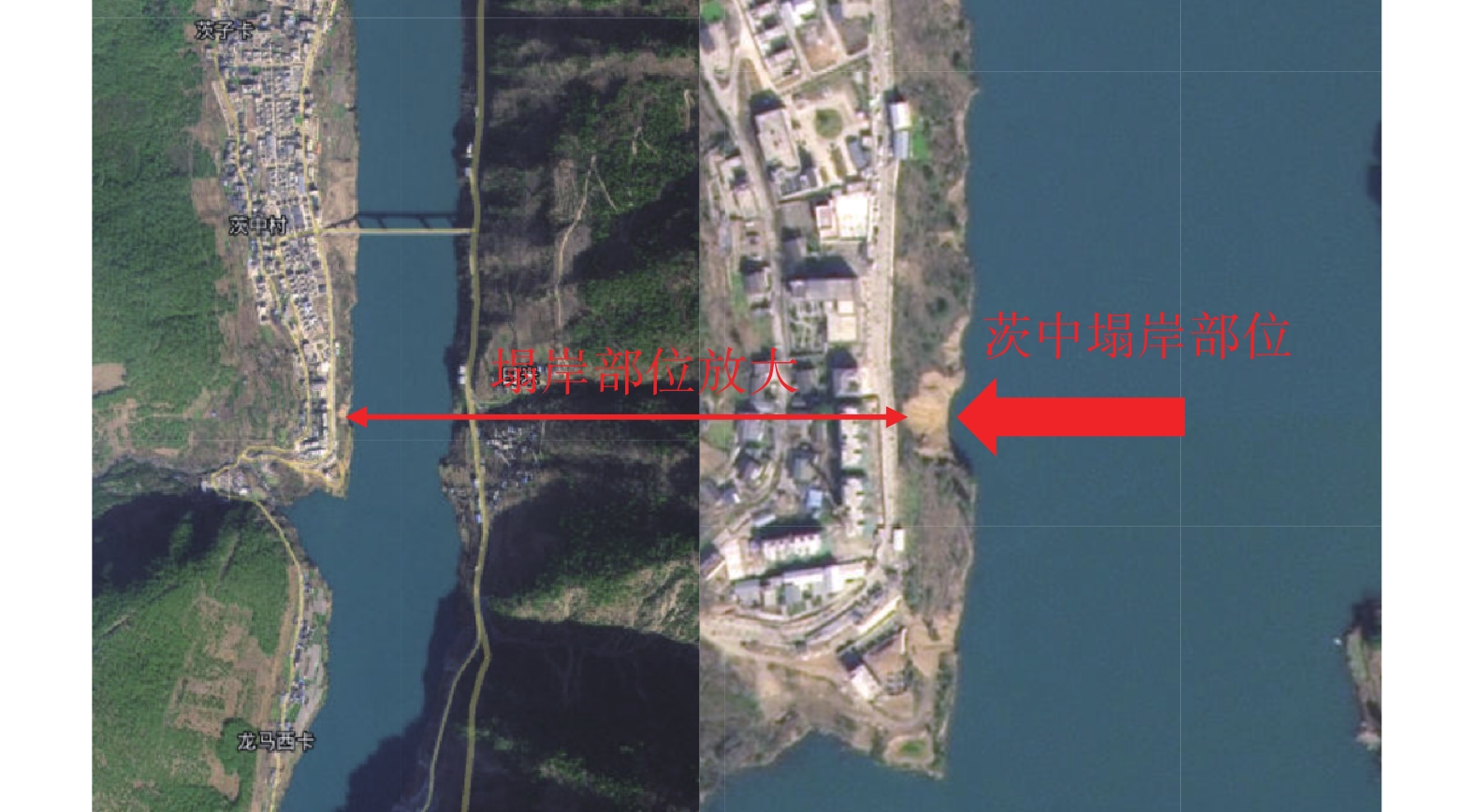
1260 m。澜沧江水流急湍，深切河谷，两岸山体雄厚，山势陡峻，一般自然岸坡坡度45°～65°，局部为悬崖峭壁。库段河谷两岸见有零星的Ⅰ、Ⅱ、Ⅲ级阶地分布。塌岸所在的茨中台地属于澜沧江右岸的Ⅲ级冲洪积阶地（图1），阶地的发育程度较好，分布高程在1940 m～2000 m。阶地后缘依靠雄浑的山体，山麓多堆积有崩坡积块碎石土。阶地前缘天然坡度较陡，坡度30°～38°，蓄水前，阶地前缘高出河水位（1848 m左右）约80 m～85 m左右。台地前缘江边局部有残留的Ⅰ～Ⅱ级阶地，蓄水后多淹没于水下。塌岸的茨中村属于德钦县燕门乡，距离乌弄龙水电站约10.5 km。塌岸区域于汛期开始逐渐向岸里产生持续剥落式坍塌，塌岸形状呈顶部窄，下部宽的不规则梯形。2023年年初开始，塌岸重新复活，5月开始塌岸持续加剧，至6月塌岸已经延伸至安置点12 m大道的路中间，人行道和行车道的半幅路基已经坍塌，路面悬空，塌岸造成该段公路已无法通行。同时塌岸顶部的边缘距离安置点新建的6层公租楼水平距离仅余8 m左右，边坡塌岸发展和建筑物相互影响可能会进一步加剧坍塌安全风险，2023年7月初，开始进行塌岸部位的水下回填工作，如图2所示。
![]()
图 2 茨中村安置点前缘塌岸现象Figure 2. Bank Collapse Phenomenon at the Front Edge of Cizhong Village Resettlement Site1.2 现场调研
通过对澜沧江流域塌岸模式调查，发现该地区塌岸模式可分为侵蚀-剥蚀型、坍塌型、滑移型、崩塌型和流土型等类型[11]。该地区由于河流冲刷、库水位升降、风浪作用诱发了堆积体和岸坡出现多样的塌岸模式，典型破坏模式如图3所示。
![]()
图 3 调查澜沧江库岸的主要塌岸模式Figure 3. Investigate of the Main Collapse Modes along the Lancang River Reservoir Banks2. 茨中前缘塌岸机制
2.1 成因分析
经勘查分析，蓄水初期影响塌岸的因素主要为库岸地质条件、风浪作用。茨中安置点前缘塌岸的下游受无加陇巴河沟口洪积物向左岸推移的影响，河床略被束窄，偏向左岸，形成凹岸，塌岸部位易受主流顶冲淘刷，如图4所示。
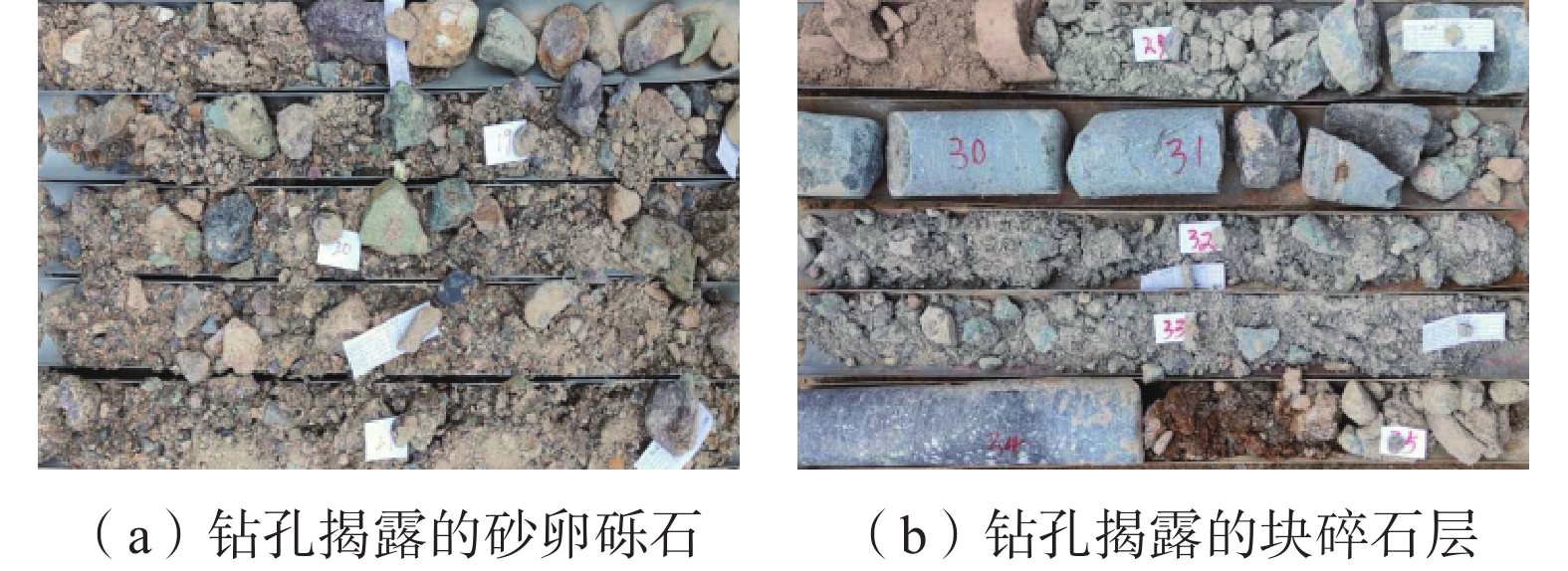
通过现场踏勘发现，塌岸区域前缘较陡，坡度可达40°，无基岩裸露，属于高陡的土质岸坡。分析钻孔勘察结果，如图5（a-b）所示，塌岸部位覆盖层厚度大，粒径粗细不一，分选性差，呈多元结构，厚度超50 m，从上到下各层的结构可归纳为杂填土层、含漂石砂砾石层、块碎石层以及基岩等。蓄水后库水动水作用淘刷水位附近土体，细粒物质被冲刷带走，加之水的软化作用，颗粒之间的连接弱化，导致下部被掏空呈浪蚀龛，上部土体失去支撑产生掉落坍塌。
![]()
图 5 塌岸部位钻孔地层结构Figure 5. Stratigraphic Structure of Drilled Cores at the Collapse Site2022年茨中安置点开工建设德钦县保障性租赁住房，包括3栋剪力墙结构的楼房，楼高6层。该住房作为外荷载施加在岸坡上，且距离塌岸部位比较近，会对外侧高陡岸坡稳定及土压力平衡产生不良影响。2023年以来，由于附近施工，当地施工重型渣土车满载通行。前缘塌岸后公路外缘土体较为单薄，难以抵抗上部附加荷载。综合分析认为蓄水后岩土体软化、岸坡地形、地层结构、顶流冲刷以及房屋荷载是诱发该岸坡发生塌岸的主要因素。
2.2 演化过程
通过对成因机制和主要影响因素分析认为，茨中前缘为典型的坍塌后退型塌岸，其演化过程为：①该段库岸为冲洪积深厚覆盖层构成的土质高陡岸坡，受右侧无加陇巴河冲沟沟口洪积物的影响，该段为凹岸，较上下游易受水流冲刷掏蚀。但在长期的地质作用下，已形成暂稳定坡体。②蓄水后波浪冲击岸坡后，顺底部产生回流，对水下浅滩进行冲刷，同时水下土体饱和软化，物理力学性质降低，在水流冲刷作用下，产生夷平作用，岸坡逐渐趋缓。③在正常蓄水位线附近，风浪冲刷岸坡壁，形成浪蚀龛，上部岩土体失去支撑坍塌、崩落，并逐层向岸里剥离，向后退缩。④水流波荡将塌落物击碎，并搬运至深水处。随着时间推移在新的平衡条件下库岸变形逐渐停止。⑤当塌岸部位存在暴雨入渗、动力荷载等外界因素有较大变化时，内部的应力、应变条件发生改变，诱发岸坡岩土体再次产生坍塌，直至变形达到新的平衡。
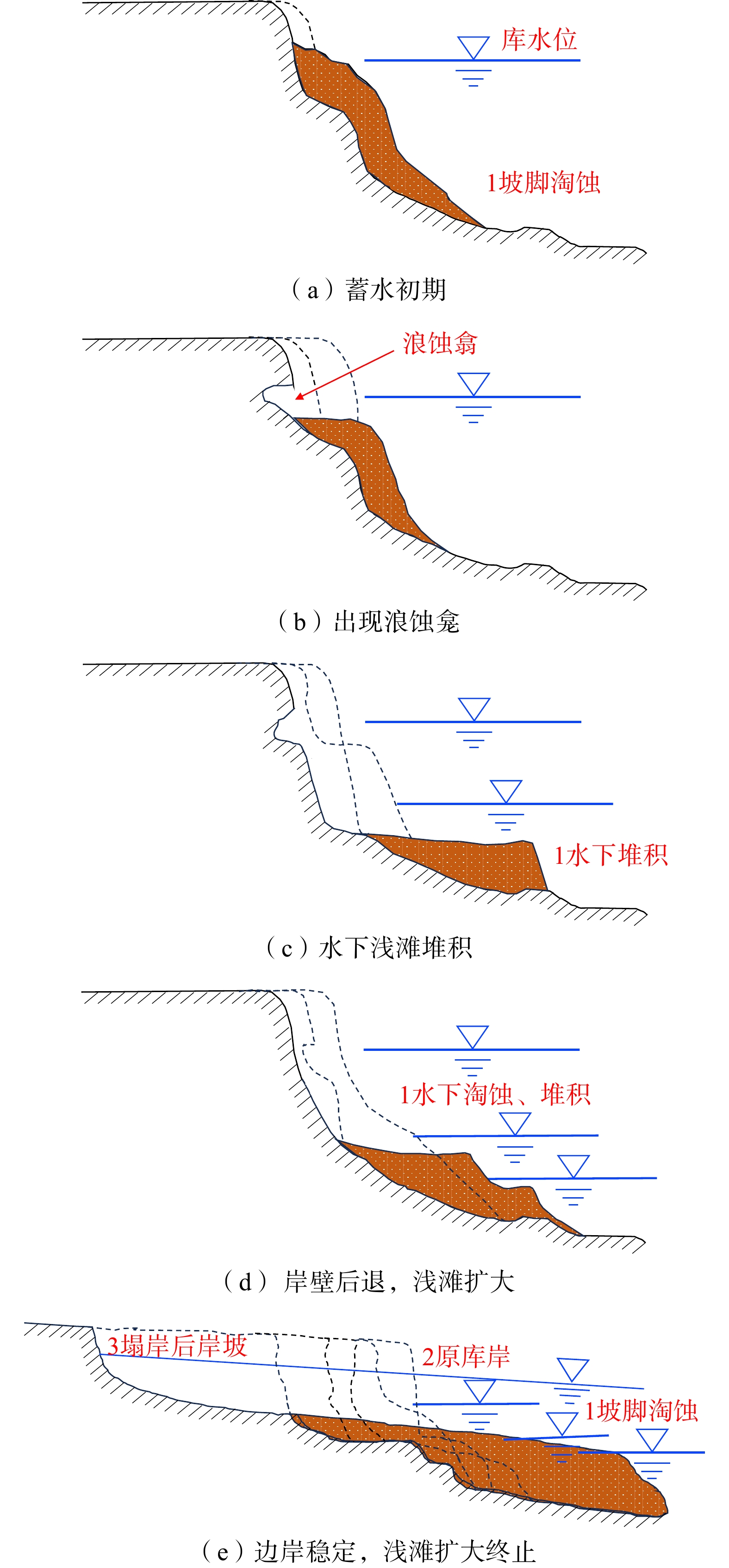
蓄水初期，在水流的持续冲刷淘蚀作用下，水下岸坡会逐渐形成较为平缓的侵蚀堆积区域，即浅滩（图6a）。继而，坍塌后的岸壁会呈现出较为陡峭的坡度，下部通常超过35°，而顶部在短期内甚至可能达到70°−80°的陡峭程度，形成浪蚀龛（图6b）。随着风浪的持续侵蚀淘刷，岸边的破坏会不断加剧，导致岸线逐步后退，上缘陡壁坍塌剥落，水下的浅滩区域也会随之堆积并扩大（图6c）。该过程会反复循环，壁岸坍塌入水，直至水上方的岸壁和水下的浅滩区域达到一种相对稳定的状态（图6d）。在最后阶段，水流和波浪的侵蚀作用对岸壁的破坏已经基本结束，水库岸边开始趋于稳定（图6e）。坍塌后退型塌岸的特点是后退的速度较快、范围较广。
![]()
图 6 坍塌后退型塌岸演化过程Figure 6. Evolution Process of Collapse-and-Retreat Type Bank Collapse3. 模型构建及参数选取
3.1 模型构建
为确定塌岸土体的物理力学性质，西北勘测设计研究院对水库区塌岸部位的岩土体进行了调研，发现塌岸部位横剖面水下地形较原始地形变缓，总体约25°，平衡点位于
1880 m高程附近，塌岸部位横剖面的计算模型如图7所示。![]()
图 7 茨中安置点前缘塌岸计算模型图Figure 7. Calculation Model Diagram of the Bank Collapse in Front edge of the Cizhong Resettlement Site3.2 地层参数选取
由于该地区地质条件复杂，不同位置和深度的岩土体性质存在较大差异，需要通过广泛的试验来全面了解塌岸部位岩土体的特性，准确选取参数。因此，在多组钻孔内进行了多场景重型动力触探试验（N63.5）来获取地层参数数据，这一过程也确保了有限元模型输入参数的准确性和可靠性，相比传统方法仅依赖有限的经验参数或简单试验数据，更能反映实际地层的复杂特性。
3.2.1 地层参数选取过程
1. 开展勘察工作并收集成果资料
西北院在乌弄龙水电站勘察期间，于水库区开展了大量工作，积累丰富勘察成果。同时，贵州地矿基础工程公司在保障性租赁住房项目场地进行诸多勘探，也获取了相应成果。这些成果为地层参数数据的选取奠定了坚实基础，提供了多方面的数据支持。
2. 进行重型动力触探试验
为评价塌岸土体密实度等物理力学性质，在2个钻孔内开展多组重型动力触探试验（N63.5），因所夹大块石较多，其中23组可用于统计。依据试验成果，动探击数变异处于10～40 击之间，经换算得出地基土承载力约120 kPa～
1000 kPa，变形模量为4 MPa～60 MPa。此试验数据是了解地层土体力学特性的重要依据。3.2.2 地层参数选取方法
1. 计算均值和标准差
利用数理统计中的标准差法剔除异常数据并保留有效数据，这些数据构成了用于分析的数据集。针对每个参数，分别计算其均值（$ \mu $）和标准差（$ \sigma $）。对于每一个参数，其数据为$ {x_1},{x_2},\cdots{x_n} $，则通过计算式（1～2）得到每个参数各自的均值和标准差，从而确定数据的集中趋势和离散程度。
均值
$$ \mu =\frac{1}{n}\sum\limits_{i = 1}^n {{x_i}} $$ (1) 标准差
$$ \sigma = \sqrt {\frac{1}{n}\sum\limits_{i = 1}^n {（{x_i} - } \mu {）^2}} $$ (2) 2. 判断并剔除异常数据
对于每个参数数据集中的每个数据点$ {x_i} $，将其与该参数确定的合理波动范围进行比较。如果$ {x_i} < \mu - 3\sigma $或$ {x_i} > \mu + 3\sigma $，则认为该数据点是异常数据，将其从数据集中剔除。
3. 保留有效数据
经过上述剔除异常数据的操作后，每个参数数据集中剩余的数据即为在合理波动范围内的有效数据。这些有效数据能够更准确地反映岩土体物理力学性质等参数的真实情况。经过上述过程和计算步骤，得出塌岸部位岩土体物理力学参数见表1，塌岸影响区周边土体的稳定坡角参数见表2。
表 1 塌岸区岩土体物理力学参数Table 1. The Physical and Mechanical Parameters of the Rock-Soil Mass in the Bank Collapse Area地层名称 天然密度
（g/cm3）允许承载力
（kPa）压缩模量
Es（0.1～0.2）内摩擦角
tanφ粘聚力
c（kPa）①人工填土 1.8 120～180 5～10 0.35～0.4 5～10 ②砂卵砾石 2.0 250～350 30～50 0.45～0.6 10～45 ③块碎石土 2.1 350～450 40～60 0.6～0.7 0～20 ④二叠系基岩 2.7 800～ 1000 0.7～.08 300～500 表 2 塌岸预测稳定坡角建议值Table 2. Recommended Value for Predicting Stable Slope Angle of Bank Collapse地层名称 水下稳定
坡角α（°）水上稳定
坡角β（°）水位变幅区
稳定坡角γ（°）冲磨蚀角
$ \theta $（°）①松散覆盖层 27 40 18～30 18 ②砂卵、块碎石土 42 48 19～32 40 4. 结果与分析
4.1 茨中前缘塌岸方法和预测分析
本文塌岸区域位于茨中阶地下游侧，总体属于高陡的土质岸坡，无基岩出露，可采用卡丘金法进行塌岸范围预测。其失稳模式是坍塌后退型塌岸，对于该类型岸坡的塌岸预测，通用二段法和岸坡结构法均有较好的适用性。
4.1.1 卡丘金法
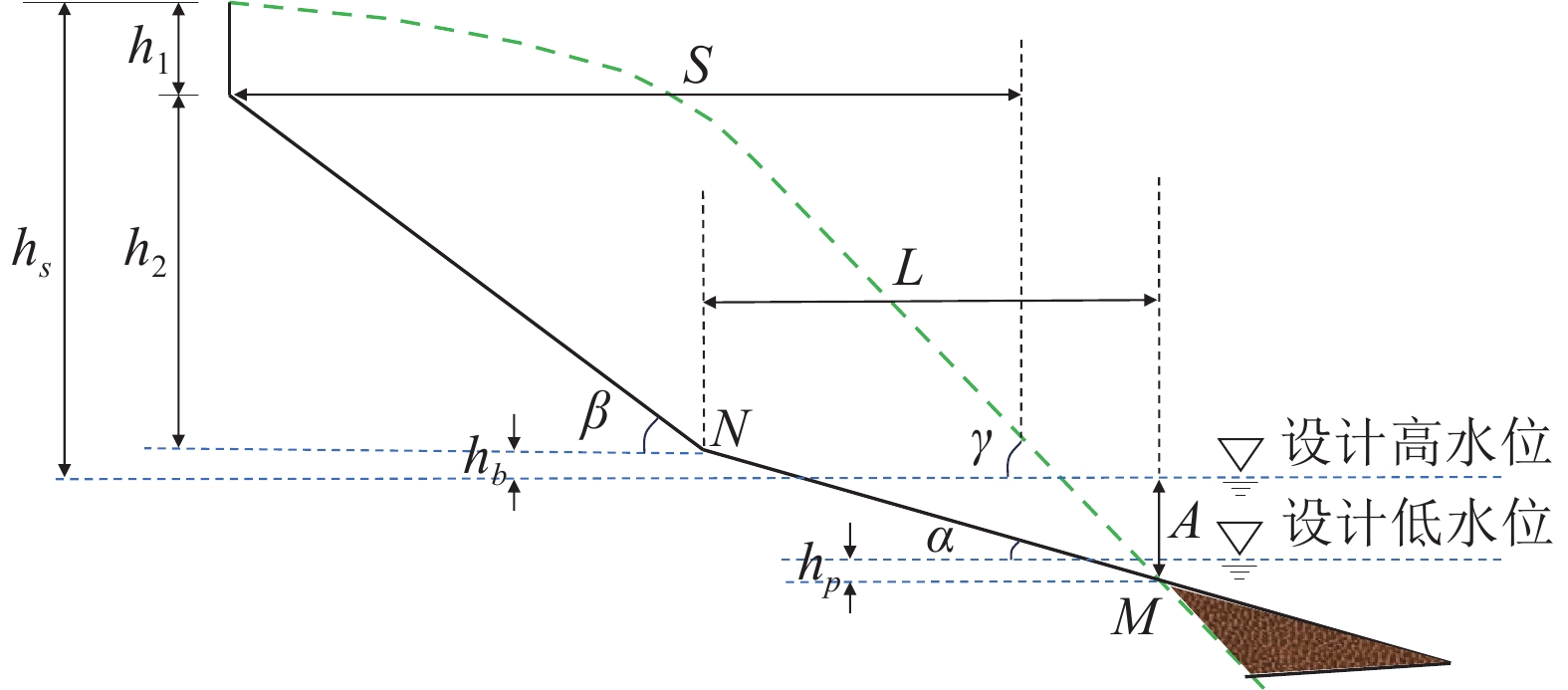
卡丘金法的起算点是设计低水位减去波浪冲蚀深度，分界点是波浪的最大冲蚀高度，将水库岸坡分为水上、水下两部分，基于此分界点选择合适的水上和水下岸坡稳定坡角来预测塌岸的宽度和高程[15, 21]，图8为卡丘金塌岸预测示意图。卡丘金预测水库塌岸宽度如式（3）：
$$ \begin{split} S =& N\left[ {\left( {A + {h_p} + {h_b}} \right)\cot \alpha + {h_2}\cot \beta - (A + {h_p})\cot \gamma } \right]\\ &{h_2} = {h_s} - {h_b} - {h_1} \end{split} $$ (3) 式中：S——塌岸最终宽度；
N——比例系数；
A——库水位变化幅度；
hp——波浪掏蚀深度；
hb——库水波浪爬高；
hs——设计高水位以上岸坡高度；
h1——垂直陡坎高度；
h2——浪爬高度以上岸坡高度；
$ \alpha $——水下冲磨蚀坡角；
$ \beta $——水上稳定坡角；
$ \gamma $——初始坡岸坡角。
经详细地质勘察，茨中安置点前缘岸坡中，松散覆盖层和砂卵、块碎石土的水下稳定坡角分别为27°和45°，水上稳定坡角分别为40°和48°，由于原始岸坡较陡，库水水深比较大，塌岸后在水下坡脚处不易形成堆积阶地，因此，比例系数N取值为1.2。浪爬高度的计算如式（4）：
$$ {h_b} = 3.2kh\tan \alpha $$ (4) 式中，k为被冲蚀的岸坡表面糙度系数，一般砂质岸坡k=0.55～0.75；砾石质岸坡k=0.85～0.9；混凝土k=1；抛石k=0.775。h为波浪高度，根据澜沧江库区实际情况，建议取0.7 m。因此，茨中安置点库区的浪爬高度为：
$$ {h_b} = 3.2 \times 0.8 \times 0.7 \times \tan {18{\text{°}}} = 0.582\;{\mathrm{m}} $$ 基于茨中安置点前缘的地质条件、地层岩性及岸坡受波浪侵蚀情况，将上述参数代入式（3）可求得卡丘金预测的塌岸宽度为62.935 m。采用图解法判断最终塌岸的可能范围如图9所示。
![]()
图 9 卡丘金预测茨中安置点前缘塌岸结果Figure 9. Kachugin Prediction Results for the Bank Collapse at the Front Edge of the Cizhong Resettlement Site$$ \begin{split} S =& 1.2[ ( {7 + 0.582 \times 2 + 0.582} )\cot {{18}{\text{°}}} + 25.585\cot {{40}{\text{°}}} -\\ &(7+0.582 \times 2)\cot {{36}{\text{°}}} ] = 62.935\;{\text{m}} \end{split} $$ 从图9分析结果可以得出，覆盖层水上稳定坡角平均为40°左右，块碎石土的水下稳定坡角约为15°～28°。图中红线为根据统计稳定坡角进行的塌岸部位稳定分析结果，由此可知，岸坡可能的不稳定区域位于1#保障性租赁住房后部。塌岸部位的覆盖层综合内摩擦角约为36°，以其作为覆盖层的天然休止角进行分析，蓝线标识为岸坡可能的不稳定区域，刚好越过1#保障性租赁住房，到达后缘垂直陡坎部位。
4.1.2 岸坡结构法
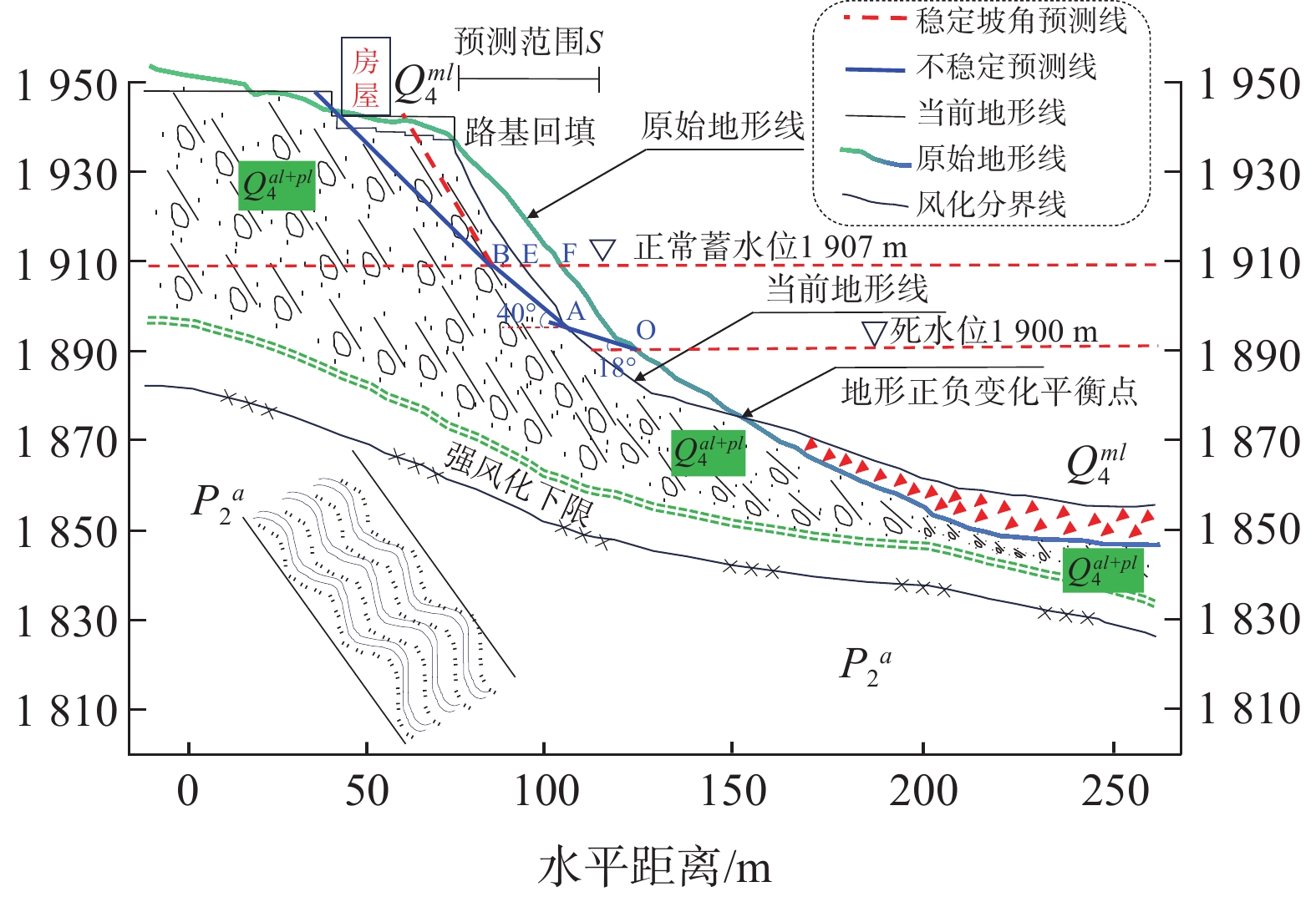
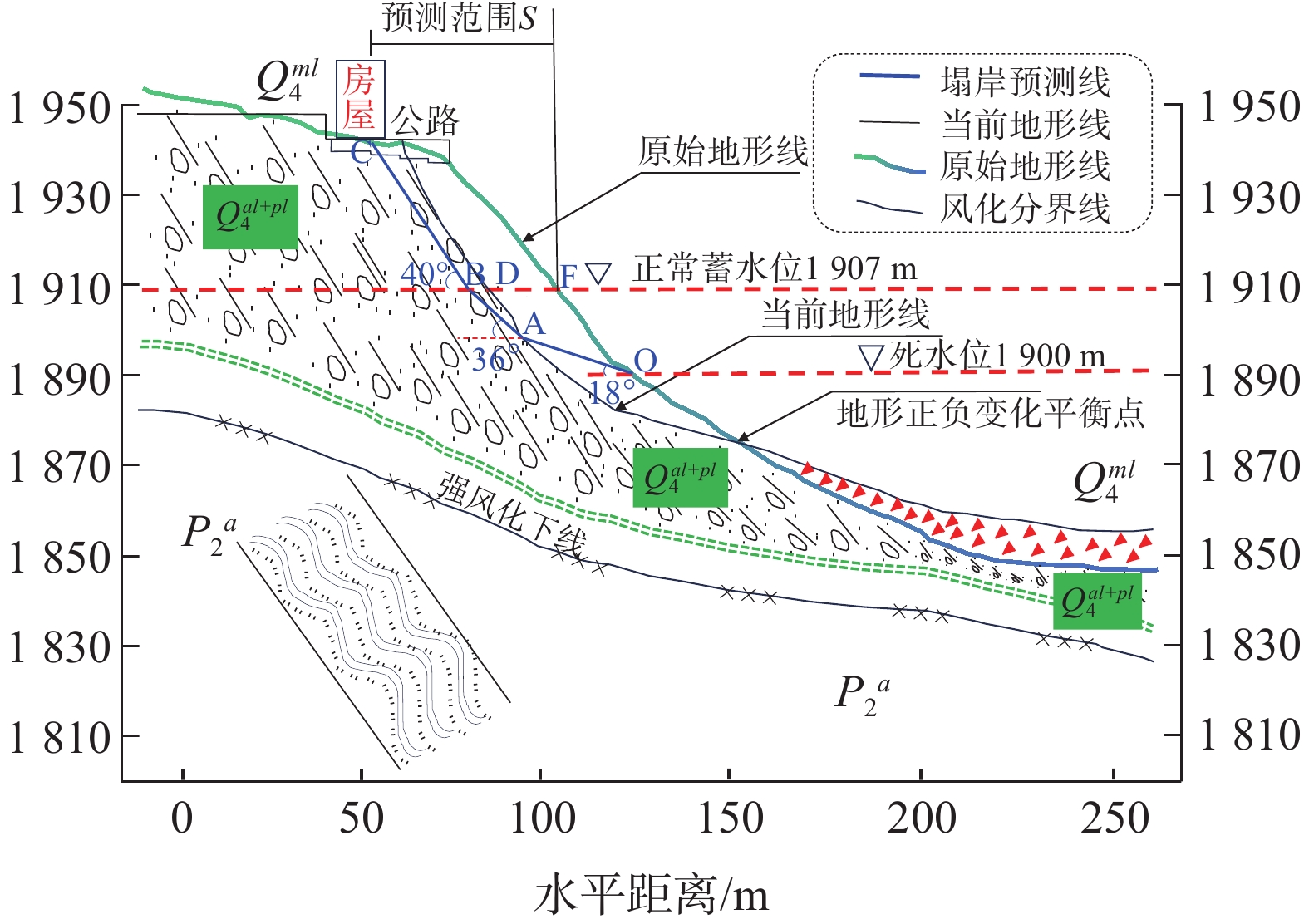
基于3.2参数取值，采用岸坡结构法预测茨中安置点前缘塌岸的宽度，需考虑死水位、正常蓄水位，预测参数则考虑冲磨蚀角、水上稳定坡角这两个参数。以死水位作为塌岸起算点，茨中安置点前缘塌岸宽度的具体预测步骤如下：
（1） 绘制茨中安置点所在岸坡计算的地层岩性和地质剖面，如图10所示。
![]()
图 10 岸坡结构法预测茨中安置点前缘塌岸结果Figure 10. Bank Slope Structure Method Prediction Results for Bank Collapse at the Front Edge of Cizhong Resettlement Site（2） 标出水库正常蓄水危险（正常蓄水水位高程
1907.0 m）和死水位线（设计低水位1900 m）（3） 以死水位线与坡面地形线相交点O为起算点，以松散覆盖层土体冲磨蚀角$ {\theta _1} $=18°为倾角作正常蓄水位下部塌岸的预测线，与基覆界限交于A点。
（4） 以A为起点，以砂卵、块碎石土冲磨蚀角$ {\theta _2} $=36°为倾角，继续绘制塌岸预测线，与水库正常蓄水位线交于B点。由图10可以发现，AD线较基覆界线要陡，说明该范围内强风化岩体偏于安全，不会发生塌岸，因此AB之间基覆界线即为该段的预测塌岸线。
（5） 以B点为起点，以松散覆盖层水上稳定坡角γ=27°为倾角作塌岸线与地形线交于C点，那么BC则是正常蓄水位以上的预测塌岸线。
（6） CF两点之间的水平距离S即为最终预测塌岸水平影响宽度，依据地质剖面图高程可知，C点高程即为预测剖面的塌岸影响高程。
乌弄龙水电站蓄水至
1907.00 m高程后，高程1900.00 ～1907.00 m之间的覆盖层土体在库水升降及波浪作用下将失稳垮塌，发生前缘塌岸。以岸坡的典型剖面为例，计算其最终塌岸高程为1943.0 m，塌岸宽度约为52.26 m。茨中安置点公租房和公路大多分布在1930.00 ～1950.00 m高程之间，预测水库正常蓄水后，该库岸范围内将发生塌岸再造危及公租房建设安全，破坏道路行车安全。4.1.3 本文有限元方法
选取澜沧江德钦-维西段乌弄龙水电站库区共20多处岸坡塌岸为研究对象，基于课题组前期研究基础，提出一套适用于不同控制因素诱发的塌岸范围预测方法。
1. 影响水库塌岸范围的主控因素分析
通过文献调研和前期研究，将影响塌滑范围分为以下三个部分考虑：
水力条件：塌滑是由库水位的频繁上升和下降所致，因此将库水位的影响分两方面体现：一是库水位差变化对坡脚掏蚀及稳定性的影响，其表征指标采用库水位与塌滑体剪出口之间的高差$ \Delta h $表示；二是库水位频繁变化引起坡体内地下水位变化，主要是水压力的变化对岸坡稳定性的影响，表征指标采用孔隙水压力系数$r_u $表示。
坡体物质组成：分为坡体物质成分（粒度成分）、胶结程度、密实情况等，实际上反映的是坡体物理力学性质，其表征指标可采用强度参数，即粘聚力无量纲强度比（c/γH）、内摩擦角$ \varphi $、非均匀岩土层之间的强度比值$ {c_2}/{c_1} = \tan {\varphi _2}/\tan {\varphi _1} $。
坡体几何形态：松散堆积体稳定的主要因素是地形陡缓和高低。其表征指标为坡角$ \beta $和自定义的坡高比D（定义为坡高H与总的坡高（或地基层深度H1））之间的关系比，如图11所示。
本研究在分析影响水库塌岸范围的主控因素时，针对水力条件、坡体物质组成和坡体几何形态三个方面进行了数值试验方案设计，如表3所示。在水力条件方面，考虑库水位与滑塌体剪出高差（Δh），分别设置了5、10、20、30、60、90 m等不同水平，以体现其从低到高的变化对塌岸的影响。孔隙水压力（$ r_u $）设置为0.1、0.2、0.3、0.4等不同数值，用于研究其从小到大变化时的作用。坡体物质组成方面，黏聚力c/γH取值范围为0.06至0.5，内摩擦角φ涵盖5°至35°，层间强度比值$ {c_2}/{c_1} = \tan {\varphi _2}/\tan {\varphi _1} $从0.25至3.50，通过这些不同取值来分析坡体物质特性对塌岸的影响。坡体几何形态上，地形坡角β从5°至90°，坡高比D从1.0至4.0，以此探究地形因素在塌岸过程中的作用。通过这些不同参数组合的数值试验，为后续建立塌岸稳定性预测方程提供数据基础。
表 3 数值试验方案设计Table 3. Numerical Test Scheme Design基本条件 影响因素 方案分析 方案说明 水力条件 库水位与滑塌体剪出高差$ \Delta h$/m 5、10、20、30、60、90 低→高 孔隙水压力ru 0.1、0.2、0.3、0.4 小→大 坡体物质组成 黏聚力c/γH 0.06、0.10、0.12、0.15、0.18、0.18、0.20、0.25、0.3、0.35、0.4、0.45、0.5 低→高 内摩擦角$ \varphi $/（°） 5°、10°、15°、20°、25°、30°、35° 弱→强 层间强度比值$ {c_2}/{c_1} = \tan {\varphi _2}/\tan {\varphi _1} $ 0.25、0.50、0.80、1.00、1.10、1.20、1.40、1.50、
1.60、1.80、2.00、2.50、3.00、3.50上硬下软↓
上软下硬坡体几何形态 地形坡角β/（°） 5°、15°、30°、40°、48°、50°、52°、53°、55°、60°、75°、90° 平缓→直立 坡高比D 1.0、1.2、1.3、1.4、1.5、1.8、2.0、2.2、2.5、3.0、4.0 单层→多层 2. 基于弧形滑面搜索的塌岸稳定性预测方程
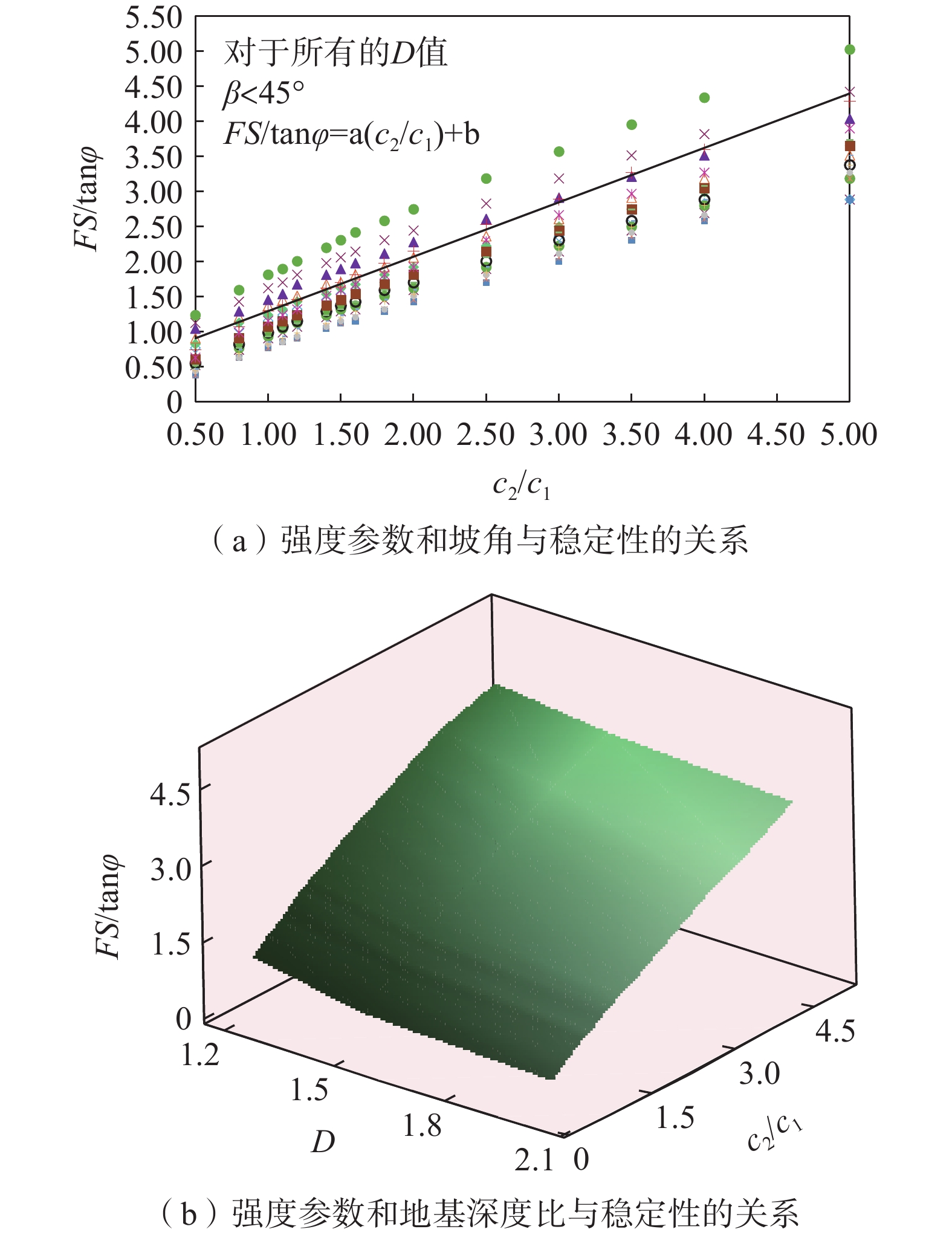
结合乌弄龙水电站库区不同成因松散堆积体（共20处）塌岸范围，选择具有代表性的塌岸剖面，依据表3中的计算方案，进行大量的敏感性数值计算分析，得出边坡稳定性与不同影响因素之间的关系曲线，如图12所示。$ FS/\tan \varphi $作为因变量与三个影响因素之间的拟合回归方程，如式（5）所示，回归方程的相关系数R2=95.91%，拟合精度相当高，说明强度特性和几何形态的变化对平缓边坡稳定性的影响较大，三者均为较敏感影响因素。
![]()
图 12 平缓岸坡的三种影响因素之间的相互关系Figure 12. Interrelationships Among Three Influencing Factors of Gentle Slopes回归方程：
$$ \begin{split} &\frac{{FS}}{{\tan \varphi }} = 0.646({c_2}/{c_1}) - 0.032\beta - 0.769D + 2.47 \\ &{R^2} = 95.91\% \end{split} $$ (5) 公式（5）体现了有限元法能够更准确地预测塌岸范围，而传统方法难以实现如此精确的量化分析和预测。从图12和回归方程可得，对乌弄龙水电站库区的松散堆积体或土质边坡的稳定性与不同土层的强度比值成正相关，与地形坡角$ \beta$和自定义的坡高比D呈负相关。而塌岸范围则与强度参数c、φ及地形坡角$ \beta$呈负相关，与蓄水位高程及潜在滑面地下水浸润面面积呈正相关关系。
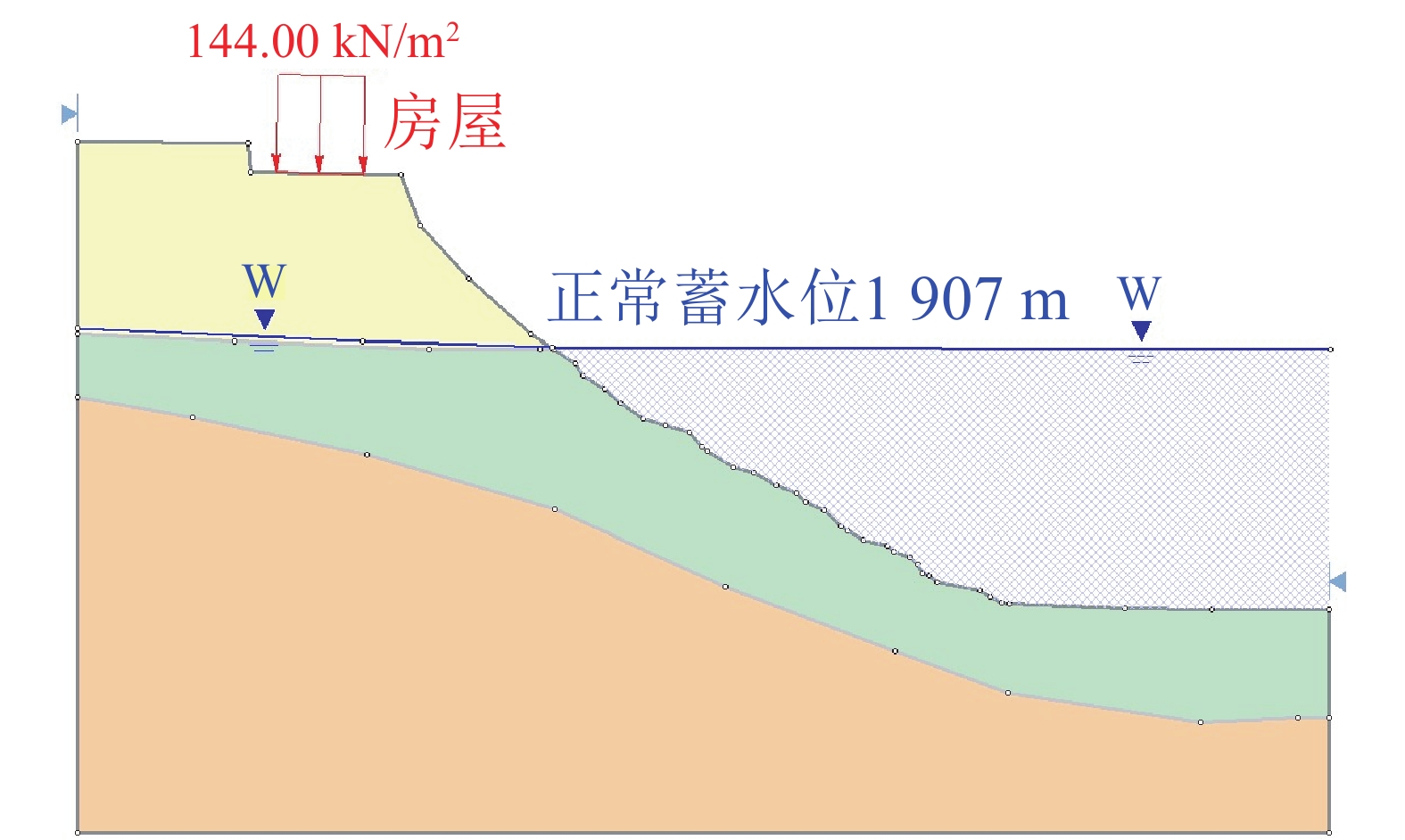
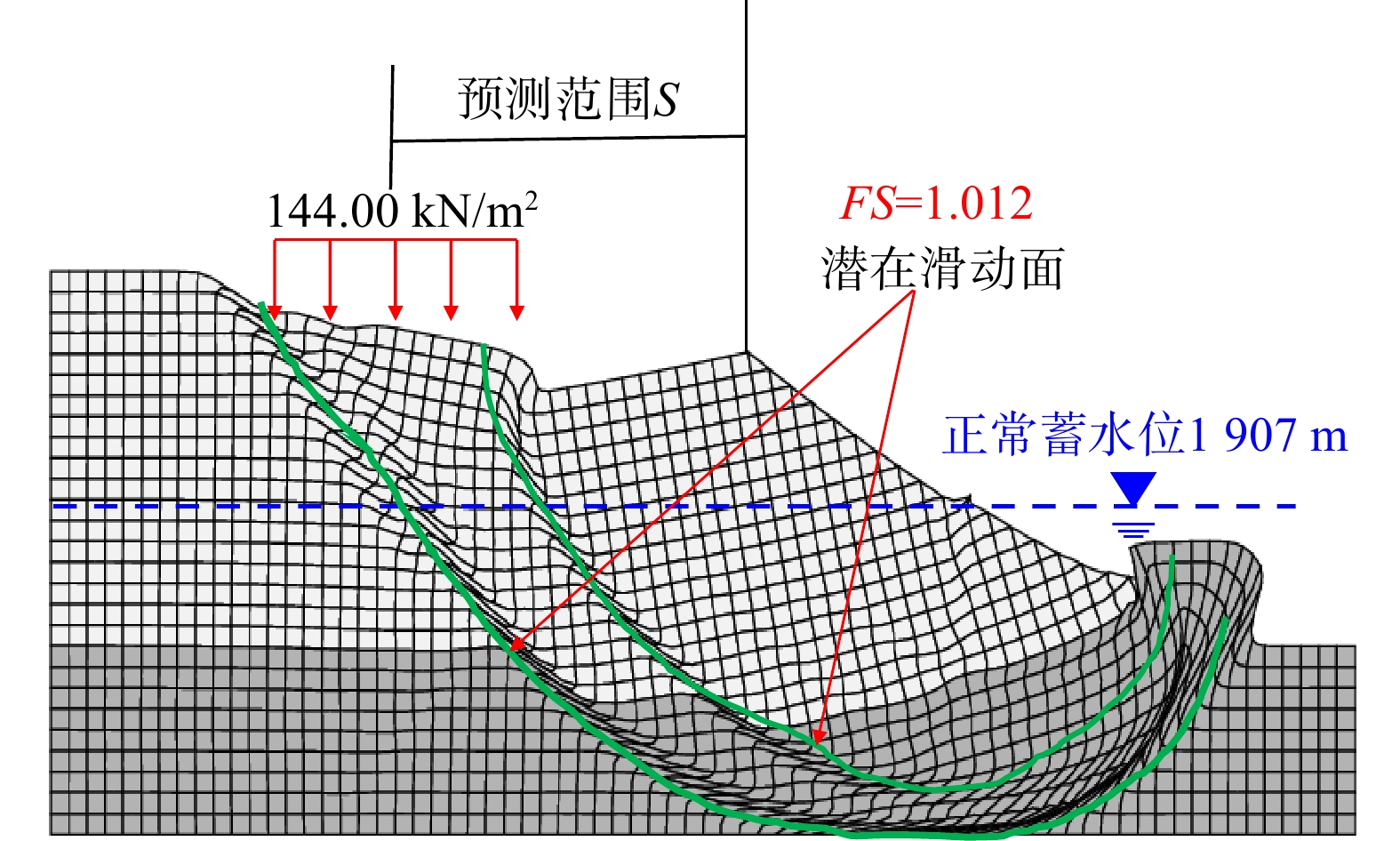
选取茨中安置点前缘塌岸剖面，如图13，塌岸部位的覆盖层综合内摩擦角约为36°<45°，塌岸的稳定性及失稳范围可参考式（5），基于表1与表2中的参数进行计算，得到塌岸潜在滑动面位置及滑动面形态，如图14所示。
![]()
图 13 茨中安置点前缘塌岸剖面图Figure 13. Cross-sectional diagram of the bank collapse at the leading Edge of the Cizhong Settlement site![]()
图 14 茨中安置点前缘塌岸潜在滑动面Figure 14. Potential sliding surface of the bank collapse at the front edge of the Cizhong settlement site由图13 − 14可知，塌岸区域综合考虑了岸坡自重、上部建筑物基础底面、库水位波动等主要荷载，在岸坡中同时出现两个临界滑动面，其失稳模式为岸坡浅层失稳和深层失稳。滑面较深的滑弧与基底相切形成深层失稳模式，较浅的滑弧并未与基底相切，深度较浅，最终在边坡的右岸出露处相汇合。浅层滑动面的安全系数FS为1.012，根据稳定状态划分标准，基本处于稳定性差～基本稳定状态。浅层滑动面位于公租房前的大道附近，深层临界滑动面在坡顶的位置主要集中在1#保障性租赁住房中后部，预估最终塌岸高程为
1945.0 m，塌岸宽度约为51.528 m。计算结果与现场坍塌的情况比较吻合，塌滑主要集中在前缘内部路大道上。该岸坡若不经系统性地永久治理，大幅增强岸坡的抗冲刷和自稳能力，可以判断随着时间推移，岸坡进一步变形松弛，或当环境条件改变时，岸坡整体失稳的风险会更大，尤其是在暴雨、地震等非正常工况下，潜在滑动面发展为更深的滑动位置，可能导致1#保障性租赁住房倾覆性倒塌破坏。
4.2 茨中前缘塌岸影响效应分析
4.2.1 预测结果对比分析
工程区属滇西纵谷山原区地貌单元，位于三江地槽褶皱系I级构造单元，查明了库岸坡度、坡面形态、沟谷切割情况，以及库岸物质组成、岸坡结构、特殊地层的分布，采用了三种不同的方法预测塌岸的范围，计算结果如表3所示。
表 4 茨中安置点前缘塌岸预测结果Table 4. Predicted results of the bank collapse at the front edge of the Cizhong resettlement site预测方法 预测塌岸宽度m 预测塌岸高程m 卡丘金法 62.935 1948.0 岸坡结构法 52.26 1943.0 本文有限元方法 51.528 1945.0 由表3可知，三种预测方法中，卡丘金法预测的宽度和高程均比较大，预测结果相对保守，偏于安全，相比另外两种方法，存在预测误差大、精确度低、变异性高等缺点。本文提出的有限元方法和岸坡结构法预测塌岸宽度和高程较为相近、预测结果离散型小、保真性高，说明这两种方法的预测结果具有一致性，可靠性强。但相对于岸坡结构法，本文提出的有限元方法可适用于多因素、多场景下的岩土体，考虑了自变量地下水位和因变量坡体物理参数对阶地土质岸坡前缘崩塌的动态影响，更加切合实际。岸坡结构法和本文有限元方法塌岸预测的位置也较为接近，位于1#保障性租赁住房的中偏后部，与现场勘查结果一致。
4.2.2 预测结果差异机制
1. 计算模型与影响因素
卡丘金法基于经验公式，在计算过程中，依赖于特定的稳定坡角等参数，这些参数的选择具有一定的经验性。在茨中安置点前缘岸坡的计算中，根据地层岩性及岸坡受波浪侵蚀情况确定水下稳定坡角（如松散覆盖层为27°，砂卵、块碎石土为45°）、水上稳定坡角（如松散覆盖层为40°，砂卵、块碎石土为48°）以及比例系数N（取值为1.2）等参数，进而计算塌岸宽度。其计算模型相对简单，主要依赖于经验参数和对坡角的假设，对复杂地质条件和多种因素相互作用的考虑不够全面。在计算过程中，对于房屋荷载、岩土体在蓄水过程中的动态变化以及多因素相互作用等情况的考虑相对有限。
有限元方法基于对影响水库塌岸范围的主控因素进行详细分析，包括水力条件（如库水位与塌滑体剪出口之间的高差$ \Delta h $、孔隙水压力系数$ r_u $）、坡体物质组成（如强度参数，即粘聚力无量纲强度比c/γH、内摩擦角$ \varphi $、非均匀岩土层之间的强度比值$ {c_2}/{c_1} = \tan {\varphi _2}/\tan {\varphi _1} $）、坡体几何形态（如坡角和自定义的坡高比 D）等。通过大量的敏感性数值计算分析，得出边坡稳定性与这些影响因素之间的关系曲线，进而建立回归方程来预测塌岸范围。在茨中安置点前缘塌岸的计算中，考虑了岸坡自重、上部建筑物荷载（如保障性租赁住房）、动水压力等主要荷载，以及两个临界滑动面的情况，通过回归方程计算塌岸潜在滑动面位置及滑动面形态，从而得到塌岸宽度和高程。
2. 适用条件与局限性
卡丘金法适用于一些地形、地质条件相对简单，且主要受波浪冲蚀和地形控制的塌岸情况。在较为复杂的地质条件和多因素影响下，其预测精度可能受到限制。在茨中前缘塌岸这种受蓄水岩土体软化、房屋荷载等多种因素共同作用的情况下，卡丘金法的局限性就较为明显，导致其预测结果相对保守，塌岸宽度和高程的预测值偏大。
本文有限元方法能够考虑多因素、多场景下的岩土体情况，对复杂的地质和工程条件具有更好的适应性。可以模拟不同工况下岸坡的变形和失稳过程，更准确地预测塌岸范围。在茨中前缘塌岸的预测中，有限元方法能够较好地反映实际情况，预测结果与现场坍塌情况较为吻合，塌岸宽度和高程的预测值相对更接近实际值。
4.2.3 塌岸影响及对周边环境影响效应
受乌弄龙水电站库区蓄水影响，2019年茨中台地前缘开始逐渐坍塌，2020年初塌岸部位顶部扩展至安置点12 m大道的路中间，5月份实际监测到的最大塌岸宽度达到了50～53 m，本文有限元方法预测的51.528 m与实际监测结果较为接近，误差小于±5%，符合允许范围。现场监测的位移总体呈缓慢增加的趋势，但每日的变形量总体较小，公租房表部的观测点数据每日呈震荡变化趋势。
在塌岸形态上，有限元方法预测的潜在滑动面位置与实际通过地质勘查和监测确定的滑动面位置基本一致，尤其是对浅层和深层滑动面的形态和位置预测较为准确。在时间发展趋势方面，有限元方法模拟的塌岸发展过程与实际监测数据在不同阶段的变化趋势较为吻合，能够较好地反映塌岸在不同水位变化下的动态响应。
通过卡丘金法、岸坡结构法和有限元方法对茨中前缘塌岸的预测分析，得到了不同的结果（如塌岸宽度、高程等），这些结果反映了各方法从不同角度对塌岸问题的理解。卡丘金法和岸坡结构法从自然地质条件和水力作用方面揭示了岸坡掏蚀在塌岸过程中的重要性，而有限元方法则进一步展示了在自然因素基础上，人为因素（如建筑物荷载）与自然因素相互作用对塌岸的影响。综合来看，茨中前缘塌岸是多种因素复杂作用的结果，各预测方法相互补充，共同为全面理解塌岸机理和准确预测塌岸范围提供了依据。在实际工程中，需要综合考虑各种因素，制定合理的防护和治理措施。
水库蓄水后，塌岸区域再次产生强烈坍塌、悬空。塌岸的范围向上、下游侧扩展，对上下游临近岸坡的稳定性造成一定的影响。根据岸坡稳定分析，如果任由岸坡的变形破坏继续发展，岸坡潜在的不稳定区域将扩展至安置房中部～后部，若不及时进行岸坡前缘的防护和治理，塌岸范围的扩大可能对保障性租赁住房稳定产生极不利影响和整体倾覆风险，甚至造成茨中村二次搬迁和移民安置的危害。
5. 结论与展望
本文以乌弄龙水电站区域的茨中移民安置点前缘塌岸为研究对象，通过现场钻孔勘查、地质调查、成因分析、预测方法等手段，分析了塌岸区域的地层岩性并确定了塌岸预测的关键参数，研究了塌岸破坏模式和塌岸机制，提出了本文有限元方法并预测了塌岸宽度和高程，最后分析了塌岸对周围环境的影响效应。本研究的主要结论如下：
（1） 茨中安置点前缘塌岸属高陡的土质岸坡，蓄水后在风浪侵蚀及库水位升降作用下，坡脚被软化并淘刷形成凹槽或空腔，岸坡上部土体发生错落、坍塌，形成坍塌后退型塌岸破坏模式。
（2） 蓄水岩土体软化、岸坡地形、地层结构、顶流冲刷以及房屋荷载是诱发该岸坡发生塌岸的主要因素。
（3） 采用卡丘金法、岸坡结构法和本文提出的有限元方法预测塌岸的宽度和高程，卡丘金方法预测塌岸范围偏大，偏于安全，岸坡结构法和有限元法预测范围比较接近，塌岸位置位于1#保障性租赁住房的中偏后部，与现场勘查结果一致，结果可靠性较强，预测塌岸宽度约为52 m，高程约为
1945 m。（4） 塌岸对周边环境影响较大，会向上、下游侧扩展，影响临近岸坡的稳定性，严重影响当地交通通行，若不及时防护和治理，可能对保障性租赁住房稳定产生不利影响和整体倾覆风险。
（5） 本文有限元法在水库塌岸预测中具有多因素耦合分析和动态模拟的能力，在复杂地质和工程条件下预测精度相对于传统方法更高。未来将考虑长期流变特性和极端环境对塌岸的影响，与遥感监测技术相结合，可以实时获取大范围库岸的地形、地貌变化信息，为有限元模型提供更及时、准确的边界条件和初始数据。
-
![]()
图 2 物质点法求解示意图（① 物质点信息映射 ②动量方程求解 ③更新物质点信息）
Figure 2. Schematic diagram of material point method solution (①Mapping of material point information, ②Solving the momentum equation, ③Updating material point information)
![]()
图 3 固结程度和时间因子关系图（修改自Abe，2014）
Figure 3. Relationship diagram between degree of consolidation and time factor (Modified from Abe, 2014)
![]()
图 4 不同摩擦系数下钢球质点位置随时间变化（修改自Lei，2022）
Figure 4. Variation of steel ball particle position over time under different friction coefficients (modified from Lei, 2022)
表 1 模拟滑面与实际滑面对比信息表（王升，2022）
Table 1 Comparison table of simulated slip surface and observed slip surface（Wang Sheng，2022）
滑面对比 剪出口水平距
/m（后缘）剪出口高差
/m（后缘）滑面最大
深度/m滑带角度
/（°）模拟滑面 544.5 111.9 53.9 5.3 实际滑面 457.0 92.1 49.7 2.2 
下载: 导出CSV
-
[1] 陈建平,辛亚波,王泽鹏,等. 样本选取对地质灾害易发性评价的影响——以山西柳林县为例[J]. 中国地质灾害与防治学报,2024,35(3):152 − 162. [CHEN Jianping,XIN Yabo,WANG Zepeng,et al. Effect of sample selection on the susceptibility assessment of geological hazards:A case study in Liulin County,Shanxi Province[J]. The Chinese Journal of Geological Hazard and Control,2024,35(3):152 − 162. (in Chinese with English abstract)] CHEN Jianping, XIN Yabo, WANG Zepeng, et al. Effect of sample selection on the susceptibility assessment of geological hazards: A case study in Liulin County, Shanxi Province［J］. The Chinese Journal of Geological Hazard and Control, 2024, 35（3）: 152 − 162. （in Chinese with English abstract）
[2] 孙玉进. 岩土大变形问题的物质点法研究[D]. 北京:清华大学,2017. [SUN Yujin. Study on large deformation of rock and soil by material point method[D]. Beijing:Tsinghua University,2017. (in Chinese with English abstract)] SUN Yujin. Study on large deformation of rock and soil by material point method［D］. Beijing: Tsinghua University, 2017. （in Chinese with English abstract）
[3] 张雄,廉艳平,刘岩,等. 物质点法[M]. 北京:清华大学出版社,2013. [ZHANG Xiong,LIAN Yanping,LIU Yan,et al. Material point method[M]. Beijing:Tsinghua University Press,2013. (in Chinese)] ZHANG Xiong, LIAN Yanping, LIU Yan, et al. Material point method［M］. Beijing: Tsinghua University Press, 2013. （in Chinese）
[4] 廉艳平,张帆,刘岩,等. 物质点法的理论和应用[J]. 力学进展,2013,43(2):237 − 264. [LIAN Yanping,ZHANG Fan,LIU Yan,et al. Material point method and its applications[J]. Advances in Mechanics,2013,43(2):237 − 264. (in Chinese with English abstract)] LIAN Yanping, ZHANG Fan, LIU Yan, et al. Material point method and its applications［J］. Advances in Mechanics, 2013, 43（2）: 237 − 264. （in Chinese with English abstract）
[5] Yerro Colom A,Alonso Pérez de Agreda E,Pinyol Puigmartí N M. The Material Point Method:A promising computational tool in geotechnics[C]//Challenges and Innovations in Geotechnics. 2013:853 − 856
[6] SULSKY D,ZHOU S J,SCHREYER H L. Application of a particle-in-cell method to solid mechanics[J]. Computer Physics Communications,1995,87(1/2):236 − 252.
[7] ZIENKIEWICZ O C,SHIOMI T. Dynamic behaviour of saturated porous media; The generalized Biot formulation and its numerical solution[J]. International Journal for Numerical and Analytical Methods in Geomechanics,1984,8(1):71 − 96.
[8] VERRUIJT A. An Introduction to Soil Dynamics[M]. DordrechtSpringer Netherlands,2010
[9] Beuth L,Benz T,Vermeer P A,et al. Large deformation analysis using a quasi-static material point method[J]. Journal of Theoretical and Applied Mechanics,2008,38(1 − 2):45 − 60
[10] BEUTH L,WIĘCKOWSKI Z,VERMEER P A. Solution of quasi-static large-strain problems by the material point method[J]. International Journal for Numerical and Analytical Methods in Geomechanics,2011,35(13):1451 − 1465.
[11] 黄鹏,张雄. 岩土边坡失效分析的物质点法研究:中国计算力学大会2010(CCCM2010) 暨第八届南方计算力学学术会议(SCCM8),中国四川绵阳,2010[C][143]. [HUANG Peng,Zhang Xiong. Material Point Method for Failure Analysis of Rock and Soil Slopes:Chinese Congress of Computational Mechanics 2010 (CCCM2010) and the 8th Southern Conference of Computational Mechanics (SCCM8),Mianyang,Sichuan,China,2010[143] (in Chinese with English abstract)] HUANG Peng, Zhang Xiong. Material Point Method for Failure Analysis of Rock and Soil Slopes: Chinese Congress of Computational Mechanics 2010 （CCCM2010） and the 8th Southern Conference of Computational Mechanics （SCCM8）, Mianyang, Sichuan, China, 2010[143] （in Chinese with English abstract）
[12] HUANG P,LI S L,GUO H,et al. Large deformation failure analysis of the soil slope based on the material point method[J]. Computational Geosciences,2015,19(4):951 − 963.
[13] LLANO-SERNA M A,FARIAS M M,PEDROSO D M. An assessment of the material point method for modelling large scale Run-out processes in landslides[J]. Landslides,2016,13(5):1057 − 1066.
[14] LI X P,WU Y,HE S M,et al. Application of the material point method to simulate the post-failure runout processes of the Wangjiayan landslide[J]. Engineering Geology,2016,212:1 − 9.
[15] 宰德志,庞锐. 基于物质点法的土体强度对边坡失稳滑动距离影响研究[J]. 水利与建筑工程学报,2021,19(5):46 − 51. [ZAI Dezhi,Pang Rui. Influence of soil strength on sliding distance of slope instability based on material point method [J]. Journal of Water Resources and Architectural Engineering,21,19(5):46 − 51. (in Chinese with English abstract)] ZAI Dezhi, Pang Rui. Influence of soil strength on sliding distance of slope instability based on material point method ［J］. Journal of Water Resources and Architectural Engineering, 21, 19（5）: 46 − 51. （in Chinese with English abstract）
[16] 姚云. 基于物质点法考虑剪胀特性的土质边坡稳定性分析[J]. 湖南交通科技,2017,43(2):82 − 85. [YAO Yun. Stability analysis of soil slope considering dilatancy characteristics based on material point method[J]. Hunan Communication Science and Technology,2017,43(2):82 − 85. (in Chinese)] YAO Yun. Stability analysis of soil slope considering dilatancy characteristics based on material point method［J］. Hunan Communication Science and Technology, 2017, 43（2）: 82 − 85. （in Chinese）
[17] 杨婷婷,杨永森,邱流潮. 基于物质点法的土体流动大变形过程数值模拟[J]. 工程地质学报,2018,26(6):1463 − 1472. [YANG Tingting,YANG Yongsen,QIU Liuchao. Mpm based numerical simulation of large deformation process of soil flow[J]. Journal of Engineering Geology,2018,26(6):1463 − 1472. (in Chinese with English abstract)] YANG Tingting, YANG Yongsen, QIU Liuchao. Mpm based numerical simulation of large deformation process of soil flow［J］. Journal of Engineering Geology, 2018, 26（6）: 1463 − 1472. （in Chinese with English abstract）
[18] QU C X,WANG G,FENG K W,et al. Large deformation analysis of slope failure using material point method with cross-correlated random fields[J]. Journal of Zhejiang University-Science A (Applied Physics & Engineering),2021,22(11):856 − 870
[19] YERRO A,ALONSO E E,PINYOL N M. Run-out of landslides in brittle soils[J]. Computers and Geotechnics,2016,80:427 − 439.
[20] LIU X,WANG Y,LI D Q. Investigation of slope failure mode evolution during large deformation in spatially variable soils by random limit equilibrium and material point methods[J]. Computers and Geotechnics,2019,111:301 − 312.
[21] 刘磊磊,梁昌奇,徐蒙,等. 考虑参数旋转各向异性空间变异性的边坡大变形概率分析[J]. 地球科学,2023,48(5):1836 − 1852. [LIU Leilei,LIANG Changqi,XU Meng,et al. Probabilistic analysis of large slope deformation considering soil spatial variability with rotated anisotropy[J]. Earth Science,2023,48(5):1836 − 1852. (in Chinese with English abstract)] LIU Leilei, LIANG Changqi, XU Meng, et al. Probabilistic analysis of large slope deformation considering soil spatial variability with rotated anisotropy［J］. Earth Science, 2023, 48（5）: 1836 − 1852. （in Chinese with English abstract）
[22] LIU L L,LIANG C Q,HUANG L,et al. Parametric analysis for the large deformation characteristics of unstable slopes with linearly increasing soil strength by the random material point method[J]. Computers and Geotechnics,2023,162:105661.
[23] LI X,TANG S,ZHENG Y,et al. Influence of the matrix of the soil-rock mixture on deformation and failure behaviors of the slope based on material point method[J]. Frontiers in Earth Science,2022,10:997928.
[24] 蒋涛,崔圣华,冉耀. 开挖和降雨耦合诱发滑坡机理分析——以四川万源前进广场滑坡为例[J]. 中国地质灾害与防治学报,2023,34(3):20 − 30. [JIANG Tao,CUI Shenghua,RAN Yao. Analysis of landslide mechanism induced by excavation and rainfall:A case study of the Qianjin Square landslide in Wanyuan City,Sichuan Province[J]. The Chinese Journal of Geological Hazard and Control,2023,34(3):20 − 30. (in Chinese with English abstract)] JIANG Tao, CUI Shenghua, RAN Yao. Analysis of landslide mechanism induced by excavation and rainfall: A case study of the Qianjin Square landslide in Wanyuan City, Sichuan Province［J］. The Chinese Journal of Geological Hazard and Control, 2023, 34（3）: 20 − 30. （in Chinese with English abstract）
[25] 张彦博,孙俊,陈涛,等. 贵州“7•23”水城滑坡触发机制及二次滑坡动力致灾分析[J/OL]. 中国地质灾害与防治学报,1 − 10[2024-09-04]. [Zhang Yanbo,Sun Jun,Chen Tao,et al. The triggering mechanism of the "7•23" Shuiceng landslide and the dynamic analysis of the secondary landslide [J/OL]. Chinese Journal of Geological Hazard and Control,1 − 10[2024-09-04]. ( in Chinese with English abstract)] Zhang Yanbo, Sun Jun, Chen Tao, et al. The triggering mechanism of the "7•23" Shuiceng landslide and the dynamic analysis of the secondary landslide ［J/OL］. Chinese Journal of Geological Hazard and Control, 1 − 10[2024-09-04]. （ in Chinese with English abstract）
[26] 顾福计,钱龙,王梦洁,等. 太行山河北段 “23•7” 强降雨引发的地质灾害规律研究[J]. 中国地质灾害与防治学报,2024,35(2):55 − 65. [GU Fuji,QIAN Long,WANG Mengjie,et al. Analysis of geological hazards caused by the “23•7” heavy rainfall in the northern section of Taihang Mountain in Hebei Province[J]. The Chinese Journal of Geological Hazard and Control,2024,35(2):55 − 65. (in Chinese with English abstract)] GU Fuji, QIAN Long, WANG Mengjie, et al. Analysis of geological hazards caused by the “23•7” heavy rainfall in the northern section of Taihang Mountain in Hebei Province［J］. The Chinese Journal of Geological Hazard and Control, 2024, 35（2）: 55 − 65. （in Chinese with English abstract）
[27] QIN J Y,MEI G,XU N X. Meshfree methods in geohazards prevention:A survey[J]. Archives of Computational Methods in Engineering,2022,29(5):3151 − 3182.
[28] WANG B,VARDON P J,HICKS M A. Rainfall-induced slope collapse with coupled material point method[J]. Engineering Geology,2018,239:1 − 12.
[29] JASSIM I,STOLLE D,VERMEER P. Two-phase dynamic analysis by material point method[J]. International Journal for Numerical and Analytical Methods in Geomechanics,2013,37(15):2502 − 2522.
[30] GIRARDI V,YERRO A,CECCATO F,et al. Modelling deformations in water retention structures with unsaturated material point method[J]. Proceedings of the Institution of Civil Engineers - Geotechnical Engineering,2021,174(5):577 − 592.
[31] HIGO Y,OKA F,KIMOTO S,et al. A coupled MPM-FDM analysis method for multi-phase elasto-plastic soils[J]. Soils and Foundations,2010,50(4):515 − 532.
[32] ZHENG X C,PISANÒ F,VARDON P J,et al. Fully implicit,stabilised MPM simulation of large-deformation problems in two-phase elastoplastic geomaterials[J]. Computers and Geotechnics,2022,147:104771.
[33] ABE K,SOGA K,BANDARA S. Material point method for coupled hydromechanical problems[J]. Journal of Geotechnical and Geoenvironmental Engineering,2014,140(3):04013033.
[34] SOŁOWSKI W T,SEYEDAN S. Granular Material Point Method:unsaturated soil modelling[J]. Geomechanics for Energy and the Environment,2023,34:100471.
[35] ZHAN Z Q,ZHOU C,LIU C Q,et al. Modelling hydro-mechanical coupled behaviour of unsaturated soil with two-phase two-point material point method[J]. Computers and Geotechnics,2023,155:105224.
[36] FENG K W,HUANG D R,WANG G. Two-layer material point method for modeling soil–water interaction in unsaturated soils and rainfall-induced slope failure[J]. Acta Geotechnica,2021,16(8):2529 − 2551.
[37] CECCATO F,YERRO A,GIRARDI V,et al. Two-phase dynamic MPM formulation for unsaturated soil[J]. Computers and Geotechnics,2021,129:103876.
[38] TRAN Q A,SOŁOWSKI W. Generalized Interpolation Material Point Method modelling of large deformation problems including strain-rate effects - Application to penetration and progressive failure problems[J]. Computers and Geotechnics,2019,106:249 − 265.
[39] 王升,曾鹏,李天斌,等. 土质滑坡失稳、运动及冲击压力物质点法模拟研究[J]. 工程地质学报,2022,30(4):1362 − 1370. [WANG Sheng,ZENG Peng,LI Tianbin,et al. Initiation,movement and impact simulation of soil landslide with material point method[J]. Journal of Engineering Geology,2022,30(4):1362 − 1370. (in Chinese with English abstract)] WANG Sheng, ZENG Peng, LI Tianbin, et al. Initiation, movement and impact simulation of soil landslide with material point method［J］. Journal of Engineering Geology, 2022, 30（4）: 1362 − 1370. （in Chinese with English abstract）
[40] WANG D,WANG B,YUAN W H,et al. Investigation of rainfall intensity on the slope failure process using GPU-accelerated coupled MPM[J]. Computers and Geotechnics,2023,163:105718.
[41] HE K,XI C J,LIU B,et al. MPM-based mechanism and runout analysis of a compound reactivated landslide[J]. Computers and Geotechnics,2023,159:105455.
[42] LEE W L,MARTINELLI M,SHIEH C L. An investigation of rainfall-induced landslides from the pre-failure stage to the post-failure stage using the material point method[J]. Frontiers in Earth Science,2021,9:764393.
[43] MOORMANN C,HAMAD F. MPM dynamic simulation of a seismically induced sliding mass[J]. IOP Conference Series:Earth and Environmental Science,2015,26:012024.
[44] ALSARDI A,YERRO A. Runout modeling of earthquake-triggered landslides with the material point method[C]//IFCEE 2021. Dallas,Texas. Reston,VA:American Society of Civil Engineers,2021:21–31.
[45] ALSARDI A,COPANA J,YERRO A. Modelling earthquake-triggered landslide runout with the material point method[J]. Proceedings of the Institution of Civil Engineers - Geotechnical Engineering,2021,174(5):563 − 576.
[46] HE M C,RIBEIRO E SOUSA L,MÜLLER A,et al. Numerical and safety considerations about the Daguangbao landslide induced by the 2008 Wenchuan earthquake[J]. Journal of Rock Mechanics and Geotechnical Engineering,2019,11(5):1019 − 1035.
[47] XU X R,JIN F,SUN Q C,et al. Three-dimensional material point method modeling of runout behavior of the Hongshiyan landslide[J]. Canadian Geotechnical Journal,2019,56(9):1318 − 1337.
[48] FENG K W,HUANG D R,WANG G,et al. Physics-based large-deformation analysis of coseismic landslides:A multiscale 3D SEM-MPM framework with application to the hongshiyan landslide[J]. Engineering Geology,2022,297:106487.
[49] FENG K W,WANG G,HUANG D R,et al. Material point method for large-deformation modeling of coseismic landslide and liquefaction-induced dam failure[J]. Soil Dynamics and Earthquake Engineering,2021,150:106907.
[50] BIELAK J. Domain reduction method for three-dimensional earthquake modeling in localized regions,part I:theory[J]. Bulletin of the Seismological Society of America,2003,93(2):817 − 824.
[51] YOSHIMURA C. Domain reduction method for three-dimensional earthquake modeling in localized regions,part II:verification and applications[J]. Bulletin of the Seismological Society of America,2003,93(2):825 − 841.
[52] KOHLER M,STOECKLIN A,PUZRIN A M. A MPM framework for large-deformation seismic response analysis[J]. Canadian Geotechnical Journal,2022,59(6):1046 − 1060.
[53] KOHLER M,HODEL D,KELLER L,et al. Case study of an active landslide at the flank of a water reservoir and its response during earthquakes[J]. Engineering Geology,2023,323:107243.
[54] KOHLER M,PUZRIN A M. Mechanism of co-seismic deformation of the slow-moving La sorbella landslide in Italy revealed by MPM analysis[J]. Journal of Geophysical Research (Earth Surface),2022,127(7):e2022JF006618.
[55] TRAN Q A,GRIMSTAD G,ALI GHOREISHIAN AMIRI S. MPMICE:A hybrid MPM-CFD model for simulating coupled problems in porous media. Application to earthquake-induced submarine landslides[J]. International Journal for Numerical Methods in Engineering,2024,125(5):e7383.
[56] FERNÁNDEZ F,VARGAS E,MULLER A L,et al. Material point method modeling in 3D of the failure and Run-out processes of the Daguangbao landslide[J]. Acta Geotechnica,2024,19(7):4277 − 4296.
[57] 吴方东,张巍,史卜涛,等. 堆载诱发型土质滑坡运动特征物质点法模拟[J]. 水文地质工程地质,2017,44(6):126 − 134. [WU Fangdong,ZHANG Wei,SHI Butao,et al. Run-out characteristic simulation of a surcharge-induced soil landslide using the material point method[J]. Hydrogeology & Engineering Geology,2017,44(6):126 − 134. (in Chinese with English abstract)] WU Fangdong, ZHANG Wei, SHI Butao, et al. Run-out characteristic simulation of a surcharge-induced soil landslide using the material point method［J］. Hydrogeology & Engineering Geology, 2017, 44（6）: 126 − 134. （in Chinese with English abstract）
[58] XIE T C,ZHU H H,ZHANG C X,et al. Modeling strip footings on slopes using the material point method[J]. Bulletin of Engineering Geology and the Environment,2023,82(4):99.
[59] ZHU H H,XIE T C,ZHANG W,et al. Numerical simulations of a strip footing on the soil slope with a buried pipe using the material point method[J]. International Journal of Geomechanics,2023,23(11):04023190.
[60] TRONCONE A,PUGLIESE L,CONTE E. Analysis of an excavation-induced landslide in stiff clay using the material point method[J]. Engineering Geology,2022,296:106479.
[61] GONZÁLEZ ACOSTA J L,VARDON P J,HICKS M A. Study of landslides and soil-structure interaction problems using the implicit material point method[J]. Engineering Geology,2021,285:106043.
[62] NG C W W,WANG C,CHOI C E,et al. Effects of barrier deformability on load reduction and energy dissipation of granular flow impact[J]. Computers and Geotechnics,2020,121:103445.
[63] CECCATO F,REDAELLI I,DI PRISCO C,et al. Impact forces of granular flows on rigid structures:comparison between discontinuous (DEM) and continuous (MPM) numerical approaches[J]. Computers and Geotechnics,2018,103:201 − 217.
[64] WYSER E,ALKHIMENKOV Y,JABOYEDOFF M,et al. Analytical and numerical solutions for three-dimensional granular collapses[J]. Geosciences,2023,13(4):119.
[65] LEI Z D,WU B S,WU S S,et al. A material point-finite element (MPM-FEM) model for simulating three-dimensional soil-structure interactions with the hybrid contact method[J]. Computers and Geotechnics,2022,152:105009.
[66] LEI X Q,CHEN X Q,YANG Z J,et al. A simple and robust MPM framework for modelling granular flows over complex terrains[J]. Computers and Geotechnics,2022,149:104867.
[67] CUOMO S,DI PERNA A,MARTINELLI M. Material point method (MPM) hydro-mechanical modelling of flows impacting rigid walls[J]. Canadian Geotechnical Journal,2021,58(11):1730 − 1743.
[68] LI X P,YAN Q W,ZHAO S X,et al. Investigation of influence of baffles on landslide debris mobility by 3D material point method[J]. Landslides,2020,17(5):1129 − 1143.
[69] ABE K,KONAGAI K. Numerical simulation for runout process of debris flow using depth-averaged material point method[J]. Soils and Foundations,2016,56(5):869 − 888.
[70] ZHAO Y D,CHOO J,JIANG Y P,et al. Coupled material point and level set methods for simulating soils interacting with rigid objects with complex geometry[J]. Computers and Geotechnics,2023,163:105708.
[71] LI X P,YAO J,SUN Y L,et al. Material point method analysis of fluid–structure interaction in geohazards[J]. Natural Hazards,2022,114(3):3425 − 3443.
[72] DI PERNA A,CUOMO S,MARTINELLI M. Empirical formulation for debris flow impact and energy release[J]. Geoenvironmental Disasters,2022,9(1):8.
[73] CUOMO S,DI PERNA A,MARTINELLI M. Analytical and numerical models of debris flow impact[J]. Engineering Geology,2022,308:106818.
[74] KAZMI Z A,KONAGAI K,IKEDA T. Field measurements and numerical simulation of debris flows from dolomite slopes destabilized during the 2005 Kashmir earthquake,Pakistan[J]. Journal of Earthquake Engineering,2014,18(3):364-388.
[75] WONG T,WEI Y J,JIE Y X. Global sensitivity analysis on debris flow energy dissipation of the artificial step-pool system[J]. Computers and Geotechnics,2022,147:104758.
[76] VICARI H,TRAN Q A,NORDAL S,et al. MPM modelling of debris flow entrainment and interaction with an upstream flexible barrier[J]. Landslides,2022,19(9):2101 − 2115.
[77] XIE X C,CECCATO F,ZHOU M L,et al. Hydro-mechanical behaviour of soils during water-soil gushing in shield tunnels using MPM[J]. Computers and Geotechnics,2022,145:104688.
[78] 谢小创,张冬梅. 基于物质点法的盾构隧道涌水涌砂模拟与应用[J]. 隧道建设(中英文),2023,43(6):1045 − 1056. [XIE Xiaochuang,ZHANG Dongmei. Simulation of water-soil gushing in shield tunnel based on material point method and its application[J]. Tunnel Construction,2023,43(6):1045 − 1056. (in Chinese with English abstract)] XIE Xiaochuang, ZHANG Dongmei. Simulation of water-soil gushing in shield tunnel based on material point method and its application［J］. Tunnel Construction, 2023, 43（6）: 1045 − 1056. （in Chinese with English abstract）
[79] 张春新,朱鸿鹄,李豪杰,等. 支护压力控制下隧道周围砂土变形破坏物质点法模拟[J]. 浙江大学学报(工学版),2021,55(7):1317 − 1326. [ZHANG Chunxin,ZHU Honghu,LI Haojie,et al. Material point method simulations of sand deformation and failure around tunnel controlled by support pressure[J]. Journal of Zhejiang University (Engineering Science),2021,55(7):1317 − 1326. (in Chinese with English abstract)] ZHANG Chunxin, ZHU Honghu, LI Haojie, et al. Material point method simulations of sand deformation and failure around tunnel controlled by support pressure［J］. Journal of Zhejiang University （Engineering Science）, 2021, 55（7）: 1317 − 1326. （in Chinese with English abstract）
[80] 王曼灵,李树忱,周慧颖,等. 基于改进对流粒子域插值物质点法的隧道大变形分析[J]. 岩土工程学报,2024,46(8):1632 − 1643. [WANG Manling,LI Shuchen,ZHOU Huiying,et al. Improved convective particle domain interpolation material point method for large deformation analysis of tunnels[J]. Chinese Journal of Geotechnical Engineering,2024,46(8):1632 − 1643. (in Chinese with English abstract)] WANG Manling, LI Shuchen, ZHOU Huiying, et al. Improved convective particle domain interpolation material point method for large deformation analysis of tunnels［J］. Chinese Journal of Geotechnical Engineering, 2024, 46（8）: 1632 − 1643. （in Chinese with English abstract）
[81] 王桂林,余浩,翟俊,等. 带裂损隧道在爆炸作用下的二次损伤响应规律[J]. 高压物理学报,2023,37(5):197 − 208. [WANG Guilin,YU Hao,ZHAI Jun,et al. Secondary damage response of cracked tunnels under explosion[J]. Chinese Journal of High Pressure Physics,2023,37(5):197 − 208. (in Chinese with English abstract)] WANG Guilin, YU Hao, ZHAI Jun, et al. Secondary damage response of cracked tunnels under explosion［J］. Chinese Journal of High Pressure Physics, 2023, 37（5）: 197 − 208. （in Chinese with English abstract）
[82] TU S Q,LI W,ZHANG C P,et al. Face stability analysis of tunnels in saturated soil considering soil-fluid coupling effect via material point method[J]. Computers and Geotechnics,2023,161:105592.
[83] CHENG X S,ZHENG G,SOGA K,et al. Post-failure behavior of tunnel heading collapse by MPM simulation[J]. Science China Technological Sciences,2015,58(12):2139 − 2152.
[84] LI S,ZHANG Y,WU J C,et al. Numerical modeling of pumping-induced earth fissures using coupled quasi-static material point method[J]. Journal of Geotechnical and Geoenvironmental Engineering,2023,149(9):04023065.
[85] 李克智,边树涛,郭晓辉. 应用物质点法实现非常规油气藏水平井压前模拟——以鄂尔多斯盆地杭锦旗区块为例[J]. 石油地质与工程,2023,37(6):109 − 113. [LI Kezhi,BIAN Shutao,GUO Xiaohui. Pre-pressure simulation of horizontal wells in conventional oil and gas reservoirs with material point method:by taking Hangjinqi Block in Ordos Basin as an example[J]. Petroleum Geology and Engineering,2023,37(6):109 − 113. (in Chinese with English abstract)] LI Kezhi, BIAN Shutao, GUO Xiaohui. Pre-pressure simulation of horizontal wells in conventional oil and gas reservoirs with material point method: by taking Hangjinqi Block in Ordos Basin as an example［J］. Petroleum Geology and Engineering, 2023, 37（6）: 109 − 113. （in Chinese with English abstract）
[86] LIANG D F,ZHAO X Y,SOGA K. Simulation of overtopping and seepage induced dike failure using two-point MPM[J]. Soils and Foundations,2020,60(4):978 − 988.
[87] TALBOT L E D,GIVEN J,TJUNG E Y S,et al. Modeling large-deformation features of the Lower San Fernando Dam failure with the Material Point Method[J]. Computers and Geotechnics,2024,165:105881.
[88] 徐云卿,周晓敏,赵世一,等. 基于B样条物质点法的溃坝流模拟研究[J]. 应用数学和力学,2023,44(8):921 − 930. [XU Yunqing,ZHOU Xiaomin,ZHAO Shiyi,et al. Simulation study on dam break flow based on the B-spline material point method[J]. Applied Mathematics and Mechanics,2023,44(8):921 − 930. (in Chinese with English abstract)] XU Yunqing, ZHOU Xiaomin, ZHAO Shiyi, et al. Simulation study on dam break flow based on the B-spline material point method［J］. Applied Mathematics and Mechanics, 2023, 44（8）: 921 − 930. （in Chinese with English abstract）
[89] REMMERSWAAL G,VARDON P J,HICKS M A. Evaluating residual dyke resistance using the Random Material Point Method[J]. Computers and Geotechnics,2021,133:104034.
[90] ZHAO X Y,LIANG D F,MARTINELLI M. Numerical simulations of dam-break floods with MPM[J]. Procedia Engineering,2017,175:133 − 140.
[91] 冯晓青,叶斌,苗沪生等. 基于固液两相耦合物质点法的饱和地基液化数值计算方法[J/OL]. 哈尔滨工业大学学报,1 − 14[2024-01-16] [FENG Xiaoqing,Ye Bin,Miao Husheng et al. Numerical calculation method of saturated foundation liquefaction based on solid-liquid two-phase coupling material point method [J/OL]. Journal of Harbin Institute of Technology,1 − 14[2024-01-16]. (in Chinese with English abstract)] FENG Xiaoqing, Ye Bin, Miao Husheng et al. Numerical calculation method of saturated foundation liquefaction based on solid-liquid two-phase coupling material point method ［J/OL］. Journal of Harbin Institute of Technology, 1 − 14[2024-01-16]. （in Chinese with English abstract）
[92] LIANG W J,ZHAO J D,WU H R,et al. Multiscale modeling of anchor pullout in sand[J]. Journal of Geotechnical and Geoenvironmental Engineering,2021,147(9):04021091.
[93] 高宇新,朱鸿鹄,张春新,等. 砂土中锚板上拔三维物质点法模拟研究[J]. 岩土工程学报,2022,44(2):295 − 304. [GAO Yuxin,ZHU Honghu,ZHANG Chunxin,et al. Three-dimensional uplift simulation of anchor plates in sand using material point method[J]. Chinese Journal of Geotechnical Engineering,2022,44(2):295 − 304. (in Chinese with English abstract)] GAO Yuxin, ZHU Honghu, ZHANG Chunxin, et al. Three-dimensional uplift simulation of anchor plates in sand using material point method［J］. Chinese Journal of Geotechnical Engineering, 2022, 44（2）: 295 − 304. （in Chinese with English abstract）
计量
- 文章访问数: 101
- HTML全文浏览量: 26
- PDF下载量: 31
邮件订阅
RSS