Analysis of soil cushion buffering characteristic for rockfall impact force through discrete element numerical simulation
-
摘要:
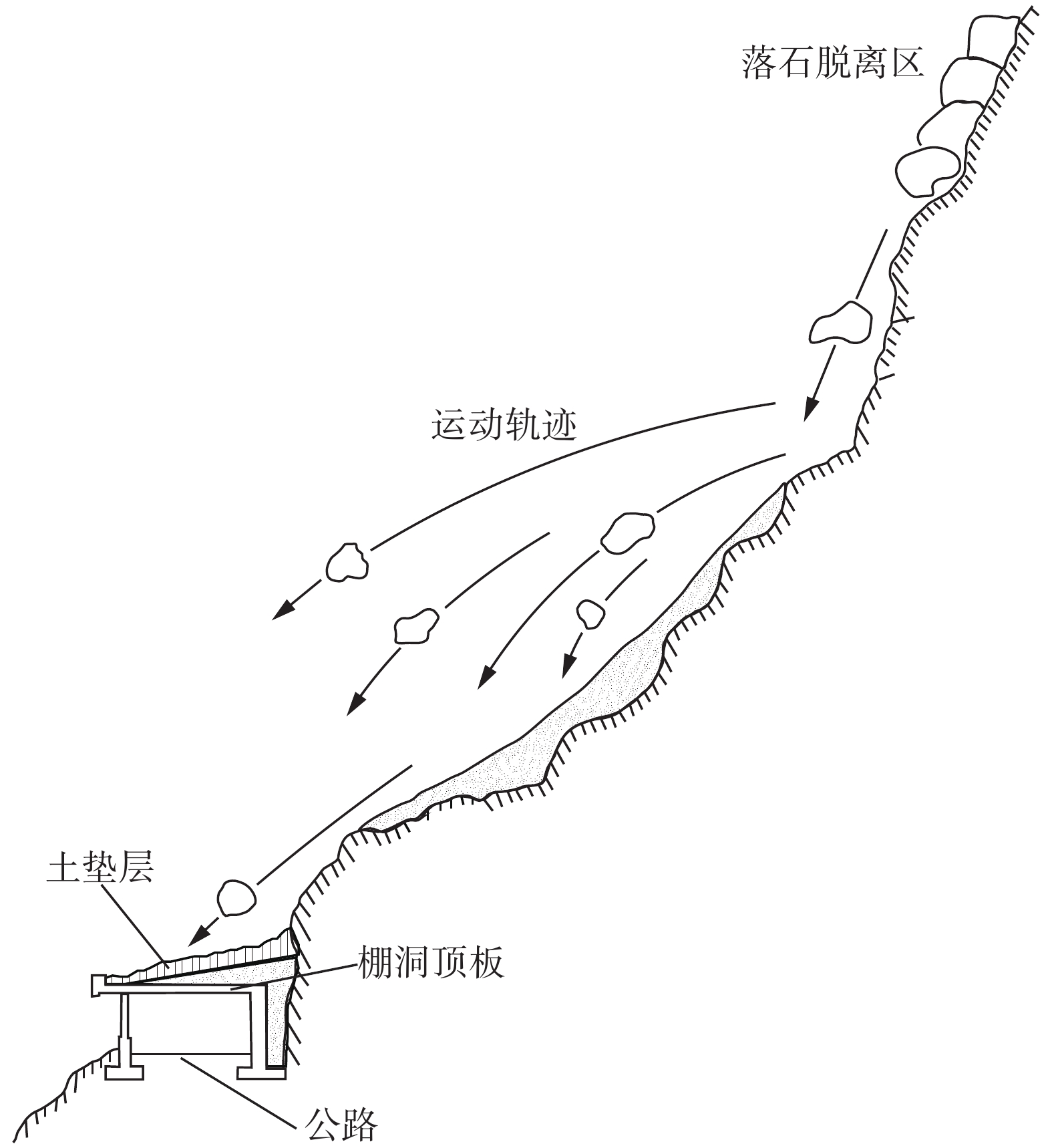
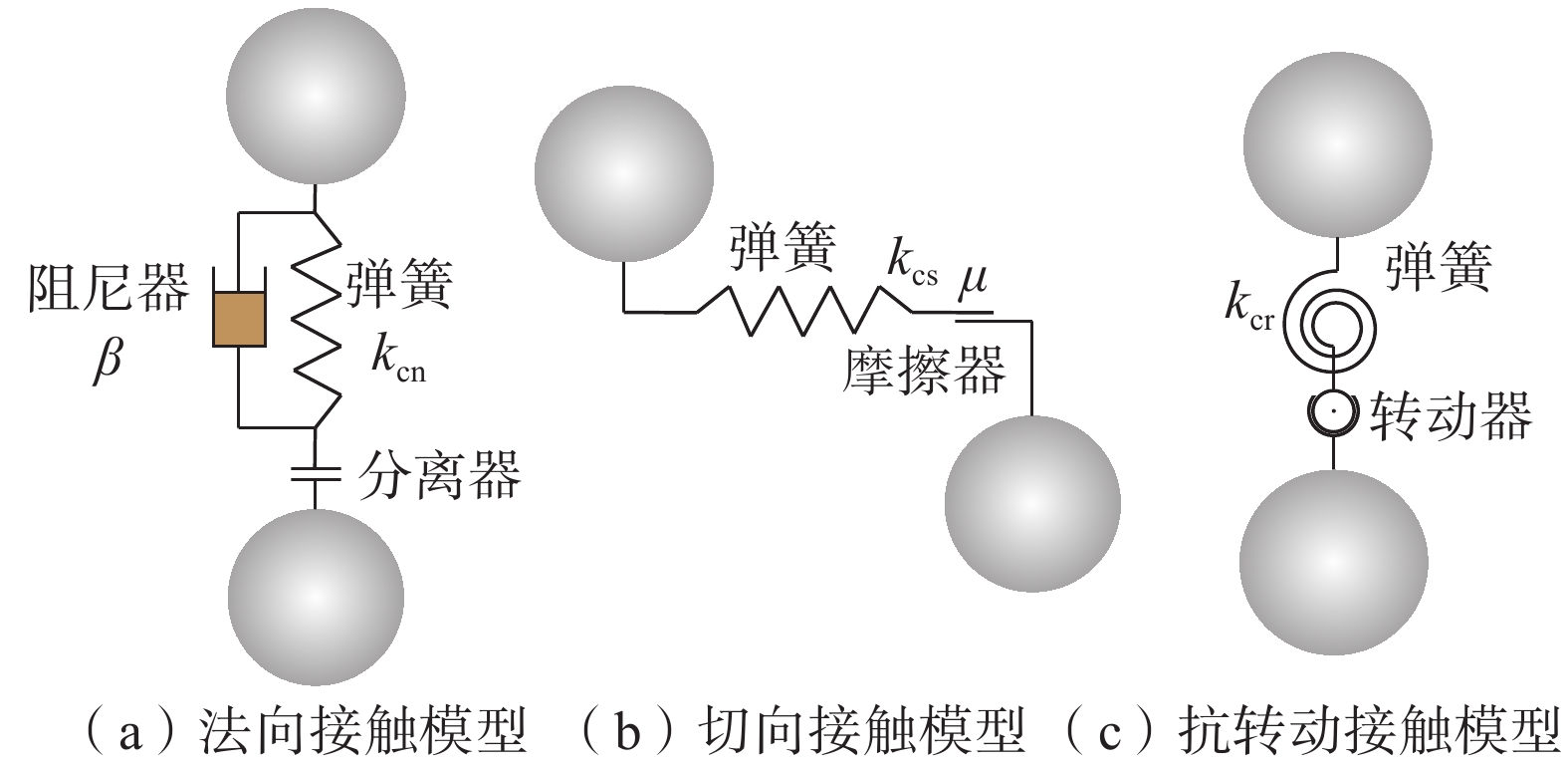
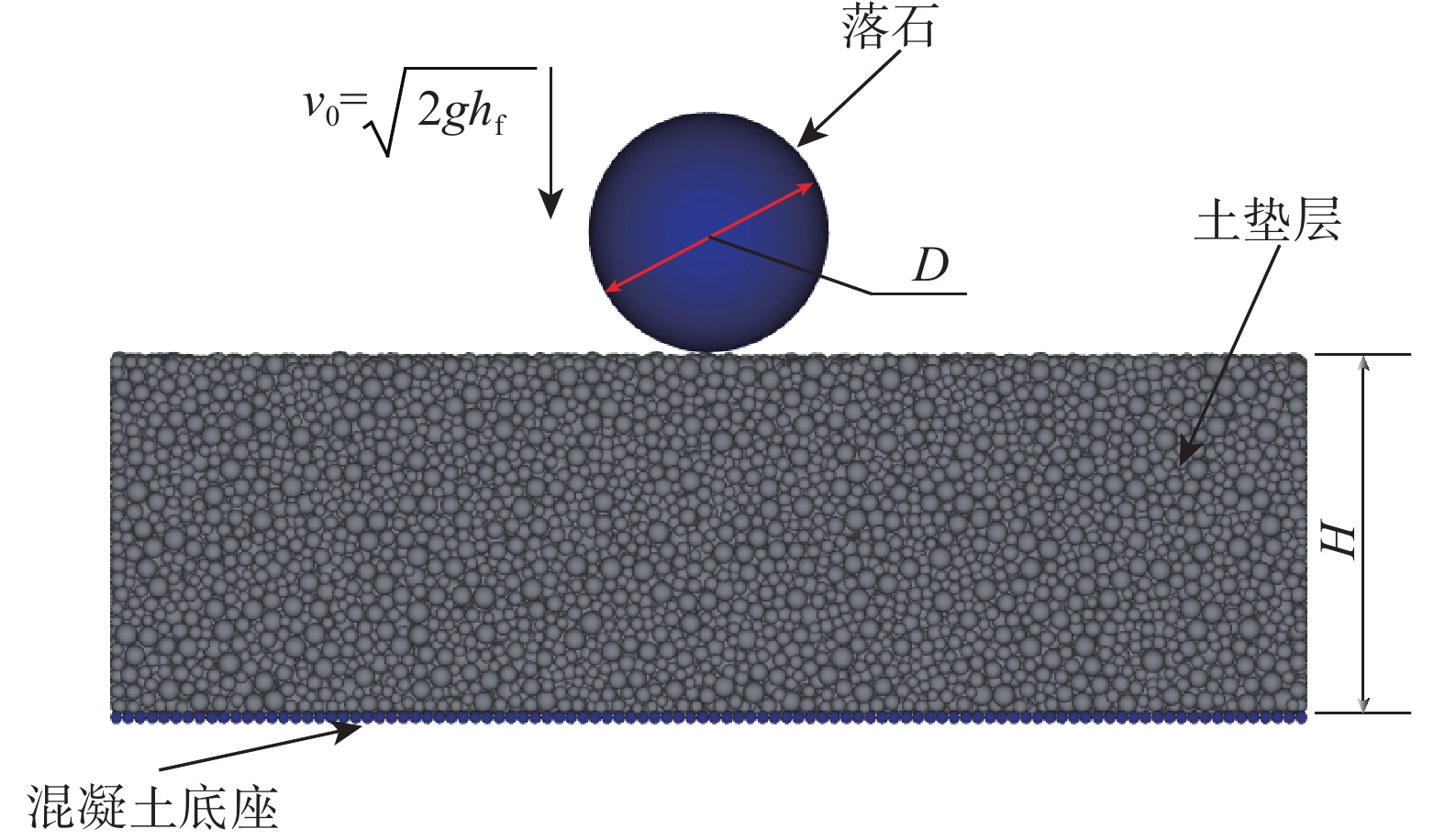
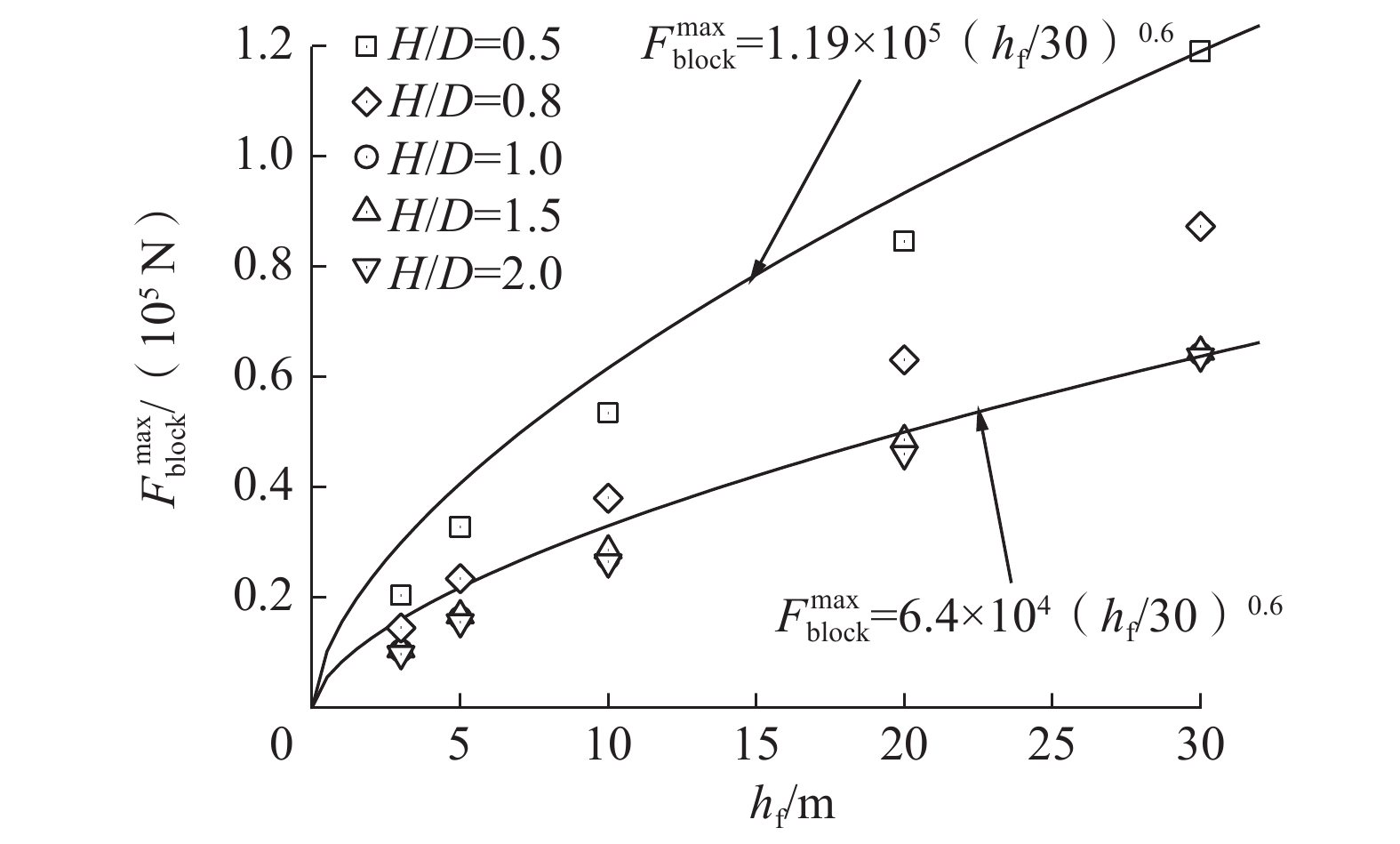
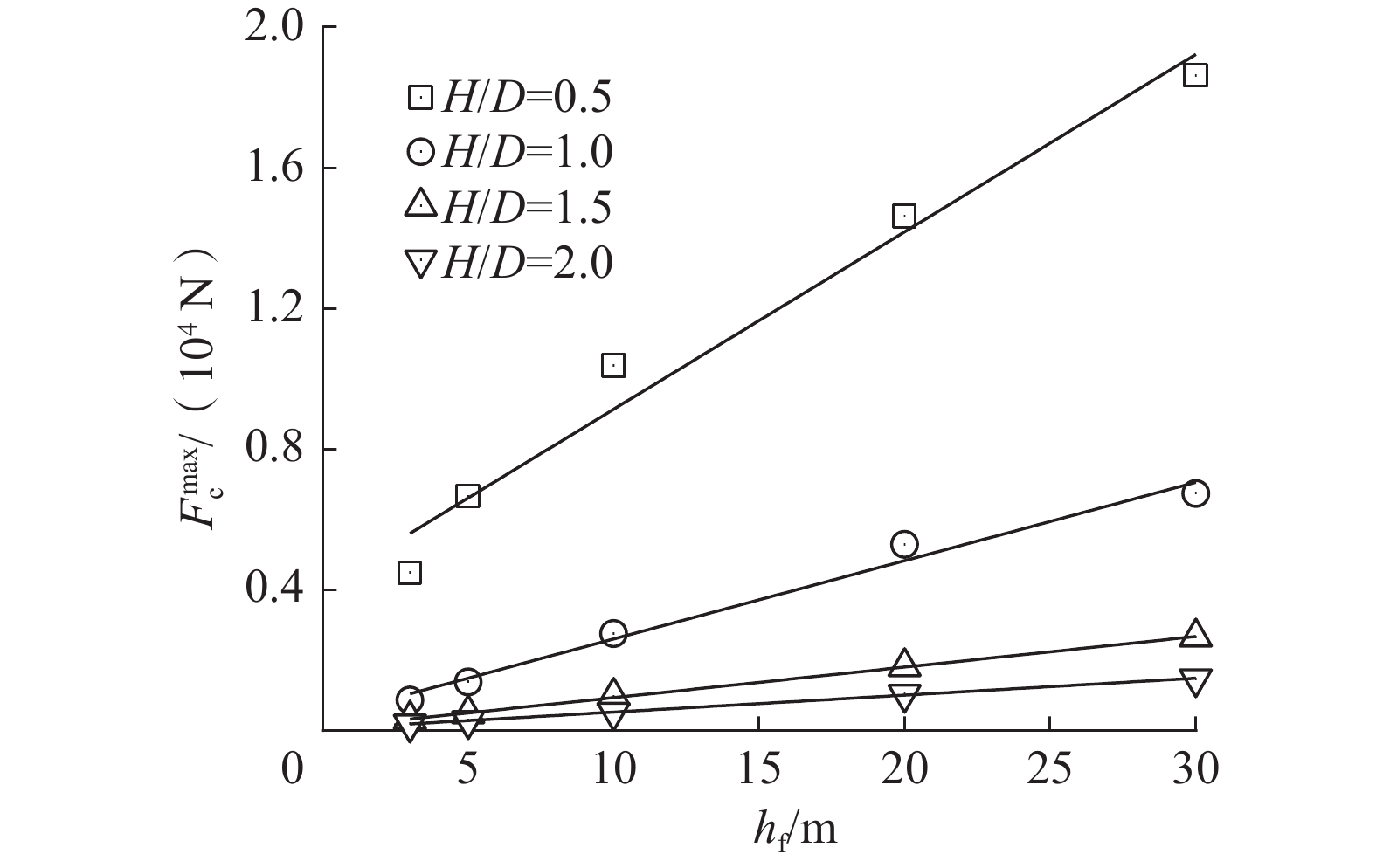
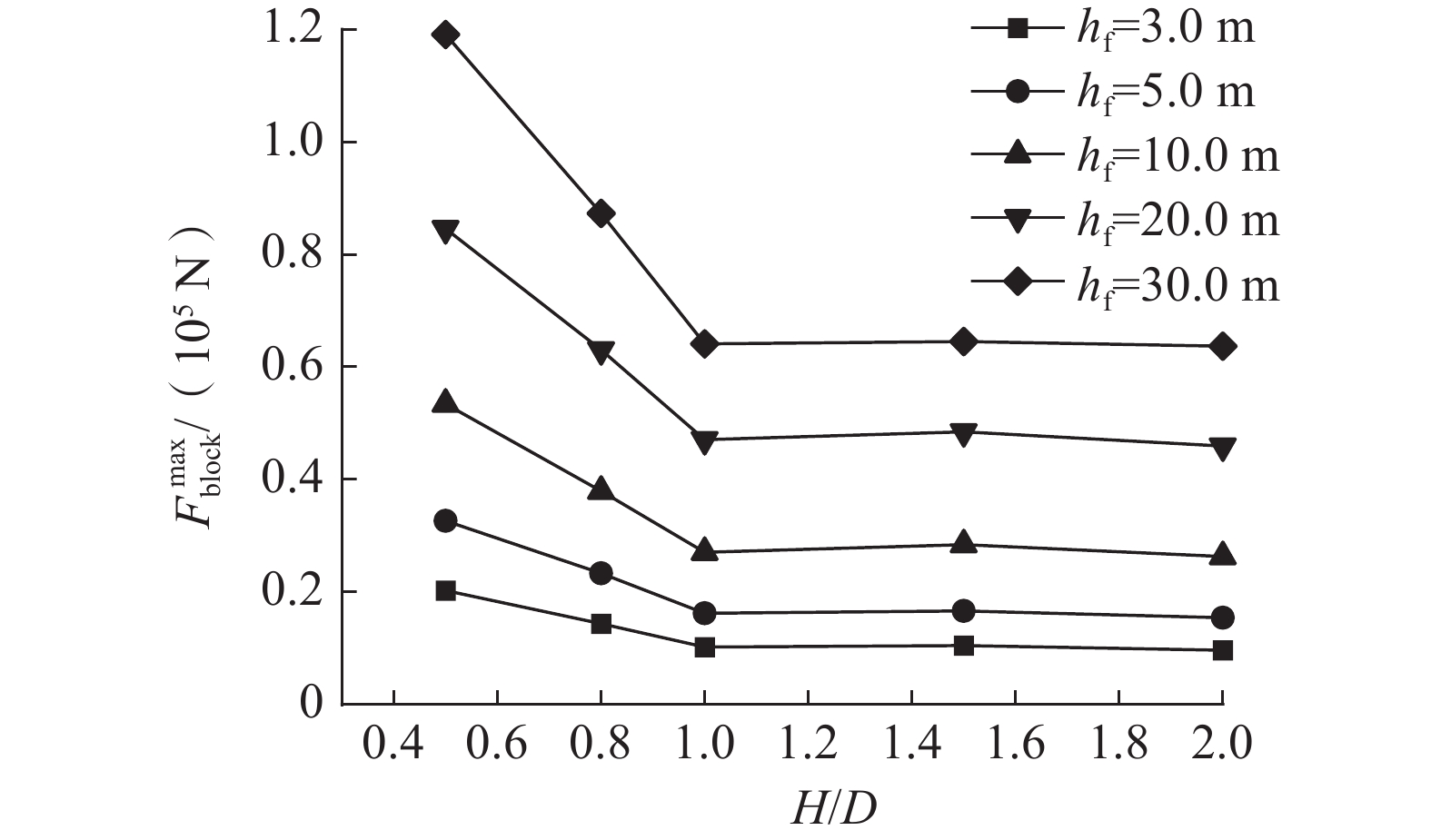
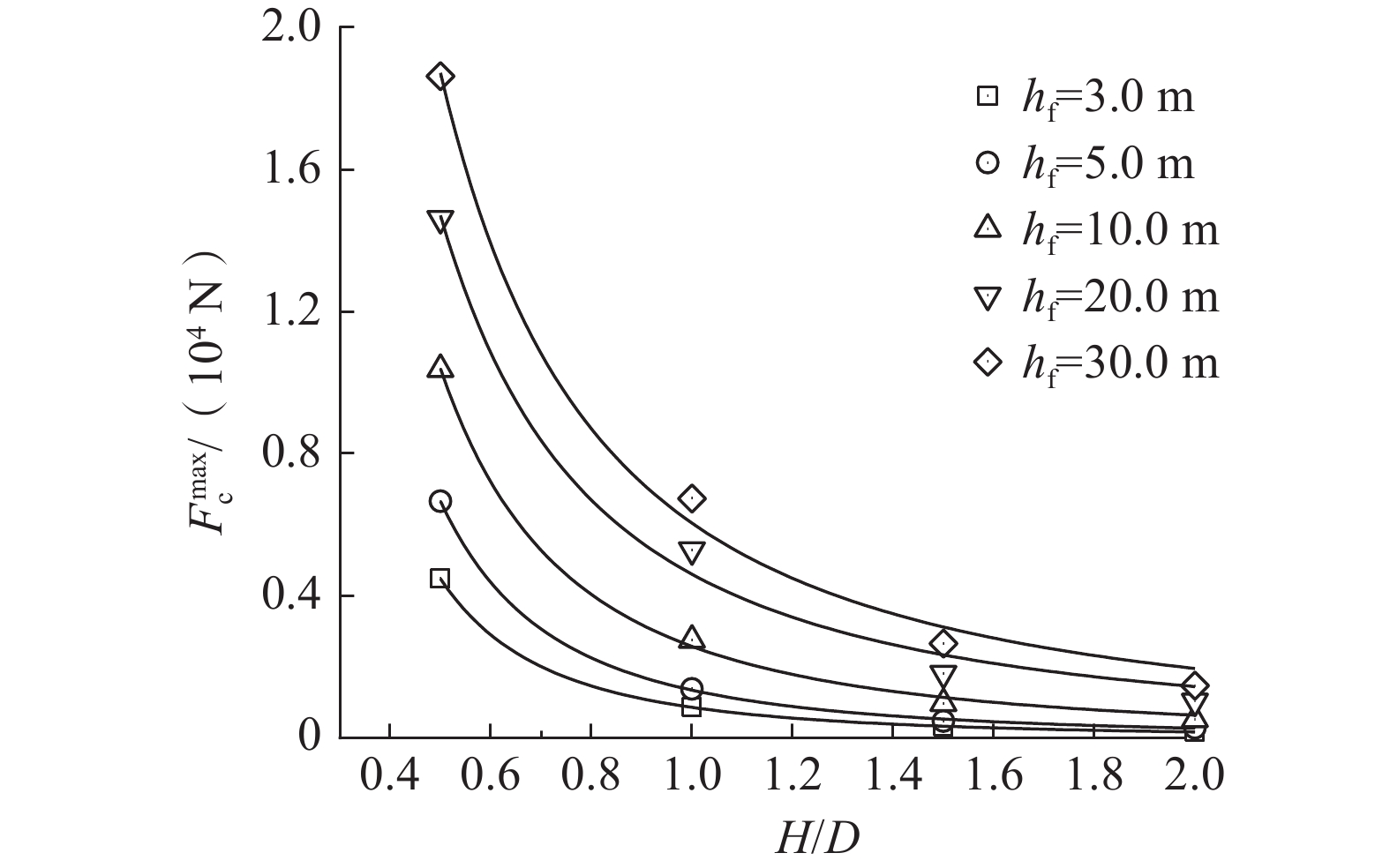
棚洞是我国西部山区防治崩塌落石灾害的主要工程措施之一。棚洞顶板以上通常铺设由砂或碎石组成的土垫层。土垫层的作用是避免落石直接冲击棚洞，缓冲落石的冲击力。长期以来，关于土垫层厚度对缓冲效果影响的研究较少，因此，还未形成统一的理论用于指导土垫层厚度的设计。文章运用离散单元方法建立落石冲击土垫层的数值模型，探究垫层厚度和落石下落高度对土垫层缓冲落石冲击力特性的影响。研究结果表明：落石冲击力峰值与落石下落高度呈幂函数关系，顶板中心力峰值与下落高度呈线性正相关关系；随着垫层厚度的增加，落石冲击力峰值减小，当垫层厚度增加到落石直径的1.0倍之后，落石冲击力峰值与垫层厚度无关；随垫层厚度的增大，顶板中心力峰值与落石冲击力峰值的比值减小，垫层缓冲效果增大，当垫层厚度增加到落石直径1.5倍之后，垫层缓冲效果增加不明显；垫层厚度建议取值为落石直径的1.5倍。
Abstract:Rock sheds is one of the main engineering solutions for mitigating rockfall disaster in the mountainous regions of western China. Typically, the roof of a rock shed is covered with a soil cushion composed of sand or gravel. The function of soil cushion is to avoid the direct impact of rockfall on the shed and absorb the impact force of the falling rocks. For a long time, there has been limited studies on the influence of soil cushion thickness on its buffering effect, leading to a lack of a unified theory guiding the design of soil cushion thickness. In this study, the discrete element method was employed to establish a numerical model of rockfall impacting onto soil cushion, and the influence of cushion thickness and rockfall falling height on the buffering characteristics of soil cushion for the rockfall impact force was investigated. The results show that there is a power function relationship between the peak of rockfall impact force and the rockfall falling height, along with a linear positive correlation between the peak of roof center force and the rockfall falling height. The peak of rockfall impact force decreases with increasing cushion thickness. Once the cushion thickness reaches 1.0 times of the diameter of rockfall, the peak of rockfall impact force becomes independent of cushion thickness as cushion thickness increases, the ratio of the peak bottom center force to the peak rockfall impact force decreases, indicating an enhancement in the soil cushion's buffering effect. However, when the cushion thickness reaches 1.5 times of the rockfall diameter, the enhancement in buffering effect becomes less significant. Therefore, the recommended cushion thickness is 1.5 times the rockfall diameter.
-
Keywords:
- rockfall /
- soil cushion /
- buffering /
- discrete element method /
- impact force
-
0. 引 言
近年来，中国建设开发了数十座软岩露天煤矿，在开采过程中采场及排土场均发生过一定规模的滑坡，对于采场底帮顺倾软岩边坡与顺倾软基底内排土场边坡滑坡灾害尤为严重。滑坡灾害直接影响剥采排工程的发展，造成人员伤害和设备损毁及地貌景观破坏，严重制约着露天矿的安全高效生产[1-2]，边坡稳定性治理问题已成为边坡工程领域亟待解决的难题之一。
目前国内外学者们应用不同理论对其展开大量有意义的研究，成果丰硕。王东等[3]综合运用极限平衡法及数值模拟法，分析了不同压帮高度下边坡稳定性变化规律，提出了逆倾软岩边坡变形的治理措施；刘子春等[4]以扎尼河露天矿为背景，通过分析扩帮、内排压角等治理措施的基础上，提出了一种条带式开采技术的边坡治理方案；陈毓等[5]采用ANSYS对黑山露天矿内排土场边坡稳定性和破坏机理进行了分析，揭示了内排土场滑坡模式为“坐落滑移式”滑动，运用削坡治理技术来保证内排土场稳定性；唐文亮等[6]系统分析了露天矿内排土场滑坡影响因素，提出了预留煤柱的滑坡治理方法；李伟[7]揭示了阴湾排土场边坡变形破坏机理并结合数值模拟法和极限平衡法，分析了内排不同压脚方案下边坡稳定性，提出了阴湾排土场滑坡治理措施；王刚等[8]基于有限元数值模拟法和极限平衡法，分析了边坡破坏机理并对边坡进行了稳定性计算，提出了削坡减载的治理措施。软岩露天煤矿采场边坡稳定性治理最经济有效的方式是内排追踪压帮，内排土场稳定是前提，但现有方法均是单一针对采场或排土场边坡稳定性分析和治理，未能同时兼顾采场与内排土场边坡的稳定性，对工程实际的指导性不强。
本文以贺斯格乌拉南露天煤矿首采区南帮为工程背景，在兼顾采场与内排土场边坡稳定性的基础上，提出了露天煤矿顺倾软岩边坡内排追踪压帮治理工程，为深入研究顺倾软岩露天煤矿边坡稳定性治理方法提供新的参考。
1. 边坡工程地质条件分析
贺斯格乌拉南露天煤矿设计生产能力为15 Mt/a，首采区南帮地层自上而下主要发育第四系、2煤组、2煤组与3煤组间夹石、3煤组、3煤组底板和盆地基底火山岩，含煤岩系主要以泥岩为主，全区可采的有2-1、3-1煤层，第四系以粉砂质黏土为主，局部夹黄-浅灰色细砂及含砾粗砂层，岩性较差，首采区土层赋存较薄，且其地层中多赋存软弱夹层，主要以3-1、3-4煤底板弱层主，属于典型的顺倾软岩边坡，岩土体物理力学指标如表1所示，典型工程地质剖面如图1所示。
表 1 岩土体物理力学指标Table 1. Physical and mechanical parameters of rock mass岩体名称 内摩擦角/（°） 黏聚力/kPa 容重/（kN·m−3） 弹性模量/MPa 泊松比 砂岩 26.00 65 19.6 35 0.42 粉质黏土 14.06 22 19.8 46 0.38 煤 29.00 85 12.1 40 0.35 泥岩 20.00 40 19.4 75 0.36 排弃物 14.49 20 19.0 60 0.40 弱层 6.00 0 19.1 20 0.42 回填岩石 20.00 40 19.0 − − 2. 采场底帮浅层边坡二维稳定性分析
影响顺倾软岩露天煤矿采场边坡稳定性的主控因素是弱层及其暴露长度，采用追踪压帮方式治理该类边坡稳定性时，可忽略软弱夹层为底界面的切层-顺层组合滑动模式[9-10]，仅考虑剪胀破坏模式。由于贺斯格乌拉南露天矿边坡体内赋存软弱夹层，主要以3-1、3-4煤底板弱层为主，顺倾角度大，岩质松软，对于此类边坡，浅部可通过平盘参数进行重新设计，深部必须利用三维效应，实现稳定性控制。可采用刚体极限平衡法中的剩余推力法对浅层边坡进行稳定性计算[11-12]。该方法的优点是可以用来计算求解给定任意边坡潜在滑面的稳定系数，并且可以考虑在复杂外力作用下的不同抗剪参数滑动岩体对边坡稳定性的影响。稳定系数求解公式为：
$$ {P_i} = \frac{{{W_i}\sin {\alpha _i}({W_i}\sin {\alpha _i}\tan {\varphi _i}) + {C_i}{L_i}}}{{{F_{\rm{s}}}}} + {\phi _i}{p_{i - 1}} $$ (1) $$ {\phi _i} = \frac{{\cos ({\alpha _{i - 1}} - {\alpha _i})\tan {\varphi _i}\sin ({\alpha _{i - 1}} - {\alpha _i})}}{{{F_{\rm{s}}}}} $$ (2) 式中：
${P_i}$ ——第$i$ 条块的剩余推力/kN；$ {W_i} $ ——第$i$ 条块的重量/（N·m−3）；$\alpha_i$ ——第$i$ 条块的滑面倾角/（°）；${\varphi _i}$ ——第$i$ 条块的推力传递系数；${C_i}$ ——第$i$ 条块的滑面黏聚力/kPa；${L_i}$ ——第$i$ 条块的底面长度/m；${\phi _i}$ ——第$i$ 条块的滑面摩擦角/（°）；${F_{\rm{s}}}$ ——稳定性系数。依据《煤炭工业露天矿设计规范》（GB 50197―2015）[13]综合考虑贺斯格乌拉南露天煤矿首采区南帮边坡服务年限、地质条件与力学参数的可靠性、潜在滑坡危害程度等，确定安全储备系数为1.2。
由于南帮压覆大量煤层，在保证安全前提下，为实现最大限度回采压覆的煤炭资源，需要对边坡形态重新设计。本文选取典型剖面为研究对象，浅层边坡形态按照40 m运输平盘、15 m保安平盘进行设计，深部利用横采内排三维支挡效应回采采场底帮深部压覆煤炭资源。通过上述情况对浅层边坡进行了分析，边坡稳定性计算结果如图2所示。
![]()
图 2 浅层边二维坡稳定性计算结果Figure 2. Calculation results of two-dimensional slope stability of the shallow side分析图2可知，浅部边坡形态可按照40 m运输平盘、15 m保安平盘进行设计，由于弱层上部存在煤岩支挡，边坡潜在滑坡模式为以圆弧为侧界面、3-1煤底板弱层为底界面、沿边坡坡脚处剪出，此时，浅层边坡能满足安全储备系数1.2的要求。
3. 采场底帮深部边坡稳定性三维效应分析
基于浅层边坡二维稳定性分析结果可知，实现深部稳定性控制，必须借助横采工作帮与内排土场的双重支挡作用进行压煤回采，因此提出了利用横采内排三维支挡效应回采采场深部压覆煤炭资源[14]。本文借助FLAC3D数值模拟软件，分析不同降深角度和不同追踪距离条件下的边坡三维稳定性，以期获得最优的边坡空间形态参数。
（1） 模型的建立
考虑到FLAC3D建模较为复杂，采用CAD与Rhino相结合的方法，首先在CAD中对剖面进行整理，然后在Rhino软件中进行模型成体与网格划分的处理，并用Griddle将网格导出，生成精细的六面体网格模型[15 − 17]，最后导入采用于FLAC3D进行数值模拟计算。为尽可能凸显边坡稳定性的三维效应，以南帮断面形态设计边坡为数值模拟对象，共计建立15种工况模型，模型如图3，追踪距离分别为50，100，200，300，400 m。为避免边界效应，在模型的底部和两侧分别施加水平和垂直位移约束，加载方式为重力加载[18]。
（2） 计算结果分析
由于计算结果过多，本文仅列举降深角度α=29°，追踪距离50，200，400 m工况下边坡位移云图（切割位置为沿模型走向中间处），如图4所示。南帮边坡三维稳定性计算结果如图5所示。
![]()
图 5 追踪距离与边坡稳定系数的关系曲线Figure 5. Relationship curve between tracking distance and slope stability coefficient分析图4、图5可知，追踪距离50 m时，三维支挡效应显著，边坡深部位移明显小于上部，发生以圆弧为侧界面、3-1煤底板弱层为底界面的切层-顺层-剪出滑动，稳定系数大于1.2。当追踪距离大于50 m时，通过对比分析不同深部边坡角（α）条件下的数值模拟结果可知，深部边坡角对边坡稳定性系数影响较小，随着追踪距离的增加，边坡的破坏模式过渡为以圆弧为侧界面、3-1煤底板弱层为底界面的切层-顺层滑动，并且此时边坡的稳定性不满足安全储备系数1.2要求。因此，内排土场追踪距离需控制在50 m以内，深部边坡角设计为29°。
4. 内排土场压帮边坡稳定性分析与治理
露天矿内排土场边坡稳定的主控因素是软弱基底，软弱基底分为自身软弱岩土层和受外界条影响转变为软弱岩土层2种类型。排土场下沉是软弱基底内排土场失稳的特征，主要现象是含有纵向强烈挤压区，基底上部岩层隆起，地面出现滑坡等[19 − 21]。在保证采场南帮安全的前提下降深至3-1煤底板，须借助横采工作帮与内排土场的双重支挡作用，内排土场稳定是前提[22]。由于内排土场基底为3-1、3-4煤底板弱层，顺倾角度较大，按照内排土场设计参数，其稳定性无法满足安全储备系数的要求[23]。从提供基底强度角度出发，采用破坏弱层回填岩石的方式提高内排土场边坡稳定性。按照排土台阶高度24 m、平盘宽度60 m、坡面角33°对不同内排压帮标高边坡稳定性进行试算，确定内排最小压帮标高为+844水平，因此本文分析了内排基于+844水平的压帮高度下内排土场基底不同的处理方式时的边坡稳定性计算结果如图6—7所示，边坡稳定性与破坏弱层回填岩石范围关系曲线如图8所示。
![]()
图 6 3-1煤层内排基底不同处理方式下边坡稳定性计算结果Figure 6. Calculation results of slope stability under different treatment methods of inner row basement (3-1)分析图6—图8可知，当内排基于+844的压帮高度，内排基底3-1底板弱层完全破坏并回填岩石，破坏3-4底板弱层并回填岩石倾向长度达60 m时，内排土场及其与采场南帮复合边坡稳定性均可满足安全系数1.2要求。边坡稳定性随破坏底板弱层回填岩石范围的增大呈正指数函数规律提高，随着回填岩石范围长度的不断增加，边坡稳定性系数不断提高。采用破坏弱层回填岩石的基底处理方法，既保证了边坡的稳定又规避了过渡处理基底的生产成本。
![]()
图 7 3-4煤层内排基底不同处理方式下边坡稳定性计算结果Figure 7. Calculation results of slope stability under different treatment methods of inner row basement (3-4)![]()
图 8 边坡稳定性与破坏弱层回填岩石范围关系曲线Figure 8. Relationship curve between slope stability and the extent of backfill rocks in the weak layer5. 结 论
（1） 弱层暴露长度是露天矿顺倾软岩边坡稳定性的主控因素，据此提出了露天矿顺倾软岩边坡内排追踪压帮治理工程，可最大限度的安全回收边坡压覆煤炭资源。
（2） 控制采场与内排土场间的追踪距离是改善边坡稳定性的有效途径。随着追踪距离的增加，边坡破坏模式从以圆弧为侧界面、弱层为底界面的切层-顺层-剪出滑动逐渐过渡为以圆弧为侧界面、弱层为底界面的切层-顺层滑动。
（3） 内排土场及其与采场构成的复合边坡稳定性随破坏底板弱层回填岩石范围的增大呈指数函数规律提高，随着回填岩石范围长度的不断增加，边坡稳定性系数不断提高。
（4） 贺斯格乌拉南露天煤矿首采区南帮浅部边坡留设40 m运输平盘、15 m保安平盘，底帮深部边坡角29°，追踪距离控制在50 m之内时可满足安全要求；内排基底弱层完全破坏并回填岩石倾向长度60 m时可满足安全需求。
-
![]()
图 3 落石冲击土垫层离散元模型
Figure 3. Discrete element model of rockfall impacting the soil cushion layer
![]()
图 4 落石冲击力和顶板中心力随时间演化曲线（hf = 3.0 m，H = 30.0 cm）
Figure 4. Time evolution curves of the impact force and the roof center force (hf = 3.0 m, H = 30.0 cm)
![]()
图 5 落石冲击力峰值与下落高度的关系
Figure 5. Dependence of the maximum rockfall impact force on the falling height
![]()
图 6 顶板中心力峰值与落石下落高度的关系
Figure 6. Dependence of the maximum roof center force on the falling height
![]()
图 7 落石冲击力峰值与标准化垫层厚度的关系
Figure 7. Dependence of the maximum rockfall impact force on the normalized soil cushion thickness
![]()
图 8 顶板中心力峰值与标准化垫层厚度的关系
Figure 8. Dependence of the maximum roof center force on the normalized soil cushion thickness
![]()
图 9 顶板中心力峰值和落石冲击力峰值的比值与落石下落高度的关系
Figure 9. Dependence of the ration between the maximum roof center force and the maximum rockfall impact force on the falling height
表 1 数值模型输入参数
Table 1 Input parameters of the numerical model
变量 数值 土垫层颗粒直径/cm [1.0, 2.0] 土颗粒密度/（kg·m−3） 2698.2 颗粒杨氏模量/MPa 1×102 颗粒泊松比 0.25 颗粒阻尼系数 0.01 颗粒摩擦系数 0.6 颗粒塑性力矩系数 0.15 计算时步/s 10−6 重力加速/（m·s−2） 9.81 
下载: 导出CSV
-
[1] 何思明,王东坡,吴永,等. 崩塌滚石灾害的力学机理与防治技术[J]. 自然杂志, 2014, 36(5):336 − 345. [HE Siming, WANG Dongpo, WU Yong, et al. Formation mechanism and key prevention technology of rockfalls[J]. Chinese Journal of Nature, 2014, 36(5):336 − 345. (in Chinese with English abstract)] HE Siming, WANG Dongpo, WU Yong, et al. Formation mechanism and key prevention technology of rockfalls［J］. Chinese Journal of Nature, 2014, 36（5）: 336 − 345. （in Chinese with English abstract）
[2] 王明辉,曹熙平,谯立家. 危岩体精细调查与崩塌过程三维场景模拟——以西南某水电站高边坡为例[J]. 中国地质灾害与防治学报,2023,34(6):86 − 96. [WANG Minghui,CAO Xiping,QIAO Lijia. Comprehensive analysis of hazardous rock mass and simulation of potential rockfall processes using 3D terrain model: a case study of the high cut slope near damsite of a hydropower station in Southern China[J]. The Chinese Journal of Geological Hazard and Control,2023,34(6):86 − 96. (in Chinese with English abstract)] WANG Minghui, CAO Xiping, QIAO Lijia. Comprehensive analysis of hazardous rock mass and simulation of potential rockfall processes using 3D terrain model: a case study of the high cut slope near damsite of a hydropower station in Southern China［J］. The Chinese Journal of Geological Hazard and Control, 2023, 34（6）: 86 − 96. （in Chinese with English abstract）
[3] 曾启强,王立朝,刘伟,等. 广州地区岩质边坡崩塌影响范围计算方法初探[J]. 水文地质工程地质,2023,50(5):159 − 168. [ZENG Qiqiang,WANG Lichao,LIU Wei,et al. Calculation methods of the collapse influence range of a simple rock slope in the Guangzhou Area[J]. Hydrogeology & Engineering Geology,2023,50(5):159 − 168. (in Chinese with English abstract)] ZENG Qiqiang, WANG Lichao, LIU Wei, et al. Calculation methods of the collapse influence range of a simple rock slope in the Guangzhou Area［J］. Hydrogeology & Engineering Geology, 2023, 50（5）: 159 − 168. （in Chinese with English abstract）
[4] 张路青,杨志法,许兵. 滚石与滚石灾害[J]. 工程地质学报,2004,12(3):225 − 231. [ZHANG Luqing,YANG Zhifa,XU Bing. Rock falls and rock fall hazards[J]. Journal of Engineering Geology,2004,12(3):225 − 231. (in Chinese with English abstract)] ZHANG Luqing, YANG Zhifa, XU Bing. Rock falls and rock fall hazards［J］. Journal of Engineering Geology, 2004, 12（3）: 225 − 231. （in Chinese with English abstract）
[5] 庞鑫,袁明,卢渊,等. 基于无人机LiDAR仿地飞行技术的高陡边坡危岩体快速识别方法[J]. 地质科技通报,2023,42(6):21 − 30. [PANG Xin,YUAN Ming,LU Yuan,et al. Rapid identification method for the dangerous rock mass of a high-steep slope based on UAV LiDAR and ground imitation flight[J]. Bulletin of Geological Science and Technology,2023,42(6):21 − 30. (in Chinese with English abstract)] PANG Xin, YUAN Ming, LU Yuan, et al. Rapid identification method for the dangerous rock mass of a high-steep slope based on UAV LiDAR and ground imitation flight［J］. Bulletin of Geological Science and Technology, 2023, 42（6）: 21 − 30. （in Chinese with English abstract）
[6] 石润,李嘉雨,陈明浩,等. 基于AHP-3DEC的危岩落石危险性分区与评价[J]. 中国地质灾害与防治学报,2023,34(3):127 − 135. [SHI Run,LI Jiayu,CHEN Minghao,et al. Hazard zoning and assessment of rockfalls based on AHP-3DEC[J]. The Chinese Journal of Geological Hazard and Control,2023,34(3):127 − 135. (in Chinese with English abstract)] SHI Run, LI Jiayu, CHEN Minghao, et al. Hazard zoning and assessment of rockfalls based on AHP-3DEC［J］. The Chinese Journal of Geological Hazard and Control, 2023, 34（3）: 127 − 135. （in Chinese with English abstract）
[7] 姚昌银. 落石冲击力的扩散机制[D]. 重庆:重庆交通大学,2018. [YAO Changyin. Diffusion mechanism of rockfall impact force[D]. Chongqing:Chongqing Jiaotong University,2018. (in Chinese with English abstract)] YAO Changyin. Diffusion mechanism of rockfall impact force［D］. Chongqing: Chongqing Jiaotong University, 2018. （in Chinese with English abstract）
[8] 刘洋. 滚石冲击棚洞防护结构动力响应及作用机理研究[D]. 成都:成都理工大学, 2017. [LIU Yang. Study on dynamic response and action mechanism of protective structure of shed tunnel impacted by rolling stone[D]. Chengdu:Chengdu University of Technology, 2017. (in Chinese with English abstract)] LIU Yang. Study on dynamic response and action mechanism of protective structure of shed tunnel impacted by rolling stone［D］. Chengdu: Chengdu University of Technology, 2017. （in Chinese with English abstract）
[9] 王玉锁. 落石冲击下拱形明洞结构概率可靠度分析[M]. 成都:西南交通大学出版社,2017. [WANG Yusuo. Probabilistic reliability analysis of arch open-cut tunnel structure under rockfall impact[M]. Chengdu:Southwest Jiaotong University Press,2017. (in Chinese)] WANG Yusuo. Probabilistic reliability analysis of arch open-cut tunnel structure under rockfall impact［M］. Chengdu: Southwest Jiaotong University Press, 2017. （in Chinese）
[10] 黄维, 艾东, 胡胜华, 等. 鄂西山区崩塌落石运动特征及危险性分析——以远安县瓦坡崩塌区为例[J]. 中国地质灾害与防治学报,2022,33(6):37 − 43. [HUANG Wei, AI Dong, HU Shenghua, et al. Characteristics of rockfall trajectory and hazard assessment in western Hubei Province:A case study of the Wapo collapse area in Yuan’an County[J]. The Chinese Journal of Geological Hazard and Control,2022,33(6):37 − 43. (in Chinese with English abstract)] HUANG Wei, AI Dong, HU Shenghua, et al. Characteristics of rockfall trajectory and hazard assessment in western Hubei Province: A case study of the Wapo collapse area in Yuan’an County［J］. The Chinese Journal of Geological Hazard and Control, 2022, 33（6）: 37 − 43. （in Chinese with English abstract）
[11] 杨涛,邓荣贵,刘小丽. 四川地区地震崩塌滑坡的基本特征及危险性分区[J]. 山地学报,2002,20(4):456 − 460. [YANG Tao,DENG Ronggui,LIU Xiaoli. The distributing and subarea character of the seismic landslides in Sichuan[J]. Journal of Mountain Research,2002,20(4):456 − 460. (in Chinese with English abstract)] YANG Tao, DENG Ronggui, LIU Xiaoli. The distributing and subarea character of the seismic landslides in Sichuan［J］. Journal of Mountain Research, 2002, 20（4）: 456 − 460. （in Chinese with English abstract）
[12] 何思明,王东坡,吴永. 崩塌滚石灾害形成演化机理与减灾关键技术[M]. 北京:科学出版社,2015. [HE Siming,WANG Dongpo,WU Yong. Formation and evolution mechanism of rockfall disaster and key technology of disaster reduction[M]. Beijing:Science Press,2015. (in Chinese)] HE Siming, WANG Dongpo, WU Yong. Formation and evolution mechanism of rockfall disaster and key technology of disaster reduction［M］. Beijing: Science Press, 2015. （in Chinese）
[13] YAN Peng,ZHANG Jinhua,FANG Qin,et al. Numerical simulation of the effects of falling rock’s shape and impact pose on impact force and response of RC slabs[J]. Construction and Building Materials,2018,160:497 − 504. DOI: 10.1016/j.conbuildmat.2017.11.087
[14] 袁博,祝介旺. 滚石冲击下棚洞破坏动力响应分析及改进对策——以川藏公路(安久拉山南麓)门式棚洞为例[J]. 水文地质工程地质,2019,46(6):57 − 66. [YUAN Bo,ZHU Jiewang. Dynamic response analyses and improvement countermeasures of shed-tunnel destruction under rolling stone impact:A case study of the shed-tunnel in the southern foot of the Anjiula Mountain on the Sichuan-Tibet Highway[J]. Hydrogeology & Engineering Geology,2019,46(6):57 − 66. (in Chinese with English abstract)] YUAN Bo, ZHU Jiewang. Dynamic response analyses and improvement countermeasures of shed-tunnel destruction under rolling stone impact: A case study of the shed-tunnel in the southern foot of the Anjiula Mountain on the Sichuan-Tibet Highway［J］. Hydrogeology & Engineering Geology, 2019, 46（6）: 57 − 66. （in Chinese with English abstract）
[15] 何思明,李新坡,吴永. 考虑弹塑性变形的泥石流大块石冲击力计算[J]. 岩石力学与工程学报,2007,26(8):1664 − 1669. [HE Siming,LI Xinpo,WU Yong. Calculation of impact force of outrunner blocks in debris flow considering elastoplastic deformation[J]. Chinese Journal of Rock Mechanics and Engineering,2007,26(8):1664 − 1669. (in Chinese with English abstract)] HE Siming, LI Xinpo, WU Yong. Calculation of impact force of outrunner blocks in debris flow considering elastoplastic deformation［J］. Chinese Journal of Rock Mechanics and Engineering, 2007, 26（8）: 1664 − 1669. （in Chinese with English abstract）
[16] 何思明,李新坡,吴永. 滚石冲击荷载作用下土体屈服特性研究[J]. 岩石力学与工程学报,2008,27(增刊1):2973 − 2977. [HE Siming,LI Xinpo,WU Yong. Research on yield property of soil under rock-fall impact[J]. Chinese Journal of Rock Mechanics and Engineering,2008,27(Sup):2973 − 2977. (in Chinese with English abstract)] HE Siming, LI Xinpo, WU Yong. Research on yield property of soil under rock-fall impact［J］. Chinese Journal of Rock Mechanics and Engineering, 2008, 27（Sup）: 2973 − 2977. （in Chinese with English abstract）
[17] 何思明. 滚石对防护结构的冲击压力计算[J]. 工程力学,2010,27(9):175 − 180. [HE Siming. Calculation of compact pressure of rock-fall on shield structures[J]. Engineering Mechanics,2010,27(9):175 − 180. (in Chinese with English abstract)] HE Siming. Calculation of compact pressure of rock-fall on shield structures［J］. Engineering Mechanics, 2010, 27（9）: 175 − 180. （in Chinese with English abstract）
[18] 何思明,沈均,罗渝,等. 滚石坡面法向冲击动力响应特性研究[J]. 工程力学,2011,28(6):118 − 124. [HE Siming,SHEN Jun,LUO Yu,et al. Study on the characteristics of normal impact of post-earthquake rock-fall on slope[J]. Engineering Mechanics,2011,28(6):118 − 124. (in Chinese with English abstract)] HE Siming, SHEN Jun, LUO Yu, et al. Study on the characteristics of normal impact of post-earthquake rock-fall on slope［J］. Engineering Mechanics, 2011, 28（6）: 118 − 124. （in Chinese with English abstract）
[19] WANG Yusuo,XU Ming,YANG Chao,et al. Effects of elastoplastic strengthening of gravel soil on rockfall impact force and penetration depth[J]. International Journal of Impact Engineering,2020,136:103411. DOI: 10.1016/j.ijimpeng.2019.103411
[20] 何思明,吴永,沈均. 泥石流大块石冲击力的简化计算[J]. 自然灾害学报,2009,18(5):51 − 56. [HE Siming,WU Yong,SHEN Jun. Simplified calculation of impact force of massive stone in debris flow[J]. Journal of Natural Disasters,2009,18(5):51 − 56(in Chinese with English abstract)] HE Siming, WU Yong, SHEN Jun. Simplified calculation of impact force of massive stone in debris flow［J］. Journal of Natural Disasters, 2009, 18（5）: 51 − 56（in Chinese with English abstract）
[21] WANG Xing,XIA Yongxu,ZHOU Tianyue. Theoretical analysis of rockfall impacts on the soil cushion layer of protective structures[J]. Advances in Civil Engineering,2018:1 − 18.
[22] 候天兴,杨兴国,黄成,等. 基于冲量定理的滚石对构筑物冲击力计算方法[J]. 岩石力学与工程学报,2015,34(增刊1):3116 − 3122. [HOU Tianxing,YANG Xingguo,HUANG Cheng,et al. A calculation method based on impulse theorem to determine impact force of rockfall on structure[J]. Chinese Journal of Rock Mechanics and Engineering,2015,34(Sup 1):3116 − 3122. (in Chinese with English abstract)] HOU Tianxing, YANG Xingguo, HUANG Cheng, et al. A calculation method based on impulse theorem to determine impact force of rockfall on structure［J］. Chinese Journal of Rock Mechanics and Engineering, 2015, 34（Sup 1）: 3116 − 3122. （in Chinese with English abstract）
[23] WANG Baolin,CAVERS D S. A simplified approach for rockfall ground penetration and impact stress calculations[J]. Landslides,2008,5(3):305 − 310. DOI: 10.1007/s10346-008-0123-6
[24] DI PRISCO C,VECCHIOTTI M. A rheological model for the description of boulder impacts on granular strata[J]. Géotechnique,2006,56(7):469 − 482.
[25] ZHANG Guangcheng,TANG Huiming,XIANG Xin,et al. Theoretical study of rockfall impacts based on logistic curves[J]. International Journal of Rock Mechanics and Mining Sciences,2015,78:133 − 143. DOI: 10.1016/j.ijrmms.2015.06.001
[26] 罗杰,肖建春,马克俭,等. 落石冲击下多种类型土壤缓冲性能研究[J]. 防灾减灾工程学报,2019,39(1):164 − 170. [LUO Jie,XIAO Jianchun,MA Kejian,et al. Study on buffering performance of various types of soils under rockfall impact[J]. Journal of Disaster Prevention and Mitigation Engineering,2019,39(1):164 − 170. (in Chinese with English abstract)] LUO Jie, XIAO Jianchun, MA Kejian, et al. Study on buffering performance of various types of soils under rockfall impact［J］. Journal of Disaster Prevention and Mitigation Engineering, 2019, 39（1）: 164 − 170. （in Chinese with English abstract）
[27] 王林峰,刘丽,唐芬,等. 基于落石棚洞冲击试验的落石冲击力研究[J]. 防灾减灾工程学报,2018,38(6):973 − 979. [WANG Linfeng,LIU Li,TANG Fen,et al. Study on impact force of rockfall impact experiment on shed tunnel[J]. Journal of Disaster Prevention and Mitigation Engineering,2018,38(6):973 − 979. (in Chinese with English abstract)] WANG Linfeng, LIU Li, TANG Fen, et al. Study on impact force of rockfall impact experiment on shed tunnel［J］. Journal of Disaster Prevention and Mitigation Engineering, 2018, 38（6）: 973 − 979. （in Chinese with English abstract）
[28] CALVETTI F,PRISCO C,VECCHIOTTI M. Experimental and numerical study of rock-fall impacts on granular soils Rivista Italiana di Geotecnica[J]. Rivista Italiana di Geotecnica,2005,4:95 − 109.
[29] KAWAHARA S,MURO T. Effects of dry density and thickness of sandy soil on impact response due to rockfall[J]. Journal of Terramechanics,2006,43(3):329 − 340. DOI: 10.1016/j.jterra.2005.05.009
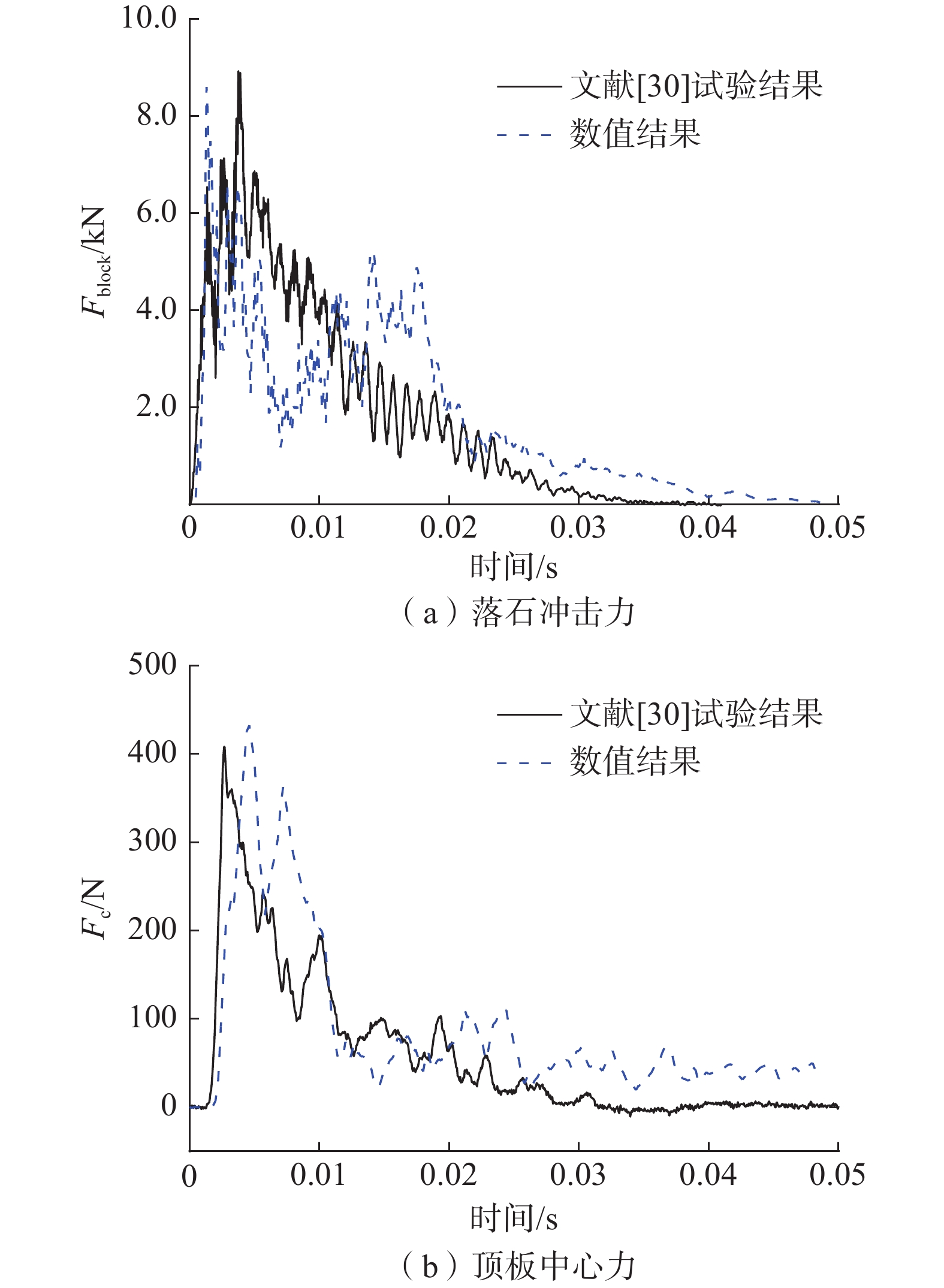
[30] 王林峰,姚昌银,邹政,等. 基于离散元方法的落石冲击力变化规律研究[J]. 铁道建筑,2017,57(6):101 − 105. [WANG Linfeng,YAO Changyin,ZOU Zheng,et al. Study on change law of rockfall impact force based on discrete element method[J]. Railway Engineering,2017,57(6):101 − 105. (in Chinese with English abstract)] WANG Linfeng, YAO Changyin, ZOU Zheng, et al. Study on change law of rockfall impact force based on discrete element method［J］. Railway Engineering, 2017, 57（6）: 101 − 105. （in Chinese with English abstract）
[31] 江巍,宋鹏程,陈玮,等. 基于PFC2D的土体缓冲落石冲击能力研究[J]. 长江科学院院报,2019,36(4):49 − 54. [JIANG Wei,SONG Pengcheng,CHEN Wei,et al. Cushioning capacity of soils against rockfall’s impact force based on two-dimensional particle flow code[J]. Journal of Yangtze River Scientific Research Institute,2019,36(4):49 − 54. (in Chinese with English abstract)] JIANG Wei, SONG Pengcheng, CHEN Wei, et al. Cushioning capacity of soils against rockfall’s impact force based on two-dimensional particle flow code［J］. Journal of Yangtze River Scientific Research Institute, 2019, 36（4）: 49 − 54. （in Chinese with English abstract）
[32] ZHANG Lingran,LAMBERT S,NICOT F. Discrete dynamic modelling of the mechanical behaviour of a granular soil[J]. International Journal of Impact Engineering,2017,103:76 − 89. DOI: 10.1016/j.ijimpeng.2017.01.009
[33] WANG Yucang,MORA P. The ESyS_Particle:A New 3-D Discrete Element Model with Single Particle Rotation[M]//Advances in Geocomputing. Berlin,Heidelberg:Springer,2009:183 − 228.
[34] SHEN Weigang,ZHAO Tao,DAI Feng. Influence of particle size on the buffering efficiency of soil cushion layer against rockfall impact[J]. Natural Hazards,2021,108(2):1469 − 1488. DOI: 10.1007/s11069-021-04741-6
-
期刊类型引用(1)
1. 管少杰，吕进国，王康，张砚力. 露天矿下伏采空区距坡脚水平距离对边坡稳定性的影响. 工矿自动化. 2025(02): 113-120 . 
百度学术
其他类型引用(0)
计量
- 文章访问数: 254
- HTML全文浏览量: 58
- PDF下载量: 93
- 被引次数: 1
邮件订阅
RSS