Geological hazard risk assessment and suggestions for risk control in Chaya County, eastern Xizang
-
摘要:
以藏东察雅县城为研究区,选取高程、坡度、坡形、坡向、斜坡结构、地层、距断层距离共7个评价指标,运用证据权重法,构建了地质灾害易发性评价模型。以4种降雨频率(10%、5%、2%、1%)下的年最大日降雨量作为动态诱发因子,建筑、人口和交通设施作为承灾体,评价地质灾害的动态风险性。结果表明,研究区斜坡以中、低风险为主,围绕县城场镇两侧的斜坡出现少量高风险和极高风险区。随着降雨频率的降低,区内高风险区、极高风险区面积最大增长5.34%和0.07%;低风险区、中风险区面积最大变化幅度分别为28.33%和23.32%。基于风险评价结果,提出考虑不同降雨频率的地质灾害风险源头管控方法,具体为:针对10%、5%、2%和1% 不同降雨频率下的极高风险区,建议分别采取工程治理、工程治理/专业监测、专业监测、专业监测/群专结合的管控手段;针对1%降雨频率下的高风险与中风险区,建议采取群专结合与群测群防。该风险管控体系考虑了不同降雨频率下斜坡的动态风险,可提高山区城镇地质灾害风险的管控精细化水平。
Abstract:Chaya County in eastern Xizang was selected as the research area for the susceptibility assessment of geological disasters. Seven evaluation indexes, including elevation, slope grade, slope form, slope direction, slope structure, stratum, and distance from fault, were selected to construct an evaluation model of geological disaster susceptibility using the evidence weight method. Using the annual maximum daily rainfall under four rainfall frequencies (10%, 5%, 2%, 1%) as the dynamic inducing factor and building population and transportation facilities as the hazard bearing body, the dynamic risk of geological hazards in the town was evaluated. The results show that except for the small slopes on both sides of the county town, which were mainly high-risk and extremely high-risk areas, other areas in the research area were mainly medium and low-risk areas. As the frequency of rainfall decreased, the areas of high-risk and extremely high-risk areas increased by a maximum of 5.34% and 0.07%, respectively, while the areas of low-risk and medium-risk areas changed by a maximum of 28.33% and 23.32%, respectively. Based on the risk assessment results, a method for controlling the source of geological hazard risk considering different rainfall frequencies was proposed. Specifically, for the extremely high-risk areas under the four rainfall frequencies of 10%, 5%, 2% and 1%, it is recommended to adopt engineering management, engineering management/professional monitoring, professional monitoring, and professional monitoring/combination of mass monitoring and professional monitoring. For the high-risk and medium-risk areas under a 1% rainfall frequency, the recommended risk control measures were the combination of mass monitoring and professional monitoring, and the combination of mass supervision and mass prevention.The risk management and control system accounted for the dynamic risks of slopes under different rainfall frequencies, which would enhance the management and control of geological hazard risks in mountainous urban areas in a refined manner.
-
Keywords:
- susceptibility /
- probability /
- risk assessment /
- risk control /
- Chaya County
-
0. 引言
地质灾害风险评价是对风险区遭受地质灾害的可能性和后果进行定量分析与评价,以及采取相应措施来降低风险可能性的一门交叉学科[1],主要包含易发性、危险性和易损性3个层次的评价内容。目前,国内外学者基于GIS对地质灾害风险评价进行了大量探索性研究。区域尺度上,Corominas等[2]系统总结了区域滑坡风险评价的方法;吴树仁等[3]于2009年初步提出了定性与定量评价相结合的地质灾害风险评估技术方法,首次编写完成了中国地质灾害风险评价指南,奠定了国内地质灾害风险评估与管理的研究基础;城镇地质灾害风险评价尺度上,康婧等[4]采用模糊数学法完成了长兴岛的灾害风险综合评价;李天华等[5]采用层次分析法与GIS空间分析结合法,建立了次生地质灾害风险评估模型;罗路广等[6]采用信息量模型和层次分析法评价“8•8”九寨沟景区地震地质灾害风险;张茂省等[7]在调查与评价的基础上,提出了适用于山区城镇地质灾害调查与风险评价的思路与技术方法;王佳佳[8]、吴芳[9]、肖婷[10]以三峡库区为研究区,对万州区滑坡灾害风险进行了评价和探索。总体上,地质灾害易发性、危险性评价是风险评价的基础,常用的方法一般有定性分析、概率统计法和确定性分析3种。对城镇评价尺度而言,半定量的概率统计方法使用较多[12 − 14],采用的模型包括信息量模型、逻辑回归模型、证据权模型、确定性系数模型以及基于机器学习的人工神经网络模型、决策树模型等。整体而言,城镇尺度上已初步形成相对完整的地质灾害风险评价方法,以往研究多集中在震区或中低山丘陵区,针对高原、极高山区城镇的风险评价研究较少,且考虑不同降雨频率下斜坡动态风险评价及源头管控方法研究仍整体处于探索阶段。
西藏昌都市地处青藏高原东缘,地形地貌差异较大,地质构造复杂,是西藏自治区地质灾害最易发的地区之一[15]。察雅县城位于昌都市中部麦曲河右岸,主要受到滑坡、崩塌和泥石流灾害的威胁。基于野外详细调查与编录,形成区内较完整的环境背景资料和灾害数据集。结合评价尺度和灾害特点,选用半定量的证据权法对研究区斜坡灾害开展易发性评价,并据此考虑在不同降雨工况下斜坡的危险性。参考中国地质调查局风险评价相关技术要求,以建筑、人口和交通设施作为承灾体,开展不同降雨频率的地质灾害风险评价,并提出斜坡动态风险的源头管控对策建议。该对策建议可提高山区城镇地质灾害风险管控的精细化水平,以达到经济合理、精准管控的目的。
1. 地质灾害风险评价方法
1.1 基于证据权法的地质灾害易发性评价方法
证据权法的基础为贝叶斯概率统计,它最早应用于矿产资源储量评价中。近年来,陆续应用在滑坡评价研究中[16 − 18],且被验证是相对较合适的评价方法。该方法基于滑坡发生区与非滑坡发生区来计算每个滑坡影响因子的权重,其核心假设是相同区域条件下,未来滑坡发生在类似滑坡已发生的区域内。采用式(1)—(3)来计算地质灾害的证据权:
$$ {{W}}_{{i}}^{+}={\ln}\frac{{P}\left\{{F|L}\right\}}{{P}\left\{{F|}\overline{{L}}\right\}} $$ (1) $$ {{W}}_{{i}}^{-}={\ln}\frac{{P}\left\{\overline{{F}}{|L}\right\}}{{P}\left\{\overline{{F}}{|}\overline{{L}}\right\}} $$ (2) $$ {{W}}_{{{\rm{f}}}}={{W}}_{{i}}^{+}-{{W}}_{{i}}^{-} $$ (3) 式中:
$ {W}_{i}^{+} $ ——该影响因子级别内部发生地质灾害的正相关关系大小;$ {W}_{i}^{-} $ ——该影响因子级别内部发生地质灾害的负相 关关系大小;P{F|L}/P{F|
$ \overline{L} $ }——地质灾害发生的充分率;P{
$ \overline{F} $ |L}/P{$ \overline{F} $ |$ \overline{L} $ }——地质灾害发生的必要率;${W}_{{\rm{f}}}$ ——该影响级别内发生地质灾害的权重。1.2 考虑不同降雨频率的地质灾害危险性评价方法
地质灾害危险性评价是在地质灾害易发性评价的基础上,进一步考虑地质灾害的诱发因素,来刻画和预测地质灾害发生的可能性大小。结合野外调查情况,本文考虑以降雨为主的诱发因子,采用年最大日降雨量作为时间动态因素,对研究区地质灾害开展危险性评价,计算公式为:
$$ {H=S}\cdot ({{H}}_{{24{{P}}}}{/}{\overline{{H}}}_{{24}} ) $$ (4) 式中:H——地质灾害危险性;
S——地质灾害易发性;
H24P——P降雨频率下的年最大日降雨量;
$ {\overline{{H}}}_{\text{24}} $ ——年最大日降雨量均值。1.3 地质灾害承灾体易损性评价方法
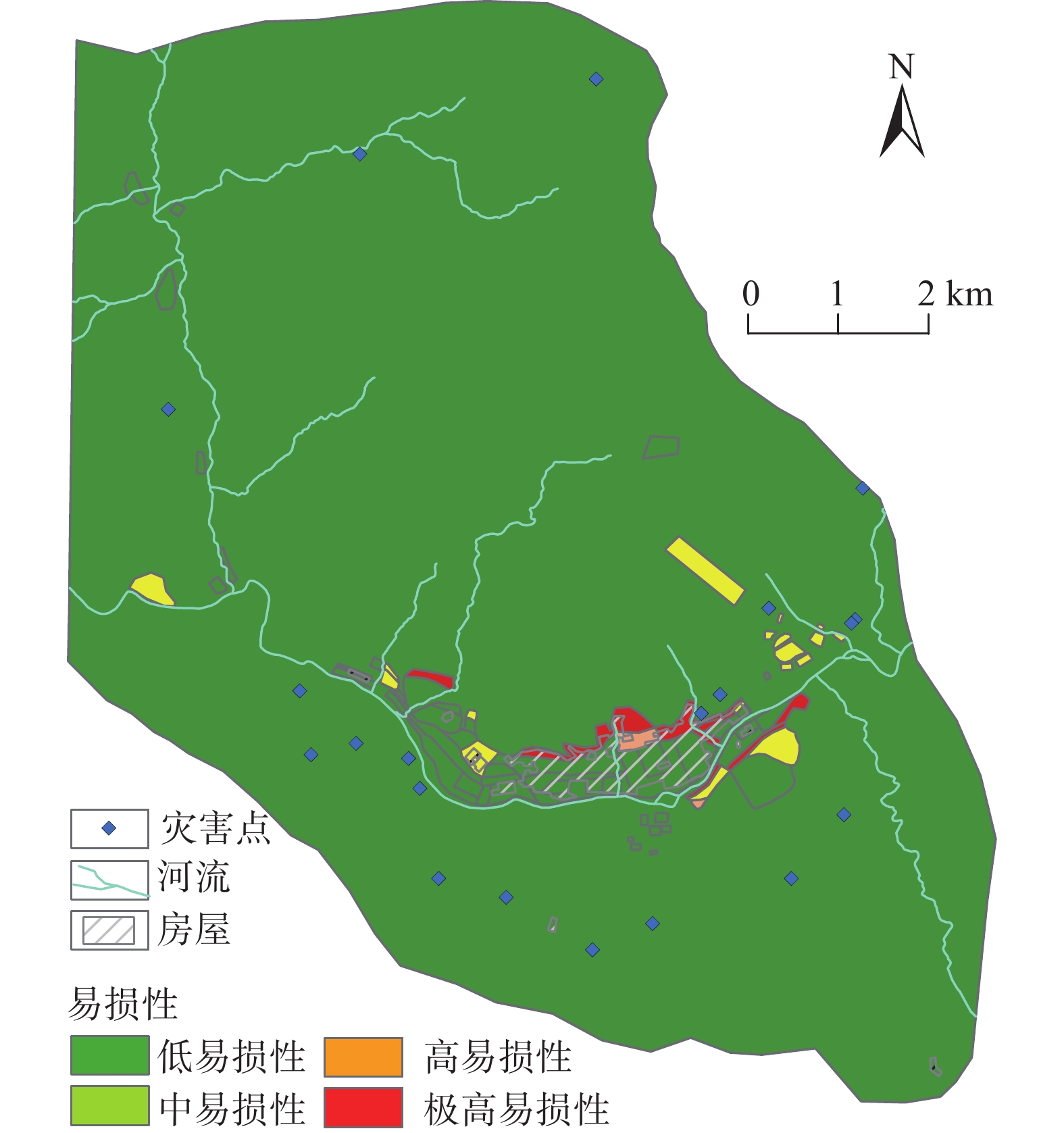
承灾体是指遭受地质灾害危害的对象,如人类、财产、资源或生态环境[19]。易损性是指承灾体受到一定强度的地质灾害作用时可能的损失程度。通常用0~1来定量表达,0表示无损失,1表示完全损失。结合察雅县城周边生态环境情况,将建筑、人口、交通设施作为承灾体,主要考虑威胁人口和造成经济损失2方面因素。同时,为方便研究,将常住人数和经济价值类似的建筑类型合并成同一类型,其要素与赋值关系详见表1。
表 1 研究区承灾体易损性赋值表Table 1. Vulnerability assessment table for hazard-bearing bodies in the study area承灾体类型 分类 易损性 对应属性字段 对应属性 建筑及
人口类型>1000人 0.9 类型 密集多层居住区 0.9 类型 医院 0.9 类型 学校 100~1000人 0.8 类型 密集低矮居住区 0.7 类型 寺庙 0.6 类型 加油站 0.8 类型 行政办公区 10~100人 0.4 类型 基础设施区 0.2 类型 一般居住区 0.3 类型 商业设施区 <10人 0.1 类型 临时居住区 0.1 类型 农业区 0.1 类型 荒地区 0.1 类型 避难场地区 交通设施 县级公路 0.3 GB 420301 专用公路 0.2 GB 420400 其他公路 0.1 GB 420800 城市道路 0.2~0.3 GB 430501、430501 乡村道路 0~0.1 GB 440100、440300 1.4 地质灾害风险评价方法
地质灾害风险是指在一定区域和给定时段内,地质灾害对人类生命财产和经济活动产生损失的可能性或期望值。地质灾害风险的计算公式为:
$$ {R=f(H,V)=H\cdot V} $$ (5) 式中:R——地质灾害风险性;
H——地质灾害危险性;
$V $ ——地质灾害易损性。地质灾害风险性评价采用矩阵分析方法[20],等级划分矩阵见图1。
2. 研究区地质环境条件
2.1 工程地质概况
察雅县城位于西藏自治区昌都市察雅县中西部,面积为81.71 km2。大地构造位于青藏高原东部、三江地区北段,属构造—侵蚀、侵蚀高山地貌区。为高原温带半干旱季风气候,多年平均降雨量为488 mm/a,集中在每年3—10月。
研究区地处澜沧江断裂带内,褶皱和断裂构造发育。察雅断层从县城处通过(NW—SE),下盘发育多个向、背斜;出露的地层岩性以侏罗系细粒碎屑岩为主。区内河流沟谷发育,麦曲由北向南贯穿整个察雅县城,与其他支流形成地表径流网。近年来,人类工程活动强烈,主要包括道路修建、城镇化建设等。
2.2 地质灾害
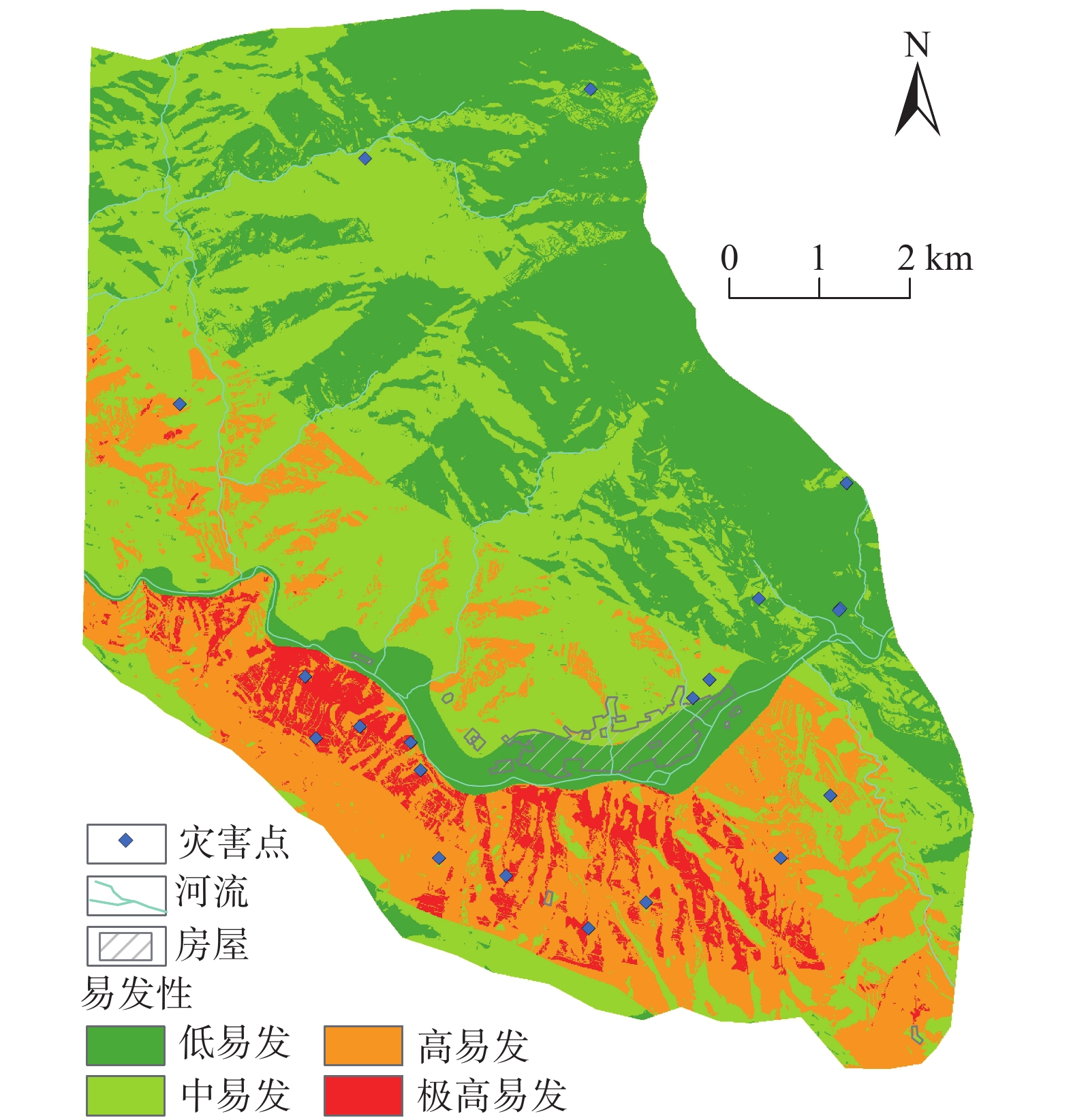
主要采用精度为1∶10000~1∶2000的工程地质调查方法,结合无人机测量和野外实地调查,研究区内共有地质灾害26处(图2),其中滑坡16处、崩塌2处、泥石流8处。研究区地质灾害主要以滑坡为主,普遍为历史老(古)滑坡,规模多为大型—特大型。本文仅讨论研究区内斜坡地质灾害风险评价。
3. 地质灾害风险评价
3.1 县城地质灾害易发性评价及精度检验
3.1.1 易发性评价
选取高程、坡度、坡形、坡向、斜坡结构、地层、距断层距离作为研究区地质灾害易发性评价指标,并对其进行相关性检验[21 − 23]。将分级之后的各因子矩阵导入SPSS中,采用Pearson相关性分析方法,得到如表2的各指标因子相关性统计表。当相关性系数R≤0.5时即可以认为各因子之间弱相关或不相关[11],验证了本次指标因子的选择具有合理性。
表 2 各指标因子间的相关性统计Table 2. Statistical table for correlation among each index factor高程 坡度 坡形 坡向 斜坡结构 地层 距断层
距离高程 1 坡度 0.020 1 坡形 0.032 −0.001 1 坡向 0.069 0.168 0.026 1 斜坡结构 0.134 0.128 0.021 0.258 1 地层 −0.316 −0.148 0.079 −0.030 −0.110 1 距断层距离 0.343 0.080 0.023 0.114 0.016 −0.172 1 所有的影响因子专题图均以10 m×10 m大小的栅格存储,利用ArcGIS空间分析功能计算各因子内各级别的
$ {{W}}_{{i}}^{{+}}、{{W}}_{{i}}^{-} $ 、$ W_{\rm{f}}$ ,详细结果见表3。对以上各因子进行叠加分析,得到研究区斜坡地质灾害易发性评价图(图3),易发指数范围为−22.0209~6.5123,分4级:低易发性(−22.0209~−5.9976)、中易发性(−5.9976~−1.6241)、高易发性(−1.6241~4.5929)、极高易发性(4.5929~6.5123)。表 3 研究区各证据因子权重值表Table 3. Summary table of weighted values of each featured factor in the study area影响因子及分级 ${ {W} }_{ {i} }^{ {+} } $ ${ {W} }_{ {i} }^{-}$ $W_{\rm{f}}$ 高程/m 3000~3500 0.2483 −0.2112 0.4596 3500~4000 −0.1064 0.0935 −0.1999 4000~4500 −0.9514 0.0689 −1.0203 坡度/(°) <10 −0.7271 0.0691 −0.7962 10~20 0.2796 −0.0750 0.3546 20~30 0.1089 −0.0616 0.1705 30~40 0.0396 −0.0146 0.0542 40~50 −0.6449 0.0374 −0.6823 50~60 −1.1721 0.0095 −1.1816 >60 −4.3125 0.0014 −4.3139 坡向 平面 0.5463 −0.0792 0.6256 北 1.5770 −0.2022 1.7792 东北 1.0220 −0.2189 1.2409 东 −0.1133 0.0128 −0.1260 东南 −0.6638 0.0553 −0.7192 南 −2.3082 0.1450 −2.4532 西南 −3.3031 0.1624 −3.4655 西 −1.5441 0.1140 −1.6580 西北 0.2250 −0.0326 0.2575 坡型 凹形 −0.2290 0.0491 −0.2780 凸型 0.1337 −0.2348 0.3685 直线型 −0.2392 0.0546 −0.2938 斜坡结构 河谷 −7.4634 0.0526 −7.5160 顺向飘倾坡 −2.6380 0.0150 −2.6530 顺向层面坡 −0.7848 0.0088 −0.7936 顺向伏倾坡 −0.5073 0.0425 −0.5498 斜顺向坡 −0.5159 0.0577 −0.5737 横向坡 −0.2863 0.0976 −0.3839 斜逆向坡 0.3733 −0.1057 0.4790 逆向坡 0.5425 −0.2225 0.7649 地层 T3d 1.3006 −0.1359 1.4366 J2d −2.5677 0.2464 −2.8140 J2c 0.0000 0.0007 −0.0007 J1w 0.1942 −0.5731 0.7673 ${\rm{Q} }{\rm{h} }^{el}$ −3.7152 0.0512 −3.7664 距断层距离/m <200 0.5295 −0.0395 0.5690 200~500 0.8501 −0.1114 0.9614 500~1000 0.8722 −0.2073 1.0795 1000~2000 0.4909 −0.1912 0.6820 >2000 −1.6415 0.6672 −2.3087 3.1.2 易发性评价结果检验
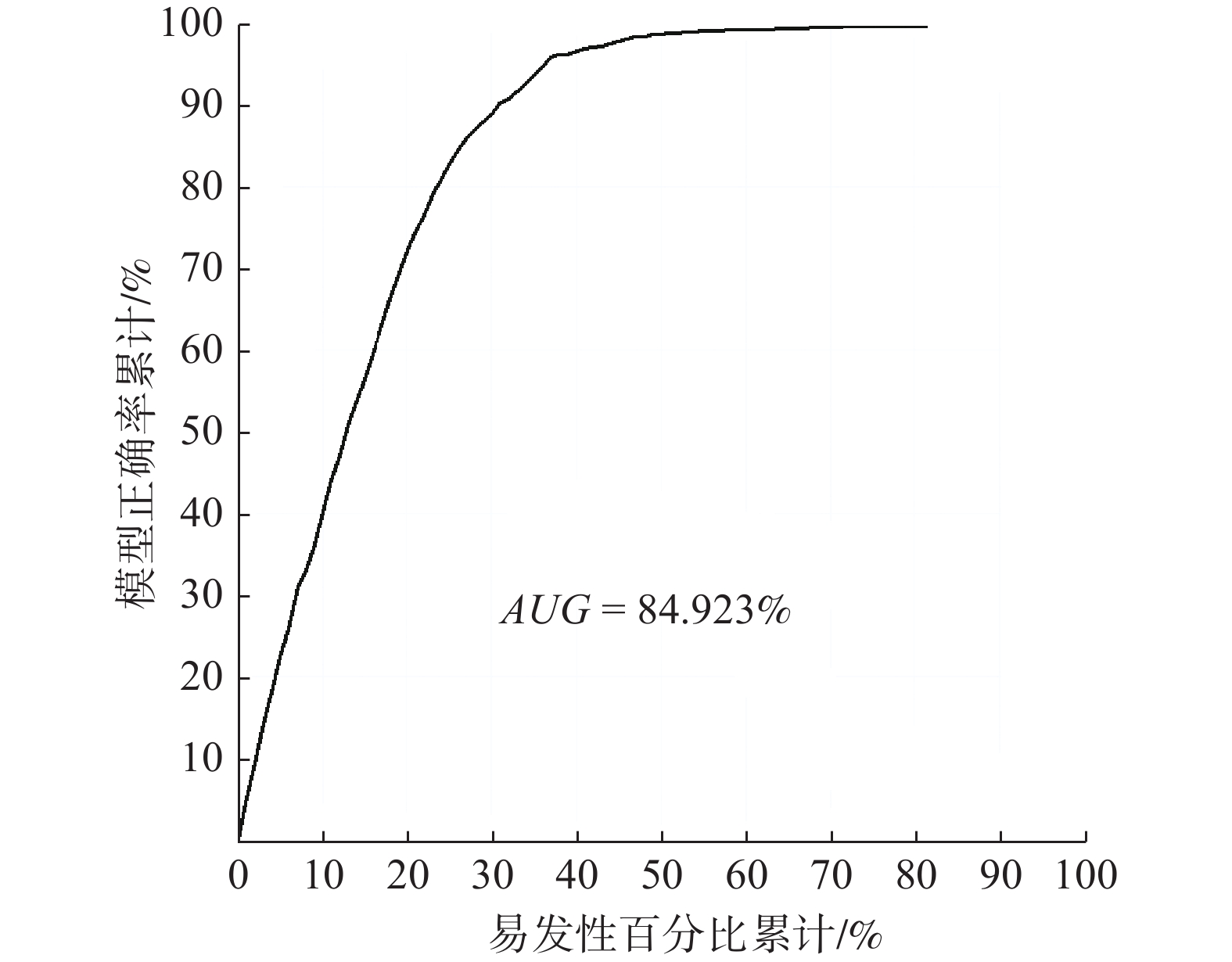
地质灾害易发性评价过程中,常采用成功率曲线(receiver operating characteristic curve,ROC)及曲线下的面积(area under curve,AUC)的值来评价结果的准确性[24 − 25]。将易发性评价结果从高到低按照1%的面积间隔等分为100等分,分别求取这100个级别内地质灾害发生的百分比,构建易发性成功率曲线,结果见图4。结果显示AUC值为84.923%,说明该易发性评价结果较为合理。
3.2 县城地质灾害危险性评价
由昌都市1980—2015年降雨数值,确定研究区年最大日降雨量均值
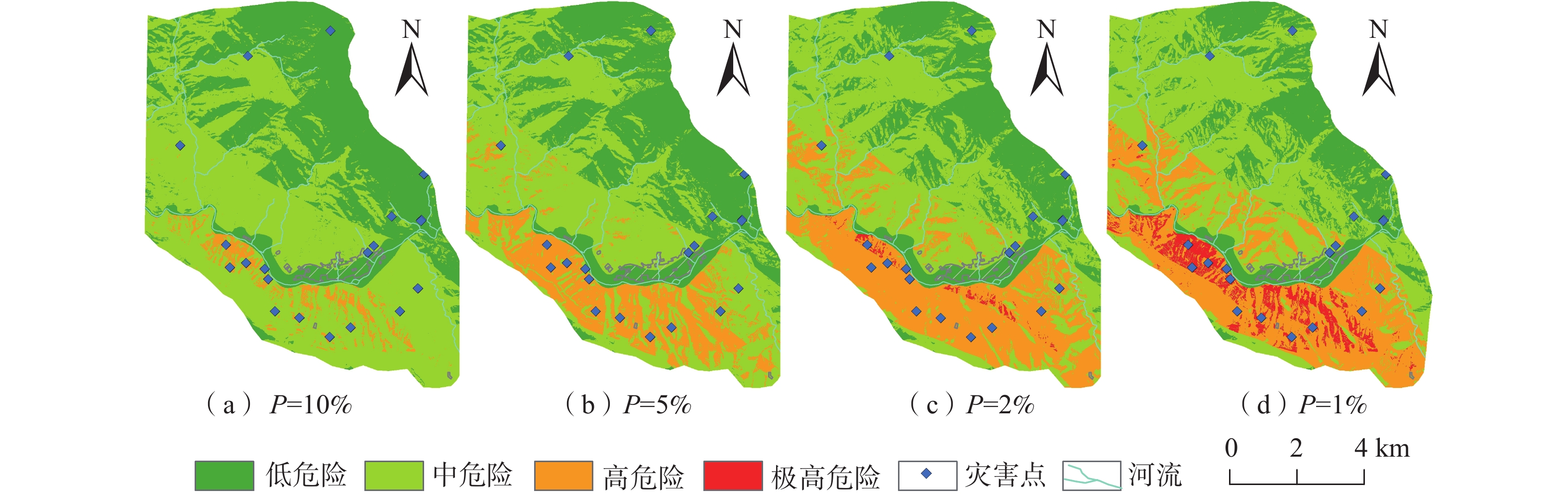
$ {\overline{{H}}}_{\text{24}} $ 取28.4 mm,变差系数Cv取0.16。选用皮尔逊Ⅲ型分布函数对研究区进行频率分析,通过其离均系数KP,计算不同降雨频率下的年最大日降雨量H24P,计算式见(8),计算结果见表4。表 4 研究区不同降雨频率下的年最大日降雨量估算结果Table 4. Estimation results of annual maximum daily rainfall under different rainfall frequencies in the study areaP/% KP H24P/mm 1 1.436 55.14 2 1.374 52.76 5 1.286 49.38 10 1.212 46.54 $$ H_{24P} = K_{P} × {\overline{{H}}}_{\text{24}} $$ (8) 将研究区地质灾害易发性与4种不同频率的年最大日降雨量叠加分析,得到各工况下察雅县城地质灾害危险性评价图(图5)。分为4级:低危险(0~20.5242)、中危险(20.5242~32.4620)、高危险(32.4620~38.1521)、极高危险(>38.1521)。
3.3 县城地质灾害承灾体易损性评价
对研究区内的不同承灾体开展遥感解译与野外调查,共统计县城内建筑人口包括密集多层居住区、医院、学校、寺庙、加油站、行政办公区等14类;共统计包括县级公路、专用公路、其他公路等5类道路。将各类建筑的用途、结构、人口,道路属性等作为承灾体参数,构建察雅察雅县城承灾体空间数据库。将不同类型承灾体易损性进行叠加,获得综合易损性评价图(图6),并划分为4个等级:低易损性(0~0.25)、中易损性(0.25~0.5)、高易损性(0.5~0.75)、极高易损性(0.75~1.0)。
3.4 县城地质灾害风险评价
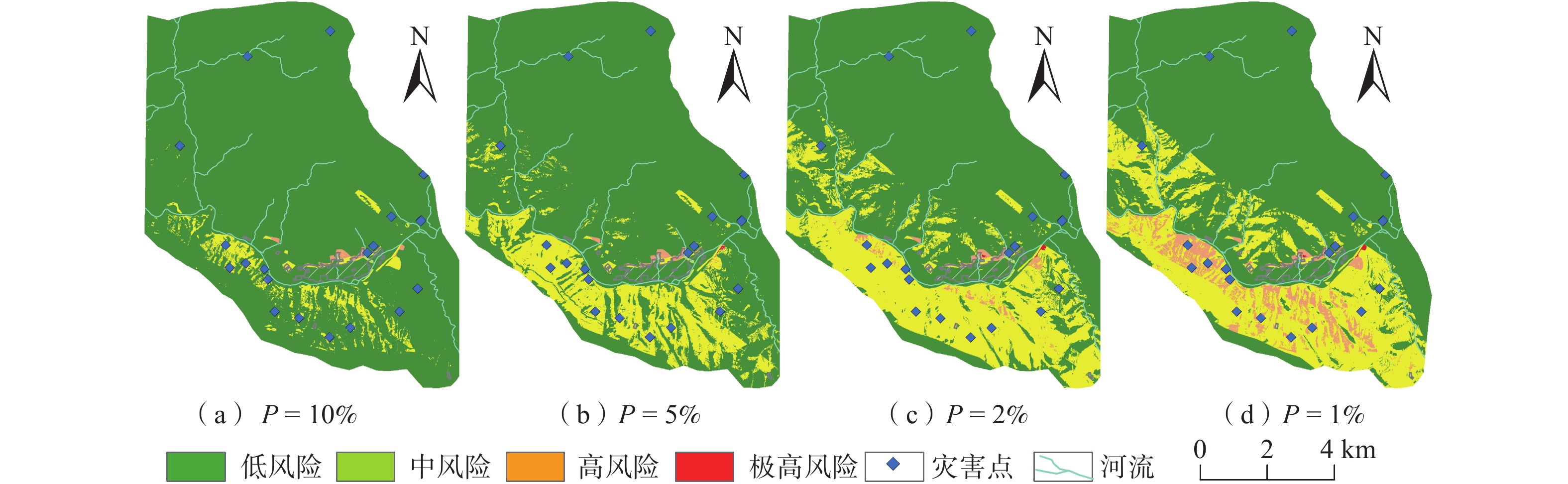
基于察雅县城地质灾害危险性和易损性评价结果,划分为低风险、中风险、高风险和极高风险4级(图7)。
4. 结果分析与风险管控建议
4.1 结果分析
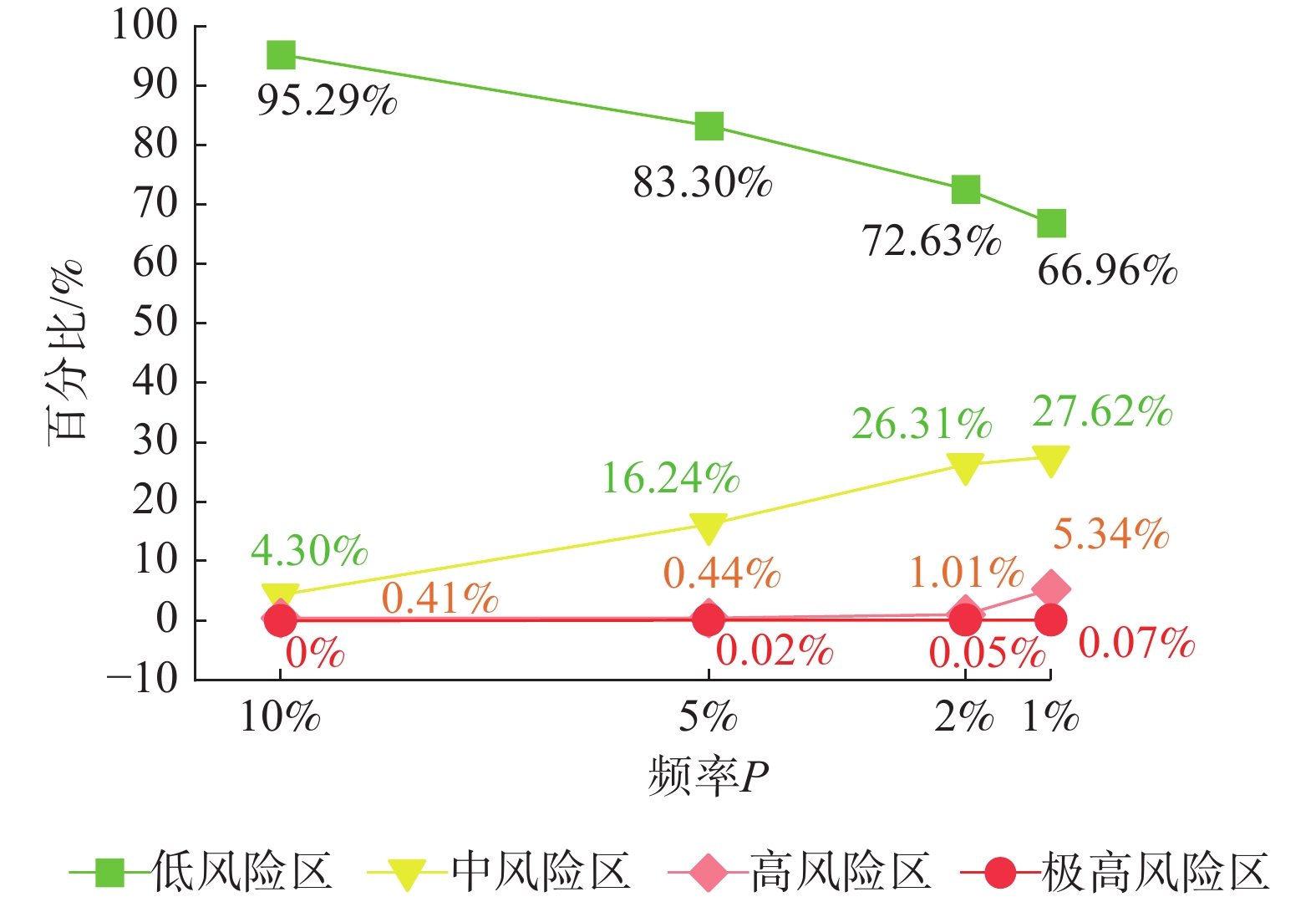
(1)察雅县城整体以低风险与中风险为主,围绕县城两侧斜坡发现少量高风险与极高风险区。随着降雨频率的降低,区内低风险区面积发生明显降低,最大下降幅度为28.33%;中风险区面积呈现明显增长趋势,最大增长幅度达23.32%。
(2)察雅县城有0.41%的区域始终保持高风险状态,如察雅县城北侧、察雅县城南东侧等地,亟需立即开展相关的管控措施。随着降雨频率的降低,高风险区面积比例最大增长至5.34%,极高风险区面积比例最大增长至0.07%,主要是因为这些区域建筑、人口财产等分布集中,且地质灾害易发程度也多为极高易发—高易发状态。
(3)随着降雨频率的降低,察雅县城城区两侧斜坡的风险等级也逐渐由中低风险为主过渡到以中高风险为主,至百年一遇降雨频率下,极高—高风险区占比为5.41%,其发生灾害后风险较大,可能形成淹没前缘房屋、堵塞麦曲等危害。
(4)察雅县城存在66.96%区域始终保持低风险状态,分布在研究区北侧远离县城建筑区域,主要是因为这些区域人迹罕至且工程建设程度低。
4.2 风险管控措施体系及建议
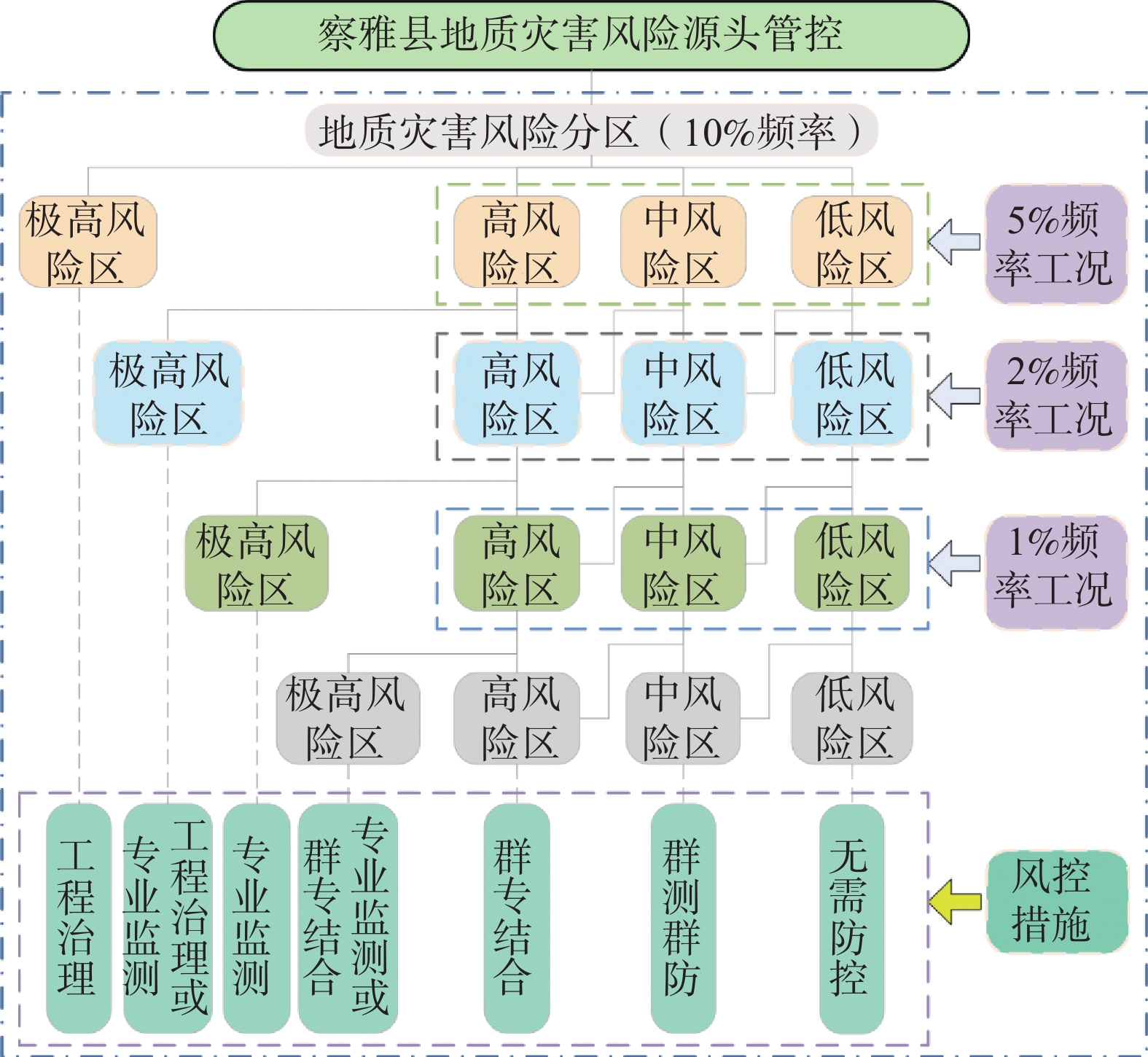
基于风险评价结果,提出考虑不同降雨频率的察雅县城地质灾害风险源头管控方法(图9)。其核心思想是优先判别出最受关注的极高风险区或随降雨频率改变情况下增加的极高风险区,非极高风险区再按照10%、5%、2%和1%的降雨频率逐级判别,依据不同降雨频率和风险级别大小分别采取针对的源头管控措施,以达到经济合理、精准管控的目的。管控措施主要为工程治理、专业监测、群专结合和群测群防4类。
(1)针对10%降雨频率下为极高风险的区域,由于在高频降雨条件下风险极高,建议规划设计相关防治工程,制定应急避险方案,降低地质灾害风险;
(2)针对5%降雨频率下由高风险转为极高风险的区域,如察雅县城北侧斜坡、县城东侧麦曲左岸等斜坡,由于是在中度降雨频率影响下即转化为极高风险区,建议视斜坡变形破坏程度和危害程度,适当选择工程治理或专业监测的管控措施,降低或管控地质灾害风险;
(3)针对2%工况下由高风险转化为极高风险的区域,如察雅县城北侧部分斜坡等,由于是受略低的降雨频次影响后才转化为极高风险区,建议按照斜坡灾害发育阶段和特征,适当选择合适的专业监测手段,管控地质灾害风险。
(4)针对1%工况下由高风险转化为极高风险的区域,如察雅县城北侧部分斜坡等,由于其是在低降雨频率影响后才转化为极高风险区,建议视斜坡发育特征,适当选取专业监测或群专结合手段来管控地质灾害风险。
(5)针对1%工况下高风险区,如察雅县城南侧斜坡等,存在下滑堵江的可能,建议根据10%、5%、2%降雨频率下是否为高风险区且判断是否可能产生二次灾害为依据,合理选择不同群专结合方案,管控地质灾害风险;针对1%工况下中风险区,由于风险等级中等,建议采取的主要风险管控措施为群测群防;针对1%工况下低风险区,由于其发生灾害后可能产生的损失小,建议短期内无需管控。
5. 结论
(1)运用证据权方法,选取与县城地质灾害形成有关的、相互独立的7个指标因子,构建了地质灾害易发性评价模型,采用成功率曲线(ROC)对评价结果进行检验,结果显示曲线下的面积(AUC)为84.923%,反映了评价结果较为合理。
(2)选取10%、5%、2%和1% 4种降雨频率下年最大日降雨量作为动态诱发因子,建筑人口和交通设施作为承灾体,完成了动态风险性评价。结果表明,察雅县城整体以低风险与中风险为主,围绕县城两侧斜坡发现少量高风险、极高风险区。随着降雨频率的降低,察雅县城低风险区面积发生明显降低,最大下降幅度为28.33%;随着降雨频率的降低,区内低风险区面积发生明显降低,最大下降幅度为28.33%;中风险区面积呈现明显增长趋势,最大增长幅度为23.32%;高风险区面积比例最大增长至5.34%,极高风险区面积比例最大增长至0.07%;此外,不论降雨频率如何变化,区内0.41%的区域始终保持高风险状态;66.96%区域始终保持低风险状态。
(3)基于风险评价结果,提出考虑不同降雨频率的察雅县城地质灾害风险源头管控方法。核心思想是优先判别出最受关注的极高风险区或随降雨频率改变情况下增加的极高风险区,非极高风险区再按照10%、5%、2%和1%的降雨频率逐级判别,依据不同降雨频率和风险级别大小分别采取针对的源头管控措施,以达到经济合理、精准管控的目的。管控措施主要为工程治理、专业监测、群专结合和群测群防4类。
-
表 1 研究区承灾体易损性赋值表
Table 1 Vulnerability assessment table for hazard-bearing bodies in the study area
承灾体类型 分类 易损性 对应属性字段 对应属性 建筑及
人口类型>1000人 0.9 类型 密集多层居住区 0.9 类型 医院 0.9 类型 学校 100~1000人 0.8 类型 密集低矮居住区 0.7 类型 寺庙 0.6 类型 加油站 0.8 类型 行政办公区 10~100人 0.4 类型 基础设施区 0.2 类型 一般居住区 0.3 类型 商业设施区 <10人 0.1 类型 临时居住区 0.1 类型 农业区 0.1 类型 荒地区 0.1 类型 避难场地区 交通设施 县级公路 0.3 GB 420301 专用公路 0.2 GB 420400 其他公路 0.1 GB 420800 城市道路 0.2~0.3 GB 430501、430501 乡村道路 0~0.1 GB 440100、440300 表 2 各指标因子间的相关性统计
Table 2 Statistical table for correlation among each index factor
高程 坡度 坡形 坡向 斜坡结构 地层 距断层
距离高程 1 坡度 0.020 1 坡形 0.032 −0.001 1 坡向 0.069 0.168 0.026 1 斜坡结构 0.134 0.128 0.021 0.258 1 地层 −0.316 −0.148 0.079 −0.030 −0.110 1 距断层距离 0.343 0.080 0.023 0.114 0.016 −0.172 1 表 3 研究区各证据因子权重值表
Table 3 Summary table of weighted values of each featured factor in the study area
影响因子及分级 ${ {W} }_{ {i} }^{ {+} } $ ${ {W} }_{ {i} }^{-}$ $W_{\rm{f}}$ 高程/m 3000~3500 0.2483 −0.2112 0.4596 3500~4000 −0.1064 0.0935 −0.1999 4000~4500 −0.9514 0.0689 −1.0203 坡度/(°) <10 −0.7271 0.0691 −0.7962 10~20 0.2796 −0.0750 0.3546 20~30 0.1089 −0.0616 0.1705 30~40 0.0396 −0.0146 0.0542 40~50 −0.6449 0.0374 −0.6823 50~60 −1.1721 0.0095 −1.1816 >60 −4.3125 0.0014 −4.3139 坡向 平面 0.5463 −0.0792 0.6256 北 1.5770 −0.2022 1.7792 东北 1.0220 −0.2189 1.2409 东 −0.1133 0.0128 −0.1260 东南 −0.6638 0.0553 −0.7192 南 −2.3082 0.1450 −2.4532 西南 −3.3031 0.1624 −3.4655 西 −1.5441 0.1140 −1.6580 西北 0.2250 −0.0326 0.2575 坡型 凹形 −0.2290 0.0491 −0.2780 凸型 0.1337 −0.2348 0.3685 直线型 −0.2392 0.0546 −0.2938 斜坡结构 河谷 −7.4634 0.0526 −7.5160 顺向飘倾坡 −2.6380 0.0150 −2.6530 顺向层面坡 −0.7848 0.0088 −0.7936 顺向伏倾坡 −0.5073 0.0425 −0.5498 斜顺向坡 −0.5159 0.0577 −0.5737 横向坡 −0.2863 0.0976 −0.3839 斜逆向坡 0.3733 −0.1057 0.4790 逆向坡 0.5425 −0.2225 0.7649 地层 T3d 1.3006 −0.1359 1.4366 J2d −2.5677 0.2464 −2.8140 J2c 0.0000 0.0007 −0.0007 J1w 0.1942 −0.5731 0.7673 ${\rm{Q} }{\rm{h} }^{el}$ −3.7152 0.0512 −3.7664 距断层距离/m <200 0.5295 −0.0395 0.5690 200~500 0.8501 −0.1114 0.9614 500~1000 0.8722 −0.2073 1.0795 1000~2000 0.4909 −0.1912 0.6820 >2000 −1.6415 0.6672 −2.3087 表 4 研究区不同降雨频率下的年最大日降雨量估算结果
Table 4 Estimation results of annual maximum daily rainfall under different rainfall frequencies in the study area
P/% KP H24P/mm 1 1.436 55.14 2 1.374 52.76 5 1.286 49.38 10 1.212 46.54 -
[1] 齐信,唐川,陈州丰,等. 地质灾害风险评价研究[J]. 自然灾害学报,2012,21(5):33 − 40. [QI Xin,TANG Chuan,CHEN Zhoufeng,et al. Research of geohazards risk assessment[J]. Journal of Natural Disasters,2012,21(5):33 − 40. (in Chinese with English abstract) DOI: 10.13577/j.jnd.2012.0506 QI Xin, TANG Chuan, CHEN Zhoufeng, et al. Research of geohazards risk assessment[J]. Journal of Natural Disasters, 2012, 21(5): 33-40. (in Chinese with English abstract) DOI: 10.13577/j.jnd.2012.0506
[2] COROMINAS J,VAN WESTEN C,FRATTINI P,et al. Recommendations for the quantitative analysis of landslide risk[J]. Bulletin of Engineering Geology and the Environment,2014,73(2):209 − 263.
[3] 吴树仁,石菊松,张春山,等. 地质灾害风险评估技术指南初论[J]. 地质通报,2009,28(8):995 − 1005. [WU Shuren,SHI Jusong,ZHANG Chunshan,et al. Preliminary discussion on technical guideline for geohazard risk assessment[J]. Geological Bulletin of China,2009,28(8):995 − 1005. (in Chinese with English abstract) DOI: 10.3969/j.issn.1671-2552.2009.08.001 WU Shuren, SHI Jusong, ZHANG Chunshan, et al. Preliminary discussion on technical guideline for geohazard risk assessment[J]. Geological Bulletin of China, 2009, 28(8): 995-1005. (in Chinese with English abstract) DOI: 10.3969/j.issn.1671-2552.2009.08.001
[4] 康婧,王伟伟,程林,等. 基于模糊数学方法的海岛地质灾害风险评价—以长兴岛为例[J]. 海洋环境科学,2016,35(6):861 − 867. [KANG Jing,WANG Weiwei,CHENG Lin,et al. Risk assessment of geological hazard based on fuzzy mathematics:A case study of Changxing Island[J]. Marine Environmental Science,2016,35(6):861 − 867. (in Chinese with English abstract) DOI: 10.13634/j.cnki.mes.2016.06.035 KANG Jing, WANG Weiwei, CHENG Lin, et al. Risk assessment of geological hazard based on fuzzy mathematics—a case study of Changxing Island[J]. Marine Environmental Science, 2016, 35(6): 861-867. (in Chinese with English abstract) DOI: 10.13634/j.cnki.mes.2016.06.035
[5] 李天华,袁永博. 地震重灾区诱发次生地质灾害风险评价研究[J]. 地震工程学报,2018,40(1):111 − 115. [LI Tianhua,YUAN Yongbo. Risk assessment of secondary geological disasters induced in an earthquake-stricken area[J]. China Earthquake Engineering Journal,2018,40(1):111 − 115. (in Chinese with English abstract) DOI: 10.3969/j.issn.1000-0844.2018.01.111 LI Tianhua, YUAN Yongbo. Risk assessment of secondary geological disasters induced in an earthquake-stricken area[J]. China Earthquake Engineering Journal, 2018, 40(1): 111-115. (in Chinese with English abstract) DOI: 10.3969/j.issn.1000-0844.2018.01.111
[6] 罗路广,裴向军,谷虎,等. 基于GIS的“8•8”九寨沟地震景区地质灾害风险评价[J]. 自然灾害学报,2020,29(3):193 − 202. [LUO Luguang,PEI Xiangjun,GU Hu,et al. Risk assessment of geohazards induced by “8•8” earthquake based on GIS in Jiuzhaigou scenic area[J]. Journal of Natural Disasters,2020,29(3):193 − 202. (in Chinese with English abstract) DOI: 10.13577/j.jnd.2020.0321 LUO Luguang, PEI Xiangjun, GU Hu, et al. Risk assessment of geohazards induced by “8.8” earthquake based on GIS in Jiuzhaigou scenic area[J]. Journal of Natural Disasters, 2020, 29(3): 193-202. (in Chinese with English abstract) DOI: 10.13577/j.jnd.2020.0321
[7] 张茂省,薛强,贾俊,等. 山区城镇地质灾害调查与风险评价方法及实践[J]. 西北地质,2019,52(2):125 − 135. [ZHANG Maosheng,XUE Qiang,JIA Jun,et al. Methods and practices for the investigation and risk assessment of geo-hazards in mountainous towns[J]. Northwestern Geology,2019,52(2):125 − 135. (in Chinese with English abstract) DOI: 10.19751/j.cnki.61-1149/p.2019.02.013 ZHANG Maosheng, XUE Qiang, JIA Jun, et al. Methods and practices for the investigation and risk assessment of geo-hazards in mountainous towns[J]. Northwestern Geology, 2019, 52(2): 125-135. (in Chinese with English abstract) DOI: 10.19751/j.cnki.61-1149/p.2019.02.013
[8] 王佳佳. 三峡库区万州区滑坡灾害风险评估研究[D]. 武汉: 中国地质大学, 2015 WANG Jiajia. Landslide risk assessment in Wanzhou County, Three Gorges Reservoir[D]. Wuhan: China University of Geosciences, 2015. (in Chinese with English abstract)
[9] 王芳. 万州区滑坡灾害风险评价与管理研究[D]. 武汉: 中国地质大学, 2017 WANG Fang. Study on risk assessment and management of landslide in Wanzhou District[D]. Wuhan: China University of Geosciences, 2017. (in Chinese with English abstract)
[10] 肖婷. 三峡库区万州区及重点库岸段滑坡灾害风险评价[D]. 武汉: 中国地质大学, 2020 XIAO Ting. Landslide risk assessment in Wanzhou district and a key section, Three Gorges Reservoir[D]. Wuhan: China University of Geosciences, 2020. (in Chinese with English abstract)
[11] 周超,常鸣,徐璐,等. 贵州省典型城镇矿山地质灾害风险评价[J]. 武汉大学学报(信息科学版),2020,45(11):1782 − 1791. [ZHOU Chao,CHANG Ming,XU Lu,et al. Risk assessment of typical urban mine geological disasters in Guizhou Province[J]. Geomatics and Information Science of Wuhan University,2020,45(11):1782 − 1791. (in Chinese with English abstract) ZHOU Chao, CHANG Ming, XU Lu, et al. Risk assessment of typical urban mine geological disasters in Guizhou Province[J]. Geomatics and Information Science of Wuhan University, 2020, 45(11): 1782-1791. (in Chinese with English abstract)
[12] 熊小辉,汪长林,白永健,等. 基于不同耦合模型的县域滑坡易发性评价对比分析—以四川普格县为例[J]. 中国地质灾害与防治学报,2022,33(4):114 − 124. [XIONG Xiaohui,WANG Changlin,BAI Yongjian,et al. Comparison of landslide susceptibility assessment based on multiple hybrid models at County level:A case study for Puge County,Sichuan Province[J]. The Chinese Journal of Geological Hazard and Control,2022,33(4):114 − 124. (in Chinese with English abstract) XIONG Xiaohui, WANG Changlin, BAI Yongjian, et al. Comparison of landslide susceptibility assessment based on multiple hybrid models at County level: a case study for Puge County, Sichuan Province[J]. The Chinese Journal of Geological Hazard and Control, 2022, 33(4): 114-124. (in Chinese with English abstract)
[13] 解明礼,巨能攀,刘蕴琨,等. 崩塌滑坡地质灾害风险排序方法研究[J]. 水文地质工程地质,2021,48(5):184 − 192. [XIE Mingli,JU Nengpan,LIU Yunkun,et al. A study of the risk ranking method of landslides and collapses[J]. Hydrogeology & Engineering Geology,2021,48(5):184 − 192. (in Chinese with English abstract) DOI: 10.16030/j.cnki.issn.1000-3665.202007011 XIE Mingli, JU Nengpan, LIU Yunkun, et al. A study of the risk ranking method of landslides and collapses[J]. Hydrogeology & Engineering Geology, 2021, 48(5): 184-192. (in Chinese with English abstract) DOI: 10.16030/j.cnki.issn.1000-3665.202007011
[14] 范强,巨能攀,解明礼,等. 2017年九寨沟MS7.0地震前后地质灾害风险对比[J]. 地震研究,2019,42(3):419 − 427. [FAN Qiang,JU Nengpan,XIE Mingli,et al. Comparation of geological hazard risks before and after Jiuzhaigou MS7.0 earthquake in 2017[J]. Journal of Seismological Research,2019,42(3):419 − 427. (in Chinese with English abstract) DOI: 10.3969/j.issn.1000-0666.2019.03.016 FAN Qiang, JU Nengpan, XIE Mingli, et al. Comparation of geological hazard risks before and after Jiuzhaigou MS7.0 earthquake in 2017[J]. Journal of Seismological Research, 2019, 42(3): 419-427. (in Chinese with English abstract) DOI: 10.3969/j.issn.1000-0666.2019.03.016
[15] 关朝阳,李章国. 西藏昌都地质灾害特点及防治对策[J]. 中国地质灾害与防治学报,2018,29(2):104 − 107. [GUAN Chaoyang,LI Zhangguo. Characteristics and prevention measures of geological hazards in Changdu City,Tibet[J]. The Chinese Journal of Geological Hazard and Control,2018,29(2):104 − 107. (in Chinese with English abstract) DOI: 10.16031/j.cnki.issn.1003-8035.2018.02.17 GUAN Chaoyang, LI Zhangguo. Characteristics and prevention measures of geological hazards in Changdu City, Tibet[J]. The Chinese Journal of Geological Hazard and Control, 2018, 29(2): 104-107. (in Chinese with English abstract) DOI: 10.16031/j.cnki.issn.1003-8035.2018.02.17
[16] 范强,巨能攀,向喜琼,等. 证据权法在区域滑坡危险性评价中的应用—以贵州省为例[J]. 工程地质学报,2014,22(3):474 − 481. [FAN Qiang,JU Nengpan,XIANG Xiqiong,et al. Landslides hazards assessment with weights of evidence:A case study in Guizhou,China[J]. Journal of Engineering Geology,2014,22(3):474 − 481. (in Chinese with English abstract) DOI: 10.13544/j.cnki.jeg.2014.03.017 FAN Qiang, JU Nengpan, XIANG Xiqiong, et al. Landslides hazards assessment with weights of evidence—a case study in Guizhou, China[J]. Journal of Engineering Geology, 2014, 22(3): 474-481. (in Chinese with English abstract) DOI: 10.13544/j.cnki.jeg.2014.03.017
[17] 郭长宝,唐杰,吴瑞安,等. 基于证据权模型的川藏铁路加查—朗县段滑坡易发性评价[J]. 山地学报,2019,37(2):240 − 251. [GUO Changbao,TANG Jie,WU Ruian,et al. Landslide susceptibility assessment based on WOE model along Jiacha—Langxian County section of Sichuan—Tibet railway,China[J]. Mountain Research,2019,37(2):240 − 251. (in Chinese with English abstract) DOI: 10.16089/j.cnki.1008-2786.000418 GUO Changbao, TANG Jie, WU Ruian, et al. Landslide susceptibility assessment based on WOE model along Jiacha—Langxian County section of sichuan—tibet railway, China[J]. Mountain Research, 2019, 37(2): 240-251. (in Chinese with English abstract) DOI: 10.16089/j.cnki.1008-2786.000418
[18] 胡燕,李德营,孟颂颂,等. 基于证据权法的巴东县城滑坡灾害易发性评价[J]. 地质科技通报,2020,39(3):187 − 194. [HU Yan,LI Deying,MENG Songsong,et al. Landslide susceptibility evaluation in Badong County based on weights of evidence method[J]. Bulletin of Geological Science and Technology,2020,39(3):187 − 194. (in Chinese with English abstract) HU Yan, LI Deying, MENG Songsong, et al. Landslide susceptibility evaluation in Badong County based on weights of evidence method[J]. Bulletin of Geological Science and Technology, 2020, 39(3): 187-194. (in Chinese with English abstract)
[19] 刘传正. 崩塌滑坡灾害风险识别方法初步研究[J]. 工程地质学报,2019,27(1):88 − 97. [LIU Chuanzheng. Analysis methods on the risk identification of landslide disasters[J]. Journal of Engineering Geology,2019,27(1):88 − 97. (in Chinese with English abstract) DOI: 10.13544/j.cnki.jeg.2019-009 LIU Chuanzheng. Analysis methods on the risk identification of landslide disasters[J]. Journal of Engineering Geology, 2019, 27(1): 88-97. (in Chinese with English abstract) DOI: 10.13544/j.cnki.jeg.2019-009
[20] 中国地质调查局. 地质灾害风险调查评价技术要求(1∶50000)[S]. 2020 China Geological Survey. Technical guide for geohazard risk survey and evaluation (1∶50000)[S]. 2020.(in Chinese)
[21] 张俊,殷坤龙,王佳佳,等. 三峡库区万州区滑坡灾害易发性评价研究[J]. 岩石力学与工程学报,2016,35(2):284 − 296. [ZHANG Jun,YIN Kunlong,WANG Jiajia,et al. Evaluation of landslide susceptibility for Wanzhou District of Three Gorges Reservoir[J]. Chinese Journal of Rock Mechanics and Engineering,2016,35(2):284 − 296. (in Chinese with English abstract) DOI: 10.13722/j.cnki.jrme.2015.0318 ZHANG Jun, YIN Kunlong, WANG Jiajia, et al. Evaluation of landslide susceptibility for Wanzhou district of Three Gorges Reservoir[J]. Chinese Journal of Rock Mechanics and Engineering, 2016, 35(2): 284-296. (in Chinese with English abstract) DOI: 10.13722/j.cnki.jrme.2015.0318
[22] 汤明高, 陈浩文, 赵欢乐, 等. 青藏高原冰湖溃决灾害隐患识别、发育规律及危险性评价[J]. 地质通报,2023,42(5):730 − 742. [TANG Minggao, CHEN Haowen, ZHAO Huanle, et al. Identification, development law and risk assessment of the hidden dangers of glacial lake outburst disasters on the Qinghai-Tibet Plateau[J]. Geological Bulletin of China,2023,42(5):730 − 742. (in Chinese with English abstract) [TANG Minggao, CHEN Haowen, ZHAO Huanle, et al. Identification, development law and risk assessment of the hidden dangers of glacial lake outburst disasters on the Qinghai-Tibet Plateau[J]. Geological Bulletin of China, 2023, 42(5): 730-742.(in Chinese with English abstract)
[23] 李彩虹, 李雪, 郭长宝, 等. 青藏高原东部鲜水河断裂带地震滑坡危险性评价[J]. 地质通报,2022,41(8):1473 − 1486. [LI Caihong, LI Xue, GUO Changbao, et al. Seismic landslide hazards assessment along the Xianshuihe fault zone, Tibetan Plateau, China[J]. Geological Bulletin of China,2022,41(8):1473 − 1486. (in Chinese with English abstract) LI Caihong, LI Xue, GUO Changbao, et al. Seismic landslide hazards assessment along the Xianshuihe fault zone, Tibetan Plateau, China[J]. Geological Bulletin of China, 2022, 41(8): 1473-1486.(in Chinese with English abstract)
[24] CHUNG C,FABBRI A. Probabilistic prediction models for landslide hazard mapping[J]. Photogrammetric Engineering and Remote Sensing,1999,65:1389 − 1400.
[25] 袁东, 张广泽, 王栋, 等. 西部山区交通廊道泥石流发育特征及选线对策[J]. 地质通报,2023,42(5):743 − 752. [YUAN Dong, ZHANG Guangze, WANG Dong, et al. Analysis on development characteristics of debris flow and route selection countermeasures along the traffic lines in mountain areas of Western China[J]. Geological Bulletin of China,2023,42(5):743 − 752. (in Chinese with English abstract) [YUAN Dong, ZHANG Guangze, WANG Dong, et al. Analysis on development characteristics of debris flow and route selection countermeasures along the traffic lines in mountain areas of Western China[J]. Geological Bulletin of China, 2023, 42(5): 743-752.(in Chinese with English abstract)
-
期刊类型引用(3)
1. 张华湘,曾牡丹,孙乾征. 典型区域地质灾害风险评价及管控对策研究. 贵州科学. 2025(01): 72-77 .  百度学术
百度学术
2. 何娜,朱习松,吴福,刘昶,吴秋菊,黄希明,蒋力,肖吉贵,文海涛,何添杰,常鸣. 基于全连接神经网络的广西北流市崩塌滑坡风险评价. 水土保持通报. 2025(01): 127-136 .  百度学术
百度学术
3. 冯博 ,程旭 ,范芳 ,卢辉雄 ,李瑞炜 ,魏本赞 . 基于信息量模型的云南禄丰地质灾害风险性评价. 世界核地质科学. 2024(06): 1260-1274 .  百度学术
百度学术
其他类型引用(0)





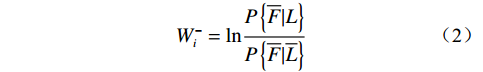
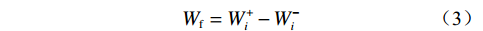
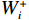
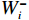
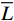
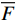
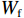
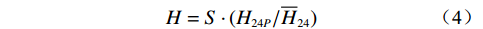
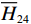
 下载:
下载:
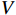


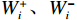
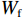
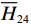
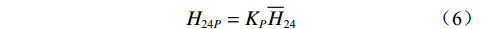
 邮件订阅
邮件订阅 RSS
RSS